\(\lim_{{x \to 0}} \limits\) \(\frac{cos(sin x) - cos x }{x^4}\) is equal to :
\(\lim_{{x \to 0}} \limits\) \(\frac{cos(sin x) - cos x }{x^4}\) is equal to :
\(\frac{1}{3}\)
\(\frac{1}{4}\)
\(\frac{1}{6}\)
\(\frac{1}{12}\)
The Correct Option is C
Solution and Explanation
The correct answer is (C) : \(\frac{-11}{9}\)
\(\lim_{{x \to 0}} \limits\) \(\frac{cos(sinx) - cosx}{x^4}\) = \(\lim_{{x \to 0}} \limits\) \(\frac{2sin(x + sinx).sin(\frac{x - sinx}{2})}{x^4}\)
= \(\lim_{{x \to 0}} \limits\) \(2.\frac{(\frac{( x + sinx }{2})(\frac{x-sinx}{2})}{x^4}\)
= \(\lim_{{x \to 0}} \limits\) \(\frac{1}{2}.\) \((\frac{(x+x -\frac{x^3}{3!}+\frac{x^5}{5!}....)(x-x+\frac{x^3}{3!}....}{x^4})\)
= \(\lim_{{x \to 0}} \limits\) \(\frac{1}{2}.\) \((2-\frac{x^2}{3!}+\frac{x^4}{5!}....)(\frac{1}{3!}-\frac{x^2}{5!}-1)\)
= \(\frac{1}{6}\)
Top Questions on limits of trigonometric functions
- The value of \( \lim_{x \to 0} \left( \frac{\tan 11x}{\tan 5x} \right) \) is:
- GATE MN - 2025
- Engineering Mathematics
- limits of trigonometric functions
- Find the value of the following expression: \[ \tan^2(\sec^{-1}4) + \cot(\csc^{-1}3) \]
- MHT CET - 2025
- Mathematics
- limits of trigonometric functions
- Given a real-valued function \( f \) such that: \[ f(x) = \begin{cases} \frac{\tan^2\{x\}}{x^2 - \lfloor x \rfloor^2}, & \text{for } x > 0 \\ 1, & \text{for } x = 0 \\ \sqrt{\{x\} \cot\{x\}}, & \text{for } x < 0 \end{cases} \] Then:
- BITSAT - 2024
- Mathematics
- limits of trigonometric functions
- Evaluate the limit: \[ \lim_{\theta \to \frac{\pi}{2}} \frac{8\tan^4\theta + 4\tan^2\theta + 5}{(3 - 2\tan\theta)^4} \]
- TS EAMCET - 2024
- Mathematics
- limits of trigonometric functions
- If \( f(x) \) is defined as follows:
\[ f(x) = \begin{cases} \frac{x - \lfloor x \rfloor}{x - 2} & \text{if } x = 2 \\ \frac{|x - \lfloor x \rfloor|}{a^2 + (x - \lfloor x \rfloor)^2} & \text{if } 1 < x < 2 \\ 2a - b & \text{if } x = 1 \end{cases} \]
Then the limit \( \displaystyle \lim_{x \to 0} \frac{\sin(ax) + x \tan(bx)}{x^2} \) is:- AP EAPCET - 2023
- Mathematics
- limits of trigonometric functions
Questions Asked in JEE Main exam
- A force of 49 N acts tangentially at the highest point of a sphere (solid of mass 20 kg) kept on a rough horizontal plane. If the sphere rolls without slipping, then the acceleration of the center of the sphere is:
- JEE Main - 2025
- Quantum Mechanics
Let \( y^2 = 12x \) be the parabola and \( S \) its focus. Let \( PQ \) be a focal chord of the parabola such that \( (SP)(SQ) = \frac{147}{4} \). Let \( C \) be the circle described by taking \( PQ \) as a diameter. If the equation of the circle \( C \) is: \[ 64x^2 + 64y^2 - \alpha x - 64\sqrt{3}y = \beta, \] then \( \beta - \alpha \) is equal to:
- JEE Main - 2025
- Parabola
The expression given below shows the variation of velocity \( v \) with time \( t \): \[ v = \frac{At^2 + Bt}{C + t} \] The dimension of \( A \), \( B \), and \( C \) is:
- JEE Main - 2025
- Dimensional Analysis
The dimensions of a physical quantity \( \epsilon_0 \frac{d\Phi_E}{dt} \) are similar to [Symbols have their usual meanings]
- JEE Main - 2025
- Dimensional analysis
- Match List-I with List-II.
- JEE Main - 2025
- Dimensional analysis
Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
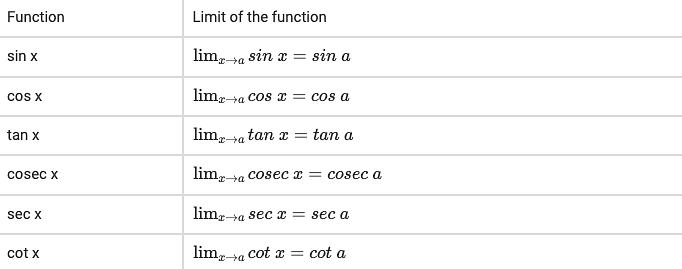
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:
