The pressure of a gas changes linearly with volume from $A$ to $B$ as shown in figure If no heat is supplied to or extracted from the gas then change in the internal energy of the gas will be Is
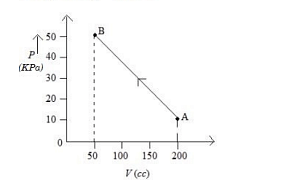
The pressure of a gas changes linearly with volume from $A$ to $B$ as shown in figure If no heat is supplied to or extracted from the gas then change in the internal energy of the gas will be Is
Show Hint
- $4.5 J$
- $-4.5 J$
- zero
- $6 J$
The Correct Option is A
Approach Solution - 1
Approach Solution -2
\[ \Delta u = - W \]
The work done by the gas is given by:\[ W = \int P \, dV \]
Since the pressure changes linearly, we can use the average pressure to calculate the work done:\[ W = P_{\text{avg}} \times \Delta V = 30 \times 10^3 \times 150 \times 10^{-6} = 4500 \times 10^{-3} = 4.5 \, \text{J} \]
Thus, the change in internal energy is \( 4.5 \, \text{J} \).Top Questions on specific heat capacity
Match List-I with List-II.
List-I List-II (A) Heat capacity of body (I) \( J\,kg^{-1} \) (B) Specific heat capacity of body (II) \( J\,K^{-1} \) (C) Latent heat (III) \( J\,kg^{-1}K^{-1} \) (D) Thermal conductivity (IV) \( J\,m^{-1}K^{-1}s^{-1} \) - JEE Main - 2025
- Physics
- specific heat capacity
- Product of uncertainty in position (\(\Delta x\)) and uncertainty in velocity (\(\Delta v\)) has unit:
- KEAM - 2025
- Physics
- specific heat capacity
- If \( \frac{C_p}{C_v} \) is unity for a process, \( PV^{\gamma} = \text{constant} \), then the process is:
- KEAM - 2025
- Physics
- specific heat capacity
- Specific heat capacity of diatomic gas at constant volume:
- KEAM - 2025
- Physics
- specific heat capacity
- Two moles a monoatomic gas is mixed with six moles of a diatomic gas. The molar specific heat of the mixture at constant volume is :
- JEE Main - 2024
- Physics
- specific heat capacity
Questions Asked in JEE Main exam
- Let $ \vec{a} $ and $ \vec{b} $ be the vectors of the same magnitude such that $ \frac{| \vec{a} + \vec{b} | + | \vec{a} - \vec{b} |}{| \vec{a} + \vec{b} | - | \vec{a} - \vec{b} |} = \sqrt{2} + 1. \quad \text{Then } \frac{| \vec{a} + \vec{b} |^2}{| \vec{a} |^2} \text{ is:} $
- JEE Main - 2025
- Vector Algebra
- Let the distance between two parallel lines be 5 units and a point \( P \) lies between the lines at a unit distance from one of them. An equilateral triangle \( POR \) is formed such that \( Q \) lies on one of the parallel lines, while \( R \) lies on the other. Then \( (QR)^2 \) is equal to _____________.
- JEE Main - 2025
- Coordinate Geometry
Let \( a \in \mathbb{R} \) and \( A \) be a matrix of order \( 3 \times 3 \) such that \( \det(A) = -4 \) and \[ A + I = \begin{bmatrix} 1 & a & 1 \\ 2 & 1 & 0 \\ a & 1 & 2 \end{bmatrix} \] where \( I \) is the identity matrix of order \( 3 \times 3 \).
If \( \det\left( (a + 1) \cdot \text{adj}\left( (a - 1) A \right) \right) \) is \( 2^m 3^n \), \( m, n \in \{ 0, 1, 2, \dots, 20 \} \), then \( m + n \) is equal to:
- JEE Main - 2025
- Determinants
- Preparation of potassium permanganate from MnO\(_2\) involves a two-step process in which the 1st step is a reaction with KOH and KNO\(_3\) to produce:
- JEE Main - 2025
- Preparation
Rate law for a reaction between $A$ and $B$ is given by $\mathrm{R}=\mathrm{k}[\mathrm{A}]^{\mathrm{n}}[\mathrm{B}]^{\mathrm{m}}$. If concentration of A is doubled and concentration of B is halved from their initial value, the ratio of new rate of reaction to the initial rate of reaction $\left(\frac{\mathrm{r}_{2}}{\mathrm{r}_{1}}\right)$ is
- JEE Main - 2025
- Chemical Kinetics
Concepts Used:
Thermodynamics
Thermodynamics in physics is a branch that deals with heat, work and temperature, and their relation to energy, radiation and physical properties of matter.
Important Terms
System
A thermodynamic system is a specific portion of matter with a definite boundary on which our attention is focused. The system boundary may be real or imaginary, fixed or deformable.
There are three types of systems:
- Isolated System – An isolated system cannot exchange both energy and mass with its surroundings. The universe is considered an isolated system.
- Closed System – Across the boundary of the closed system, the transfer of energy takes place but the transfer of mass doesn’t take place. Refrigerators and compression of gas in the piston-cylinder assembly are examples of closed systems.
- Open System – In an open system, the mass and energy both may be transferred between the system and surroundings. A steam turbine is an example of an open system.
Thermodynamic Process
A system undergoes a thermodynamic process when there is some energetic change within the system that is associated with changes in pressure, volume and internal energy.
There are four types of thermodynamic process that have their unique properties, and they are:
- Adiabatic Process – A process in which no heat transfer takes place.
- Isochoric Process – A thermodynamic process taking place at constant volume is known as the isochoric process.
- Isobaric Process – A process in which no change in pressure occurs.
- Isothermal Process – A process in which no change in temperature occurs.
Laws of Thermodynamics
Zeroth Law of Thermodynamics
The Zeroth law of thermodynamics states that if two bodies are individually in equilibrium with a separate third body, then the first two bodies are also in thermal equilibrium with each other.
First Law of Thermodynamics
The First law of thermodynamics is a version of the law of conservation of energy, adapted for thermodynamic processes, distinguishing three kinds of transfer of energy, as heat, as thermodynamic work, and as energy associated with matter transfer, and relating them to a function of a body's state, called internal energy.
Second Law of Thermodynamics
The Second law of thermodynamics is a physical law of thermodynamics about heat and loss in its conversion.
Third Law of Thermodynamics
Third law of thermodynamics states, regarding the properties of closed systems in thermodynamic equilibrium: The entropy of a system approaches a constant value when its temperature approaches absolute zero.