Question:
Let \(cos^{-1}\frac{y}{b}\)=\(log_e (\frac{x}{n})^n\), then Ay2+By1+Cy=0 is possible : (where y2=\(\frac{d^2y}{dx^2}\), y1=\(\frac{dy}{dx}\))
Let \(cos^{-1}\frac{y}{b}\)=\(log_e (\frac{x}{n})^n\), then Ay2+By1+Cy=0 is possible : (where y2=\(\frac{d^2y}{dx^2}\), y1=\(\frac{dy}{dx}\))
Updated On: Oct 21, 2024
- A=2, B=x2, C=n
- A=x2, B=x, C=n2
- A=x, B=2x, C=3n+1
- A=x2, B=3x, C=2n
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
The correct option is (B): A=x2, B=x, C=n2
Was this answer helpful?
0
9
Top Questions on limits of trigonometric functions
- Given a real-valued function \( f \) such that: \[ f(x) = \begin{cases} \frac{\tan^2\{x\}}{x^2 - \lfloor x \rfloor^2}, & \text{for } x > 0 \\ 1, & \text{for } x = 0 \\ \sqrt{\{x\} \cot\{x\}}, & \text{for } x < 0 \end{cases} \] Then:
- BITSAT - 2024
- Mathematics
- limits of trigonometric functions
- Evaluate the limit: \[ \lim_{\theta \to \frac{\pi}{2}} \frac{8\tan^4\theta + 4\tan^2\theta + 5}{(3 - 2\tan\theta)^4} \]
- TS EAMCET - 2024
- Mathematics
- limits of trigonometric functions
- If \(\lim\limits_{x \rightarrow 0} \frac{\sin(2+x)-\sin(2-x)}{x}\)= A cos B, then the values of A and B respectively are
- KCET - 2023
- Mathematics
- limits of trigonometric functions
- Find the value of \[ \tan^{-1}\left(\frac{1}{4}\right) + \tan^{-1}\left(\frac{2}{9}\right) \]
- BITSAT - 2023
- Mathematics
- limits of trigonometric functions
- \(\begin{array}{l}\displaystyle \lim_{ x\to 0}\left(\frac{(x+2\cos x)^3 + 2(x+2\cos x)^2 + 3 \sin(x+2\cos x)}{(x+2)^3+2(x+2)^2+3\sin(x+2)}\right)^{\frac{100}{x}} \end{array}\)is equal to _________.
- JEE Main - 2022
- Mathematics
- limits of trigonometric functions
View More Questions
Questions Asked in WBJEE exam
- Two straight conducting plates form an angle θ where their ends are joined. A conducting bar in contact with the plates and forming an isosceles triangle with them, starts at the vertex at time t = 0 and moves with constant velocity v to the right as shown in the figure. A magnetic field B points out of the page. The magnitude of emf induced at t = 1 second will be:
- WBJEE - 2024
- Electromagnetic induction
- A 2V cell is connected across the points A and B as shown in the figure. Assume that the resistance of each diode is zero in forward bias and infinity in reverse bias. The current supplied by the cell is:
- WBJEE - 2024
- Current electricity
- Consider a circuit where a cell of emf \( E_0 \) and internal resistance \( r \) is connected across the terminal A and B as shown in the figure. The value of \( R \) for which the power generated in the circuit is maximum, is given by:
- WBJEE - 2024
- Current electricity
- In a series LCR circuit, the rms voltage across the resistor and the capacitor are \(30 \, \text{V}\) and \(90 \, \text{V}\) respectively. If the applied voltage is \(50\sqrt{2}\sin\omega t\), then the peak voltage across the inductor is:
- WBJEE - 2024
- Alternating current
- Which of the following statement(s) is/are true in respect of nuclear binding energy?
(i) The mass energy of a nucleus is larger than the total mass energy of its individual protons and neutrons.
(ii) If a nucleus could be separated into its nucleons, an energy equal to the binding energy would have to be transferred to the particles during the separating process.
(iii) The binding energy is a measure of how well the nucleons in a nucleus are held together.
(iv) The nuclear fission is somehow related to acquiring higher binding energy.- WBJEE - 2024
- Nuclear physics
View More Questions
Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
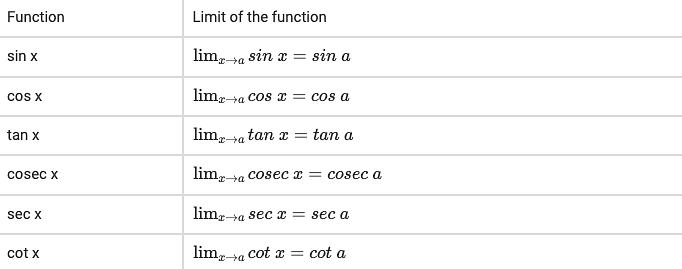
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:
