Question:
If \(\begin{array}{l}\int_{0}^{\sqrt{3}}\frac{15x^3}{\sqrt{1+x^2 + \sqrt{(1+x^2)^3}}}dx = \alpha \sqrt{2}+\beta\sqrt{3},\end{array}\)where \(α, β\) are integers, then \(α + β\) is equal to
If \(\begin{array}{l}\int_{0}^{\sqrt{3}}\frac{15x^3}{\sqrt{1+x^2 + \sqrt{(1+x^2)^3}}}dx = \alpha \sqrt{2}+\beta\sqrt{3},\end{array}\)where \(α, β\) are integers, then \(α + β\) is equal to
Updated On: Oct 2, 2025
Hide Solution
Verified By Collegedunia
Solution and Explanation
Put
\(\begin{array}{l}x = tan\theta \Rightarrow dx = sec^2\theta~ d\theta\end{array}\)
\(\begin{array}{l}\Rightarrow I = \int_{0}^{\frac{\pi}{3}}\frac{15\tan^3\theta . \sec^2\theta\ d\theta}{\sqrt{1+\tan^2\theta + \sqrt{\sec^6\theta}}}\end{array}\)
\(\begin{array}{l}\Rightarrow I = \int_{0}^{\frac{\pi}{3}}\frac{15\tan^2\theta \sec^2 \theta \ d\theta}{\sec \theta \sqrt{1+ \sec \theta}}\end{array}\)
\(\begin{array}{l}\Rightarrow I = \int_{0}^{\frac{\pi}{3}}\frac{15(\sec^2 \theta -1)\sec \theta \tan \theta \ d\theta}{\left(\sqrt{1+\sec \theta}\right)}\end{array}\)
Now put 1 + secθ = t2
\(\begin{array}{l}\Rightarrow sec~\theta ~tan~\theta~ d\theta = 2tdt\end{array}\)
\(\begin{array}{l}\Rightarrow I = \int_{\sqrt{2}}^{\sqrt{3}}\frac{15((t^2-1)^2-1)2t \ dt}{t}\end{array}\)
\(\begin{array}{l}\Rightarrow I = 30\int_{\sqrt{2}}^{\sqrt{3}}\left(t^4-2t^2 + 1-1\right)dt\end{array}\)
\(\begin{array}{l}\Rightarrow I = 30\int_{\sqrt{2}}^{\sqrt{3}}\left(t^4-2t^2 + 1-1\right)dt\end{array}\)
\(\begin{array}{l}\Rightarrow I = 30 \left(\frac{t^5}{5}-\frac{2t^3}{3}\right)_{\sqrt{2}}^{\sqrt{3}}\end{array}\)
\(\begin{array}{l}=30\left[\left(\frac{9}{5}\sqrt{3}-2\sqrt{3}\right)-\left(\frac{4\sqrt{2}}{5}-\frac{4\sqrt{2}}{3}\right)\right]\end{array}\)
\(\begin{array}{l}=(54\sqrt{3}-60\sqrt{3})-(24\sqrt{2}-40\sqrt{2})\end{array}\)
\(\begin{array}{l}=16\sqrt{2}-6\sqrt{3}\end{array}\)
\(\begin{array}{l} \therefore \alpha = 16 ~\text{and}~ \beta = – 6\\ \alpha + \beta = 10.\end{array}\)
Was this answer helpful?
1
1
Top Questions on Integration by Partial Fractions
- If $\frac{x+3}{(x+1)(x^2+2)} = \frac{a}{x+1} + \frac{bx+c}{x^2+2}$ then $a-b+c =$
- TS EAMCET - 2025
- Mathematics
- Integration by Partial Fractions
- Let {an}n=0∞ be a sequence such that a0=a1=0 and an+2=3an+1−2an+1,∀ n≥0. Then a25a23−2a25a22−2a23a24+4a22a24 is equal to
- JEE Main - 2025
- Mathematics
- Integration by Partial Fractions
- Find the value of \( \frac{5}{6} + \frac{3}{4} \).
- MHT CET - 2025
- Mathematics
- Integration by Partial Fractions
- Let for \( f(x) = 7\tan^8 x + 7\tan^6 x - 3\tan^4 x - 3\tan^2 x \), \( I_1 = \int_0^{\frac{\pi}{4}} f(x)dx \) and \( I_2 = \int_0^{\frac{\pi}{4}} x f(x)dx \). Then \( 7I_1 + 12I_2 \) is equal to:
- JEE Main - 2025
- Mathematics
- Integration by Partial Fractions
- If ∫ (2x + 3)/((x - 1)(x^2 + 1)) dx = log_x {(x - 1)^(5/2)(x^2 + 1)^a} - (1/2) tan^(-1)x + C, then the value of a is:
- MHT CET - 2025
- Mathematics
- Integration by Partial Fractions
View More Questions
Questions Asked in JEE Main exam
- A 400 g solid cube having an edge of length \(10\) cm floats in water. How much volume of the cube is outside the water? (Given: density of water = \(1000 { kg/m}^3\))
Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A): The density of the copper ($^{64}Cu$) nucleus is greater than that of the carbon ($^{12}C$) nucleus.
Reason (R): The nucleus of mass number A has a radius proportional to $A^{1/3}$.
In the light of the above statements, choose the most appropriate answer from the options given below:- JEE Main - 2025
- Nuclear physics
- The roots of the quadratic equation \( 3x^2 - px + q = 0 \) are the 10th and 11th terms of an arithmetic progression with common difference \( \frac{3}{2} \). If the sum of the first 11 terms of this arithmetic progression is 88, then \( q - 2q \) is equal to:
- JEE Main - 2025
- Arithmetic Progression and Quadratic Equations
- The type of oxide formed by the element among Li, Na, Be, Mg, B and Al that has the least atomic radius is:
- JEE Main - 2025
- Periodicity of Elements
- The number of real solution(s) of the equation \( x^2 + 3x + 2 = \min \left( |x - 3|, |x + 2| \right) \) is:
- JEE Main - 2025
- Coordinate Geometry
View More Questions
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
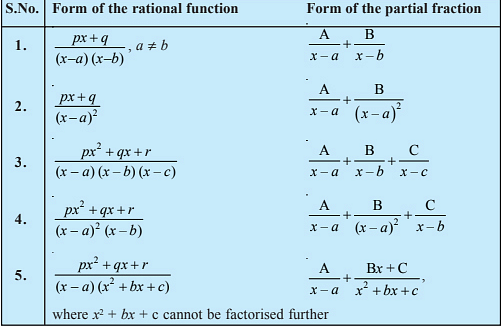
For examples,
