For l ∈ R, the equation (2l - 3) x2 + 2lxy - y2 = 0 represents a pair of distinct lines
For l ∈ R, the equation (2l - 3) x2 + 2lxy - y2 = 0 represents a pair of distinct lines
only when l = 0
for all values of l ∈ (-3, 1)
for all values of l ∈ R - (0, 1)
for all values of l ∈ R - (-3, 1)
The Correct Option is D
Solution and Explanation
To determine the values of $l$ for which the equation $(2l-3)x^2 + 2lxy - y^2 = 0$ represents a pair of distinct lines, we proceed:
1. Recognizing the Equation Type:
The given equation $(2l-3)x^2 + 2lxy - y^2 = 0$ is a homogeneous equation of degree 2, suggesting it may represent a pair of lines passing through the origin.
2. Condition for Distinct Lines:
For a homogeneous quadratic equation $ax^2 + 2hxy + by^2 = 0$ to represent a pair of distinct lines, the discriminant condition must be satisfied: $h^2 - ab > 0$. Identify the coefficients by comparing with the standard form:
$ a = 2l - 3, \quad h = l, \quad b = -1 $
3. Computing the Discriminant:
Calculate the discriminant:
$ h^2 - ab = l^2 - (2l - 3)(-1) = l^2 + (2l - 3) = l^2 + 2l - 3 $
For distinct lines, we need:
$ l^2 + 2l - 3 > 0 $
4. Solving the Inequality:
Solve the quadratic inequality $l^2 + 2l - 3 > 0$. First, find the roots of the equation $l^2 + 2l - 3 = 0$:
$ (l + 3)(l - 1) = 0 $
Roots are $l = -3$ and $l = 1$. Since the parabola $l^2 + 2l - 3$ opens upward, test the sign in the intervals $(-\infty, -3)$, $(-3, 1)$, and $(1, \infty)$:
- For $l = -4$: $(-4 + 3)(-4 - 1) = (-1)(-5) = 5 > 0$
- For $l = 0$: $(0 + 3)(0 - 1) = 3 \cdot (-1) = -3 < 0$
- For $l = 2$: $(2 + 3)(2 - 1) = 5 \cdot 1 = 5 > 0$
Thus, $(l + 3)(l - 1) > 0$ when $l < -3$ or $l > 1$.
5. Expressing the Solution:
The solution to the inequality is:
$ l \in (-\infty, -3) \cup (1, \infty) $
This can be written as:
$ l \in \mathbb{R} - [-3, 1] $
Final Answer:
The equation represents a pair of distinct lines for all $l \in \mathbb{R} - [-3, 1]$.
Top Questions on Three Dimensional Geometry
Show that the following lines intersect. Also, find their point of intersection:
Line 1: \[ \frac{x - 1}{2} = \frac{y - 2}{3} = \frac{z - 3}{4} \]
Line 2: \[ \frac{x - 4}{5} = \frac{y - 1}{2} = z \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
- Find the value of λ, if the points A(−1,−1,2), B(2,8,λ), C(3,11,6) are collinear.
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
The vector equations of two lines are given as:
Line 1: \[ \vec{r}_1 = \hat{i} + 2\hat{j} - 4\hat{k} + \lambda(4\hat{i} + 6\hat{j} + 12\hat{k}) \]
Line 2: \[ \vec{r}_2 = 3\hat{i} + 3\hat{j} - 5\hat{k} + \mu(6\hat{i} + 9\hat{j} + 18\hat{k}) \]
Determine whether the lines are parallel, intersecting, skew, or coincident. If they are not coincident, find the shortest distance between them.
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
Determine the vector equation of the line that passes through the point \( (1, 2, -3) \) and is perpendicular to both of the following lines:
\[ \frac{x - 8}{3} = \frac{y + 16}{7} = \frac{z - 10}{-16} \quad \text{and} \quad \frac{x - 15}{3} = \frac{y - 29}{-8} = \frac{z - 5}{-5} \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
- Find the length of the diagonal of a rectangle with length 6 cm and breadth 8 cm.
- MHT CET - 2025
- Mathematics
- Three Dimensional Geometry
Questions Asked in TS EAMCET exam
- In a photoelectric experiment the incident photons have frequency \( \frac{3}{2} \nu \), where \( \nu \) is the threshold frequency of the material. What is the kinetic energy of the emitted electrons?
- TS EAMCET - 2025
- Kinematics
- Find the value of \( k \) such that \( 5 \cdot 2^n - 48n + k \) is divisible by 24.
- TS EAMCET - 2025
- Quadratic Equations
- The molecular weight of a gas is 32. If 0.5 moles of the gas occupy 22.4 liters at standard temperature and pressure (STP), what is the density of the gas?
- TS EAMCET - 2025
- Gas laws
- A ball is projected vertically up with speed \( V_0 \) from a certain height \( H \). When the ball reaches the ground, the speed is \( 3V_0 \). The time taken by the ball to reach the ground and height \( H \) respectively are:
- TS EAMCET - 2025
- Projectile motion
- A body is projected vertically upward with an initial velocity of 40 m/s. Calculate the maximum height reached by the body. (Take \( g = 9.8 \, \text{m/s}^2 \))
- TS EAMCET - 2025
- Kinematics
Concepts Used:
Three Dimensional Geometry
Mathematically, Geometry is one of the most important topics. The concepts of Geometry are derived w.r.t. the planes. So, Geometry is divided into three major categories based on its dimensions which are one-dimensional geometry, two-dimensional geometry, and three-dimensional geometry.
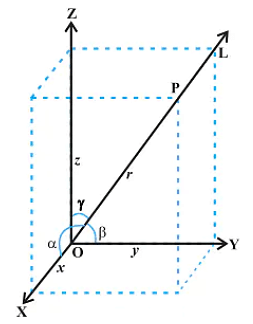
Direction Cosines and Direction Ratios of Line:
Consider a line L that is passing through the three-dimensional plane. Now, x,y and z are the axes of the plane and α,β, and γ are the three angles the line makes with these axes. These are commonly known as the direction angles of the plane. So, appropriately, we can say that cosα, cosβ, and cosγ are the direction cosines of the given line L.