State whether the following are true or false. Justify your answer.
(i) The value of tan A is always less than 1.
(ii) sec A = \(\frac{12}{5}\) for some value of angle A.
(iii) cos A is the abbreviation used for the cosecant of angle A.
(iv) cot A is the product of cot and A.
(v) sin \(\theta\) = \(\frac{4}{3}\) for some angle \(\theta\).
(i) The value of tan A is always less than 1.
(ii) sec A = \(\frac{12}{5}\) for some value of angle A.
(iii) cos A is the abbreviation used for the cosecant of angle A.
(iv) cot A is the product of cot and A.
(v) sin \(\theta\) = \(\frac{4}{3}\) for some angle \(\theta\).
Solution and Explanation
(i) Consider a \(ΔABC\), right-angled at B.
tan A=\(\frac{12}{5}\)
But\(\frac{12}{5} > 1 \)
\(∴\) tan A\( > 1\)
So, tan A \(< 1\) is not always true.
Hence, the given statement is false.
(ii) sec A = \(\frac{12}{5}\)

\(\frac{AC}{AB}=\frac{12}{5}\)
Let AC be 12k, AB will be 5k, where k is a positive integer.
Applying Pythagoras theorem in \(ΔABC,\) we obtain
\(AC^ 2 = AB ^2 + BC^ 2 \)
\((12k) ^2 = (5k)^ 2 + BC^ 2 \)
\(144k ^2 = 25k ^2 + BC ^2 \)
\(BC^ 2 = 119k^ 2\)
\(BC = 10.9k \)
It can be observed that for given two sides AC = 12k and AB = 5k,
BC should be such that,
\(AC - AB < BC < AC + AB\)
\(12k - 5k < BC < 12k + 5k \)
\(7k < BC < 17 k\)
However, BC = 10.9k. Clearly, such a triangle is possible and hence, such value of sec A is possible.
Hence, the given statement is true.
(iii) Abbreviation used for cosecant of angle A is cosec A. And cos A is the abbreviation used for cosine of angle A.
Hence, the given statement is false.
(iv) cot A is not the product of cot and A. It is the cotangent of ∠A.
Hence, the given statement is false.
(v) sin \(θ =\frac{4}{3}\)
We know that in a right-angled triangle,
\(\text{sin θ} = \frac{\text{Opposite}}{\text{Hypotenuse}}\)
In a right-angled triangle, hypotenuse is always greater than the remaining two sides. Therefore, such value of \(\text{sin θ}\) is not possible.
Hence, the given statement is false.
Top Questions on Trigonometric Ratios
If $\tan \theta = \tfrac{3}{4}$, then the value of $\cos \theta$ will be:
- UP Board X - 2025
- Mathematics
- Trigonometric Ratios
- Evaluate \( \dfrac{2 \tan 30^\circ}{1 + \tan^2 30^\circ} \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- If \( 15 \cot A = 8 \), then \( \sin A = \, ? \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- Evaluate \( (\sec A + \tan A)(1 - \sin A) = \ ? \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- Which of the following is true?
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
Questions Asked in CBSE X exam
- How did the Civil Disobedience Movement become a mass movement? Explain with examples.
- CBSE Class X - 2025
- Nationalism in India
- Explain the functioning of conservative regimes established in France in 1815.
- CBSE Class X - 2025
- The Rise of Nationalism in Europe
- "It would be beneficial to develop sustainable ways to meet the growing energy demand in India." Support the statement.
- CBSE Class X - 2025
- Minerals and Energy Resources
"ई काशी छोड़कर कहीं न जाएँ" बिस्मिल्ला खाँ के मन में काशी के प्रति विशेष अनुराग के क्या कारण थे ?
- CBSE Class X - 2025
- प्रश्न उत्तर
'संस्कृति' पाठ के आधार पर संस्कृति और असंस्कृति में अंतर बताइए।
- CBSE Class X - 2025
- प्रश्न उत्तर
Concepts Used:
Trigonometric Functions
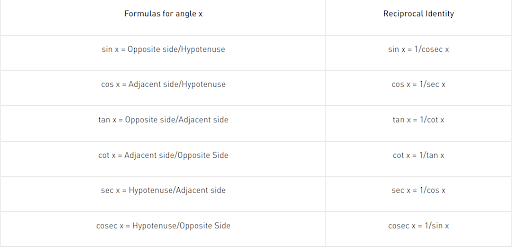
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions: