Let $f: R \rightarrow R$ be defined as,
$f(x) =
\begin{cases}
-55x, & \text{if } x < -5 \\
2x^3 -3x^2 -120x, & \text{if } 5 \le x \le 4 \\
2x^3 -3x^2-36x-336& \text{if } x > 4,
\end{cases} $
Let $A=\{ x \in R : f$ is increasing $\} .$ Then $A$ is equal to
- $(-\infty,-5) \cup(4, \infty)$
- $(-5, \infty)$
- $(-\infty,-5) \cup(-4, \infty)$
- (-5,-4)$\cup(4, \infty)$
The Correct Option is D
Approach Solution - 1
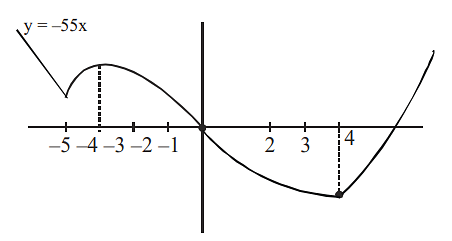
Approach Solution -2
The correct answer is (D) : (-5,-4)\(\cup(4, \infty)\)
For f'(x) ≥ 0
Case I :- -5 < x < 4
Then f'(x) ≥ 0 ≥ 0 implies
6x2 - 6x - 120 ≥ 0
⇒ x2 - x - 20 ≥ 0
⇒ (x - 5) (x + 4) ≥ 0
⇒ x ≤ - 4 or x ≥ 5
But -5 <x <4
∴ -5 < x < 4
Hence, function f(x) increases in domain x ∈ (-5, -4)
Case II :- x > 4
Then f'(x) ≥ ≥ 0 implies
6x2 - 6x - 36 ≥ 0
⇒ x2 - x - 6 ≥ 0
⇒ (x - 3) (x + 2) ≥ 0
⇒ x ≤ - 2 or x ≥ 3
But x > 4
Hence, f(x) increases in (4, ∞ )
From both cases, we can say that f(x) is increases in (-5, -4) U (4, ∞ ).
A = {x|x ∈ (-5, -4) U (4, ∞ )}
Top Questions on Functions
- Let \( f: \mathbb{R} \to \mathbb{R} \) be a function defined by \( f(x) = \left( 2 + 3a \right)x^2 + \left( \frac{a+2}{a-1} \right)x + b, a \neq 1 \). If \[ f(x + y) = f(x) + f(y) + 1 - \frac{2}{7}xy, \] then the value of \( 28 \sum_{i=1}^5 f(i) \) is:
- Let the function $ f(x) = \sqrt{\log_e(1 - x^2)} $. Then the domain of $ f(x) $ is:
- The number of points of discontinuity of the function $ f(x) = \left\lfloor \frac{x^2}{2} \right\rfloor - \left\lfloor \sqrt{x} \right\rfloor, \quad x \in [0, 4], $ where $ \left\lfloor \cdot \right\rfloor $ denotes the greatest integer function, is:
- If the domain of the function $ f(x) = \log_e \left( \frac{2x-3}{5+4x} \right) + \sin^{-1} \left( \frac{4+3x}{2-x} \right) $ is $ [\alpha, \beta] $, then $ \alpha^2 + 4\beta $ is equal to
- Let the domain of the function $ f(x) = \log_2 \log_4 \log_6 (3 + 4x - x^2) $ be (a, b). If $ \int_0^{a+b} [x^2] dx = p - q\sqrt{r} $, $ p, q, r \in \mathbb{N} $, gcd(p, q, r) = 1, where [.] is the greatest integer function, then p + q + r is equal to
Questions Asked in JEE Main exam
- A 400 g solid cube having an edge of length \(10\) cm floats in water. How much volume of the cube is outside the water? (Given: density of water = \(1000 { kg/m}^3\))
Let $ P_n = \alpha^n + \beta^n $, $ n \in \mathbb{N} $. If $ P_{10} = 123,\ P_9 = 76,\ P_8 = 47 $ and $ P_1 = 1 $, then the quadratic equation having roots $ \alpha $ and $ \frac{1}{\beta} $ is:
- JEE Main - 2025
- Relations and functions
- Density of 3 M NaCl solution is 1.25 g/mL. The molality of the solution is:
- JEE Main - 2025
- Solutions

In the given circuit the sliding contact is pulled outwards such that the electric current in the circuit changes at the rate of 8 A/s. At an instant when R is 12 Ω, the value of the current in the circuit will be A.- JEE Main - 2025
- Electrical Circuits
- Let \( \vec{a} = \hat{i} + 2\hat{j} + \hat{k} \) and \( \vec{b} = 2\hat{i} + 7\hat{j} + 3\hat{k} \). Let \( L_1 : \vec{r} = (-\hat{i} + 2\hat{j} + \hat{k}) + \lambda \vec{a}, \lambda \in {R} \) and \( L_2 : \vec{r} = (\hat{j} + \hat{k}) + \mu \vec{b}, \mu \in \mathbb{R} \) be two lines. If the line \( L_3 \) passes through the point of intersection of \( L_1 \) and \( L_2 \), and is parallel to \( \vec{a} + \vec{b} \), then \( L_3 \) passes through the point:
- JEE Main - 2025
- Vectors
Concepts Used:
Functions
A function is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. Let A & B be any two non-empty sets, mapping from A to B will be a function only when every element in set A has one end only one image in set B.
Kinds of Functions
The different types of functions are -
One to One Function: When elements of set A have a separate component of set B, we can determine that it is a one-to-one function. Besides, you can also call it injective.
Many to One Function: As the name suggests, here more than two elements in set A are mapped with one element in set B.
Moreover, if it happens that all the elements in set B have pre-images in set A, it is called an onto function or surjective function.
Also, if a function is both one-to-one and onto function, it is known as a bijective. This means, that all the elements of A are mapped with separate elements in B, and A holds a pre-image of elements of B.
Read More: Relations and Functions