In given circuit, reading of voltmeter is 1 V, then resistance of
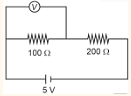

- 100 Ω
- 200 Ω
- 200√5 Ω
- 50 Ω
The Correct Option is A
Solution and Explanation
Top Questions on Heat Transfer
- From a metallic wall at 100$^\circ$C, a metallic rod protrudes to the ambient air. The temperature at the tip will be minimum when the rod is made of:
- AP PGECET - 2025
- Mechanical Engineering
- Heat Transfer
- As the distance from the leading edge increases, the thermal boundary layer thickness:
- AP PGECET - 2025
- Mechanical Engineering
- Heat Transfer
- Provision of fins on a given heat transfer surface will be more, if there are:
- AP PGECET - 2025
- Mechanical Engineering
- Heat Transfer
- Highest thermal conductivity is of
- AP PGECET - 2025
- Mechanical Engineering
- Heat Transfer
- Nusselt number is the ratio of:
- AP PGECET - 2025
- Mechanical Engineering
- Heat Transfer
Questions Asked in JEE Main exam
- Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : If Young’s double slit experiment is performed in an optically denser medium than air, then the consecutive fringes come closer.
Reason (R) : The speed of light reduces in an optically denser medium than air while its frequency does not change.
In the light of the above statements, choose the most appropriate answer from the options given below : - The increase in pressure required to decrease the volume of a water sample by 0.2percentage is \( P \times 10^5 \, \text{Nm}^{-2} \). Bulk modulus of water is \( 2.15 \times 10^9 \, \text{Nm}^{-2} \). The value of \( P \) is ________________.
- JEE Main - 2025
- System of Particles & Rotational Motion
The motion of an airplane is represented by the velocity-time graph as shown below. The distance covered by the airplane in the first 30.5 seconds is km.

- JEE Main - 2025
- Kinematics
- A force \( \mathbf{F} = 2\hat{i} + b\hat{j} + \hat{k} \) is applied on a particle and it undergoes a displacement \( \mathbf{r} = \hat{i} - 2\hat{j} - \hat{k} \). What will be the value of \( b \), if the work done on the particle is zero?
- JEE Main - 2025
- Work and Energy
Which of the following statement is true with respect to H\(_2\)O, NH\(_3\) and CH\(_4\)?
(A) The central atoms of all the molecules are sp\(^3\) hybridized.
(B) The H–O–H, H–N–H and H–C–H angles in the above molecules are 104.5°, 107.5° and 109.5° respectively.
(C) The increasing order of dipole moment is CH\(_4\)<NH\(_3\)<H\(_2\)O.
(D) Both H\(_2\)O and NH\(_3\) are Lewis acids and CH\(_4\) is a Lewis base.
(E) A solution of NH\(_3\) in H\(_2\)O is basic. In this solution NH\(_3\) and H\(_2\)O act as Lowry-Bronsted acid and base respectively.
- JEE Main - 2025
- Molecular Orbital Theory
Concepts Used:
Specific Heat Capacity
Specific heat of a solid or liquid is the amount of heat that raises the temperature of a unit mass of the solid through 1°C.
Molar Specific Heat:
The Molar specific heat of a solid or liquid of a material is the heat that you provide to raise the temperature of one mole of solid or liquid through 1K or 1°C.
Specific Heat at Constant Pressure or Volume:
The volume of solid remains constant when heated through a small range of temperature. This is known as specific heat at a constant volume. It is denoted as CV.
The pressure of solid remains constant when heated through a small range of temperature. This is known as specific heat at constant pressure which can be denoted as CP.