If $\lambda_1<\lambda_2$ are two values of $\lambda$ such that the angle between the planes $P_1: \vec{r}(3 \hat{i}-5 \hat{j}+\hat{k})=7$ and $P_2: \vec{r} \cdot(\lambda \hat{i}+\hat{j}-3 \hat{k})=9$ is $\sin ^{-1}\left(\frac{2 \sqrt{6}}{5}\right)$, then the square of the length of perpendicular from the point $\left(38 \lambda_1, 10 \lambda_2, 2\right)$ to the plane $P_1$ is _____
Correct Answer: 315
Solution and Explanation
The correct answer is 315.
\(P_{1}=\vec{r}.(3\hat{i}-5\hat{j}+\hat{k})=7\)
\(P_{2}=\vec{r}.(\lambda \hat{i}+\hat{j}-3\hat{k})=9\)
\(\theta =sin^{-1}(\frac{2\sqrt{6}}{5})\)
\(\Rightarrow sin\theta =\frac{2\sqrt{6}}{5}\)
\(\therefore cos\theta =\frac{1}{5}.\)
\(cos\theta =\frac{\vec{r}.\vec{r}}{|\vec{r}||\vec{r_{2}}|}\)
\(=\frac{(3i-5j+k)(\lambda {i}+{j}-3{k})}{\sqrt{35}.\sqrt{\lambda ^{2}+10}}\)
\(\Rightarrow 19\lambda ^{2}-95\lambda -25\lambda +125\)=0
\(\Rightarrow x=5,\frac{25}{19}\)

Perpendicular distance of point
\((38\lambda _{1},10\lambda _{2},2)=(50,50,2)\) from plane P1
\(=\frac{|30\times50-5\times50+2-7}{\sqrt{35}}\)
\(Square=\frac{105\times 105}{35}=315\)
Top Questions on Three Dimensional Geometry
- If A(2,1,-1), B(6,-3,2), C(-3,12,4) are the vertices of a triangle ABC and the equation of the plane containing the triangle ABC is $53x+by+cz+d=0$, then $\frac{d}{b+c}=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- If $(\alpha, \beta, \gamma)$ is the foot of the perpendicular drawn from a point $(-1,2,-1)$ to the line joining the points $(2,-1,1)$ and $(1,1,-2)$, then $\alpha+\beta+\gamma=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- If m:n is the ratio in which the point $\left(\frac{8}{5}, \frac{1}{5}, \frac{8}{5}\right)$ divides the line segment joining the points (2,p,2) and (p,-2,p) where p is an integer then $\frac{3m+n}{3n}=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- Let $\pi_1$ be the plane determined by the vectors $\hat{i}+\hat{j}, \hat{i}+\hat{k}$ and $\pi_2$ be the plane determined by the vectors $\hat{j}-\hat{k}, \hat{k}-\hat{i}$. Let $\vec{a}$ be a non-zero vector parallel to the line of intersection of the planes $\pi_1$ and $\pi_2$. If $\vec{b} = \hat{i}+\hat{j}-\hat{k}$ then the angle between the vectors $\vec{a}$ and $\vec{b}$ is
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
Show that the following lines intersect. Also, find their point of intersection:
Line 1: \[ \frac{x - 1}{2} = \frac{y - 2}{3} = \frac{z - 3}{4} \]
Line 2: \[ \frac{x - 4}{5} = \frac{y - 1}{2} = z \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
Questions Asked in JEE Main exam
- The area of the region enclosed by the curves \( y = x^2 - 4x + 4 \) and \( y^2 = 16 - 8x \) is:
- JEE Main - 2025
- Area between Two Curves
- Let the foci of a hyperbola be \( (1, 14) \) and \( (1, -12) \). If it passes through the point \( (1, 6) \), then the length of its latus-rectum is:
- JEE Main - 2025
- Hyperbola
- Earth has mass 8 times and radius 2 times that of a planet. If the escape velocity from the earth is 11.2 km/s, the escape velocity in km/s from the planet will be:
- JEE Main - 2025
- Speed and velocity
- Let \(E_1: \frac{x^2}{9} + \frac{y^2}{4} = 1\) be an ellipse. Ellipses \(E_i\) are constructed such that their centers and eccentricities are the same as that of \(E_1\), and the length of the minor axis of \(E_{i+1}\) is the length of the major axis of \(E_i\). If \(A_i\) is the area of the ellipse \(E_i\), then \(\frac{5}{\pi} \sum_{i=1}^{\infty} A_i\) is equal to:
- JEE Main - 2025
- Calculus
- The compound with molecular formula C\(_6\)H\(_6\), which gives only one monobromo derivative and takes up four moles of hydrogen per mole for complete hydrogenation has \(\_\_\_\_\_\)\( \pi \) electrons.
- JEE Main - 2025
- Aromaticity & chemistry of aromatic compounds
Concepts Used:
Three Dimensional Geometry
Mathematically, Geometry is one of the most important topics. The concepts of Geometry are derived w.r.t. the planes. So, Geometry is divided into three major categories based on its dimensions which are one-dimensional geometry, two-dimensional geometry, and three-dimensional geometry.
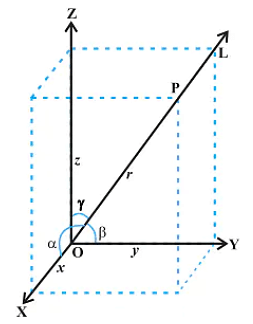
Direction Cosines and Direction Ratios of Line:
Consider a line L that is passing through the three-dimensional plane. Now, x,y and z are the axes of the plane and α,β, and γ are the three angles the line makes with these axes. These are commonly known as the direction angles of the plane. So, appropriately, we can say that cosα, cosβ, and cosγ are the direction cosines of the given line L.