For a complex number $z$, let $Re(z)$ denote the real part of $z$. Let $S$ be the set of all complex numbers $z$ satisfying $z^{4}-|z|^{4}=4 i z^{2}$, where $i=\sqrt{-1}$. Then the minimum possible value of $\left|z_{1}-z_{2}\right|^{2}$, where $z_{1}, z_{2} \in S$ with \(Re\left( z _{1}\right)\)\(>\)0 and \(Re\left( z _{2}\right)\) \(<\) 0, is ______
Correct Answer: 8
Solution and Explanation
\(|z_1 β z_2|^ 2 = 8\)
Top Questions on Complex numbers
- Let the curve \(z(1 + i) +\) \(\overline{z(1 - i) = 4}\), z \(\in\)\( \mathbb{C}\), divide the region \(|z - 3| \leq 1\) into two parts of areas \( \alpha \) and \( \beta \). Then \( |\alpha - \beta| \) equals:}
- JEE Main - 2025
- Mathematics
- Complex numbers
If \( z \) is a complex number and \( k \in \mathbb{R} \), such that \( |z| = 1 \), \[ \frac{2 + k^2 z}{k + \overline{z}} = kz, \] then the maximum distance from \( k + i k^2 \) to the circle \( |z - (1 + 2i)| = 1 \) is:
- JEE Main - 2025
- Mathematics
- Complex numbers
- Let \( z_1, z_2, z_3 \) be three complex numbers on the circle \( |z| = 1 \) with \( \arg(z_1) = -\frac{\pi}{4}, \arg(z_2) = 0 \) and \( \arg(z_3) = \frac{\pi}{4} \). If \( |z_1 \overline{z_2} + z_2 \overline{z_3} + z_3 \overline{z_1}|^2 = \alpha + \beta \sqrt{2} \), where \( \alpha, \beta \in \mathbb{Z} \), then the value of \( \alpha^2 + \beta^2 \) is :
- JEE Main - 2025
- Mathematics
- Complex numbers
- Let integers \( a, b \in [-3, 3] \) be such that \( a + b \neq 0 \). \(\text{Then the number of all possible ordered pairs}\) \( (a, b) \), \(\text{for which}\) \[ \left| \frac{z - a}{z + b} \right| = 1 \quad \text{and} \quad \left| \begin{matrix} z + 1 & \omega & \omega^2 \\ \omega^2 & 1 & z + \omega \\ \omega^2 & 1 & z + \omega \end{matrix} \right| = 1, \] \(\text{is equal to:}\)
- JEE Main - 2025
- Mathematics
- Complex numbers
- Among the statements: (S1): The set $ \{ z \in \mathbb{C} - \{-i\} : |z| = 1 \text{ and } \frac{z - i}{z + i} \text{ is purely real} \} $ contains exactly two elements.
(S2): The set $ \{ z \in \mathbb{C} - \{-1\} : |z| = 1 \text{ and } \frac{z - 1}{z + 1} \text{ is purely imaginary} \} $ contains infinitely many elements. Then, which of the following is correct?- JEE Main - 2025
- Mathematics
- Complex numbers
Questions Asked in JEE Advanced exam
The reaction sequence given below is carried out with 16 moles of X. The yield of the major product in each step is given below the product in parentheses. The amount (in grams) of S produced is ____.
Use: Atomic mass (in amu): H = 1, C = 12, O = 16, Br = 80- JEE Advanced - 2025
- Organic Chemistry
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
Let $ \mathbb{R} $ denote the set of all real numbers. Then the area of the region $$ \left\{ (x, y) \in \mathbb{R} \times \mathbb{R} : x > 0, y > \frac{1}{x},\ 5x - 4y - 1 > 0,\ 4x + 4y - 17 < 0 \right\} $$ is
- JEE Advanced - 2025
- Coordinate Geometry
The center of a disk of radius $ r $ and mass $ m $ is attached to a spring of spring constant $ k $, inside a ring of radius $ R>r $ as shown in the figure. The other end of the spring is attached on the periphery of the ring. Both the ring and the disk are in the same vertical plane. The disk can only roll along the inside periphery of the ring, without slipping. The spring can only be stretched or compressed along the periphery of the ring, following Hookeβs law. In equilibrium, the disk is at the bottom of the ring. Assuming small displacement of the disc, the time period of oscillation of center of mass of the disk is written as $ T = \frac{2\pi}{\omega} $. The correct expression for $ \omega $ is ( $ g $ is the acceleration due to gravity):
- JEE Advanced - 2025
- Waves and Oscillations
Concepts Used:
Law of Chemical Equilibrium
Law of Chemical Equilibrium states that at a constant temperature, the rate of a chemical reaction is directly proportional to the product of the molar concentrations of the reactants each raised to a power equal to the corresponding stoichiometric coefficients as represented by the balanced chemical equation.
Let us consider a general reversible reaction;
A+B β C+D
After some time, there is a reduction in reactants A and B and an accumulation of the products C and D. As a result, the rate of the forward reaction decreases and that of backward reaction increases.
Eventually, the two reactions occur at the same rate and a state of equilibrium is attained.
By applying the Law of Mass Action;
The rate of forward reaction;
Rf = Kf [A]a [B]b
The rate of backward reaction;
Rb = Kb [C]c [D]d
Where,
[A], [B], [C] and [D] are the concentrations of A, B, C and D at equilibrium respectively.
a, b, c, and d are the stoichiometric coefficients of A, B, C and D respectively.
Kf and KbΒ are the rate constants of forward and backward reactions.
However, at equilibrium,
Rate of forward reaction = Rate of backward reaction.
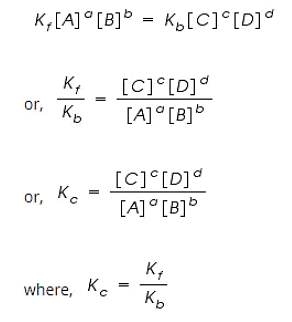
Kc is called the equilibrium constant expressed in terms of molar concentrations.
The above equation is known as the equation of Law of Chemical Equilibrium.