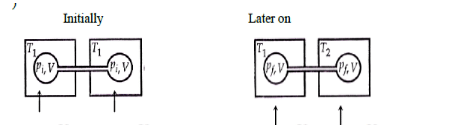
Two closed bulbs of equal volume $(V)$ containing an ideal gas initially at pressure $p_i$ and temperature $T_1$ are connected through a narrow tube of negligible volume as shown in the figure below. The temperature of one of the bulbs is then raised to $T_2$. The final pressure $p_f$ is :
- $p_{i} \left( \frac{T_{1}T_{2}}{T_{1} +T_{2}}\right) $
- $2 p_{i} \left( \frac{T_{1}}{T_{1} +T_{2}}\right) $
- $2 p_{i} \left( \frac{ T_{2}}{T_{1} +T_{2}}\right) $
- $2 p_{i} \left( \frac{T_{1}T_{2}}{T_{1} +T_{2}}\right) $
The Correct Option is C
Solution and Explanation
Top Questions on Ideal gas equation
- In a container of volume 16.62 m$^3$ at 0°C temperature, 2 moles of oxygen, 5 moles of nitrogen and 3 moles of hydrogen are present, then the pressure in the container is (Universal gas constant = 8.31 J/mol K)
- AP EAPCET - 2025
- Physics
- Ideal gas equation
- Avogadro’s hypothesis relates volume of gases and:
- OJEE - 2024
- Chemistry
- Ideal gas equation
- An electric bulb of volume 300 cm$^3$ was sealed off during manufacture at a pressure of 1 mm of mercury at 27°C. The number of air molecules contained in the bulb is, (R = 8.31 J mol$^{-1}$ K$^{-1}$ and $N_A = 6.02 \times 10^{23}$)
- COMEDK UGET - 2024
- Physics
- Ideal gas equation
- Real gas behaves like an ideal gas at:
- JEECUP - 2024
- Chemistry
- Ideal gas equation
- The stoichiometric reaction of 516 g of dimethyldichlorosilane with water results in a tetrameric cyclic product X in 75% yield. The weight (in g) of X obtained is___. [Use, molar mass (g mol−1): H = 1, C = 12, O = 16, Si = 28, Cl = 35.5]
- JEE Advanced - 2023
- Chemistry
- Ideal gas equation
Questions Asked in JEE Main exam
A conducting bar moves on two conducting rails as shown in the figure. A constant magnetic field \( B \) exists into the page. The bar starts to move from the vertex at time \( t = 0 \) with a constant velocity. If the induced EMF is \( E \propto t^n \), then the value of \( n \) is _____.
- JEE Main - 2025
- Magnetic Field
- Concentrated nitric acid is labelled as 75%by mass. The volume in mL of the solution which contains 30 g of nitric acid is: Given: Density of nitric acid solution is 1.25 g/mL.
- JEE Main - 2025
- Solutions
- A physical quantity \( Q \) is related to four observables \( a \), \( b \), \( c \), and \( d \) as follows: \[ Q = \frac{a b^4}{c d^2} \] Where:
- \( a = (60 \pm 3) \, \text{Pa} \),
- \( b = (20 \pm 0.1) \, \text{m} \),
- \( c = (40 \pm 0.2) \, \text{N·s/m}^2 \),
- \( d = (50 \pm 0.1) \, \text{m} \).
Then the percentage error in \( Q \) is:- JEE Main - 2025
- Error analysis
- Let circle $C$ be the image of
$$ x^2 + y^2 - 2x + 4y - 4 = 0 $$
in the line
$$ 2x - 3y + 5 = 0 $$
and $A$ be the point on $C$ such that $OA$ is parallel to the x-axis and $A$ lies on the right-hand side of the centre $O$ of $C$.
If $B(\alpha, \beta)$, with $\beta < 4$, lies on $C$ such that the length of the arc $AB$ is $\frac{1}{6}$ of the perimeter of $C$, then $\beta - \sqrt{3}\alpha$ is equal to: - Assume a living cell with 0.9%(\(w/w\)) of glucose solution (aqueous). This cell is immersed in another solution having equal mole fraction of glucose and water. (Consider the data up to first decimal place only) The cell will:
- JEE Main - 2025
- osmosis
Concepts Used:
Ideal Gas Equation
An ideal gas is a theoretical gas composed of a set of randomly-moving point particles that interact only through elastic collisions.
What is Ideal Gas Law?
The ideal gas law states that the product of the pressure and the volume of one gram molecule of an ideal gas is equal to the product of the absolute temperature of the gas and the universal gas constant.
PV=nRT
where,
P is the pressure
V is the volume
n is the amount of substance
R is the ideal gas constant
Ideal Gas Law Units
When we use the gas constant R = 8.31 J/K.mol, then we have to plug in the pressure P in the units of pascals Pa, volume in the units of m3 and the temperature T in the units of kelvin K.