Question:
The value of $\displaystyle\lim_{x\to\infty}\left(\frac{\pi}{2} - \tan^{-1} x\right)^{1/x} $ is
The value of $\displaystyle\lim_{x\to\infty}\left(\frac{\pi}{2} - \tan^{-1} x\right)^{1/x} $ is
Updated On: Apr 19, 2024
- 0
- 1
- -1
- e
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
Let $y=\displaystyle\lim _{x \rightarrow \infty}\left(\frac{\pi}{2}-\tan ^{-1} x\right)$
Taking log on both sides, we get $\left(\right.$ form $\left.\frac{\infty}{\infty}\right)$
$\log y=\displaystyle\lim _{x \rightarrow \infty} \frac{1}{x} \log \left(\frac{\pi}{2}-\tan ^{-1} x\right)$
$=\displaystyle\lim _{x \rightarrow \infty} \frac{\left(-\frac{1}{1+x^{2}}\right)}{\frac{\pi}{2}-\tan ^{-1} x}\,\,\,\,$ (using L' Hospital's rule)
$=\displaystyle\lim _{x \rightarrow \infty} \frac{\frac{2 x}{\left(1+x^{2}\right)^{2}}}{-\left(\frac{1}{1+x^{2}}\right)} \,\,\,\,$ (using L' Hospital's rule)
$=\displaystyle\lim _{x \rightarrow \infty} \frac{-2 x}{1+x^{2}}=0$
$\Rightarrow y=e^{0}=1$
Taking log on both sides, we get $\left(\right.$ form $\left.\frac{\infty}{\infty}\right)$
$\log y=\displaystyle\lim _{x \rightarrow \infty} \frac{1}{x} \log \left(\frac{\pi}{2}-\tan ^{-1} x\right)$
$=\displaystyle\lim _{x \rightarrow \infty} \frac{\left(-\frac{1}{1+x^{2}}\right)}{\frac{\pi}{2}-\tan ^{-1} x}\,\,\,\,$ (using L' Hospital's rule)
$=\displaystyle\lim _{x \rightarrow \infty} \frac{\frac{2 x}{\left(1+x^{2}\right)^{2}}}{-\left(\frac{1}{1+x^{2}}\right)} \,\,\,\,$ (using L' Hospital's rule)
$=\displaystyle\lim _{x \rightarrow \infty} \frac{-2 x}{1+x^{2}}=0$
$\Rightarrow y=e^{0}=1$
Was this answer helpful?
0
0
Top Questions on limits of trigonometric functions
- If \(\lim\limits_{x \rightarrow 0} \frac{\sin(2+x)-\sin(2-x)}{x}\)= A cos B, then the values of A and B respectively are
- KCET - 2023
- Mathematics
- limits of trigonometric functions
- Let \(cos^{-1}\frac{y}{b}\)=\(log_e (\frac{x}{n})^n\), then Ay2+By1+Cy=0 is possible : (where y2=\(\frac{d^2y}{dx^2}\), y1=\(\frac{dy}{dx}\))
- WBJEE - 2023
- Mathematics
- limits of trigonometric functions
- \(\begin{array}{l}\displaystyle \lim_{ x\to 0}\left(\frac{(x+2\cos x)^3 + 2(x+2\cos x)^2 + 3 \sin(x+2\cos x)}{(x+2)^3+2(x+2)^2+3\sin(x+2)}\right)^{\frac{100}{x}} \end{array}\)is equal to _________.
- JEE Main - 2022
- Mathematics
- limits of trigonometric functions
\(\lim_{{x \to 0}} \limits\) \(\frac{cos(sin x) - cos x }{x^4}\) is equal to :
- JEE Main - 2022
- Mathematics
- limits of trigonometric functions
- If f(x) = \(\begin{vmatrix} cosx & 1 & 0 \\ 0 & 2cosx & 3 \\ 0 & 1 & 2cosx \\ \end{vmatrix}\) then \(\lim\limits_{x\rightarrow\pi} f(x)=\)
- KCET - 2021
- Mathematics
- limits of trigonometric functions
View More Questions
Questions Asked in VITEEE exam
- Two identical blocks A and B, each of mass 'm' resting on a smooth floor are connected by a light spring of natural length L and spring constant K, with the spring at its natural length. A third identical block 'C' (mass m) moving with a speed v along the line joining A and B collides with A. the maximum compression in the spring is
- VITEEE - 2023
- work, energy and power
- Assertion :
The binding energy of the nucleus increases with the increase in atomic number.
Reason :
Heavier elements have a greater number of non-radioactive isotopes than radioactive isotopes. - The half-life of radioactive radon is 3.8 days. The time at the end of which \(\frac{1}{20}th\) of the Radon sample will remain undecayed is (given log10e=0.4343)
- Which element has more electron gain enthalpy among chalcogen group?
- VITEEE - 2022
- sulphur
- Coin is tossed till heads is obtained what is expectation of no. of coin tosses
- VITEEE - 2022
- Random Variables
View More Questions
Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
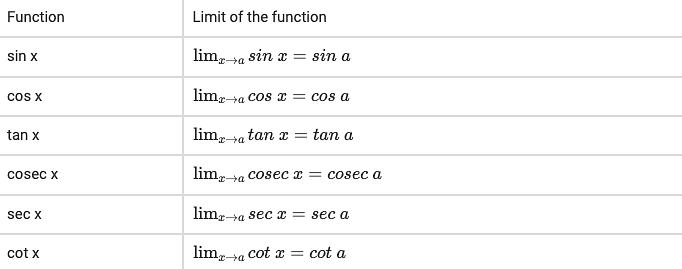
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:
