The area of the region given by A={(x,y); \(x^2\)≤y≤min{x+2,4−3x}} is
\(\frac{31}{8}\)
\(\frac{17}{6}\)
\(\frac{19}{6}\)
\(\frac{27}{8}\)
The Correct Option is B
Solution and Explanation
A = {(x, y) : x2 ≤ y ≤ min {x + 2, 4 – 3x}
So, the area of the required region
A=\(\int_{-1}^{\frac{1}{2}}\)(x+2−x2)dx+\(\int_{1}^{\frac{1}{2}}\)(4−3x−x2)dx
=[\(\frac{x^2}{2}+2x-\frac{x^3}{3}\)]\(^{\frac{1}{2}}\)-1+[4x−\(\frac{3x^2}{2}\)−\(\frac{x^3}{3}\)]\(^{\frac{1}{2}}\)1
=(\(\frac{1}{8}\)+1−\(\frac{1}{24}\))−(\(\frac{1}{2}\)−2+\(\frac{1}{3}\))+(4−\(\frac{3}{2}\)−\(\frac{1}{3}\))−(2−\(\frac{3}{8}\)−\(\frac{1}{24}\))=\(\frac{17}{6}\)
Top Questions on Area between Two Curves
- If the area of the region $ \{(x, y) : 1 + x^2 \leq y \leq \min(x + 7, 11 - 3x)\} $ is $ A $, then $ 3A $ is equal to:
- JEE Main - 2025
- Mathematics
- Area between Two Curves
- If the area of the region bounded by the curves $ y = 4 - \frac{x^2}{4} $ and $ y = \frac{x - 4}{2} $ is equal to $ \alpha $, then $ 6\alpha $ equals:
- JEE Main - 2025
- Mathematics
- Area between Two Curves
- The area of the region bounded by the curve $ y = \max\{|x|, |x-2|\} $, then x-axis and the lines x = -2 and x = 4 is equal to ____.
- JEE Main - 2025
- Mathematics
- Area between Two Curves
- The area of the region enclosed by the curves \( y = x^2 - 4x + 4 \) and \( y^2 = 16 - 8x \) is:
- JEE Main - 2025
- Mathematics
- Area between Two Curves
- The area (in sq. units) of the region bounded by the curves \( y = x^2 \) and \( y = 8 - x^2 \) is
- AP EAPCET - 2025
- Mathematics
- Area between Two Curves
Questions Asked in JEE Main exam
- Density of 3 M NaCl solution is 1.25 g/mL. The molality of the solution is:
- JEE Main - 2025
- Solutions

In the given circuit the sliding contact is pulled outwards such that the electric current in the circuit changes at the rate of 8 A/s. At an instant when R is 12 Ω, the value of the current in the circuit will be A.- JEE Main - 2025
- Electrical Circuits
- Let \( \vec{a} = \hat{i} + 2\hat{j} + \hat{k} \) and \( \vec{b} = 2\hat{i} + 7\hat{j} + 3\hat{k} \). Let \( L_1 : \vec{r} = (-\hat{i} + 2\hat{j} + \hat{k}) + \lambda \vec{a}, \lambda \in {R} \) and \( L_2 : \vec{r} = (\hat{j} + \hat{k}) + \mu \vec{b}, \mu \in \mathbb{R} \) be two lines. If the line \( L_3 \) passes through the point of intersection of \( L_1 \) and \( L_2 \), and is parallel to \( \vec{a} + \vec{b} \), then \( L_3 \) passes through the point:
- JEE Main - 2025
- Vectors
Let $ P_n = \alpha^n + \beta^n $, $ n \in \mathbb{N} $. If $ P_{10} = 123,\ P_9 = 76,\ P_8 = 47 $ and $ P_1 = 1 $, then the quadratic equation having roots $ \alpha $ and $ \frac{1}{\beta} $ is:
- JEE Main - 2025
- Relations and functions
For $ \alpha, \beta, \gamma \in \mathbb{R} $, if $$ \lim_{x \to 0} \frac{x^2 \sin \alpha x + (\gamma - 1)e^{x^2} - 3}{\sin 2x - \beta x} = 3, $$ then $ \beta + \gamma - \alpha $ is equal to:
Concepts Used:
Approximations
The theory that is part of mathematics is the approximation theory. An approximation is employed when it becomes difficult to seek out the exact value of any number. It is also essential to round off the errors resulting in approximation.
Symbol of Approximation:
In general, the wavy equal “≈” sign is used to represent the approximate values that stand for “almost equal to”.
For Example ⇢ π ≈ 3.14
Approximations of Derivatives:
Consider y = f(x) = any function of x.
Let,
Δx = the small change in x
Δy = the corresponding change in y

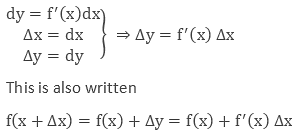
Here are some of the essential points that are required to be remembered:
- The differential of the dependent variable can not be equal to the increase of the variable whereas the differential of the independent variable can be equal to the increase of the variable.
- Absolute error in x is the change Δx.