Let the system of equations \(x+2y+3z = 5\), \(2x+3y+z = 9\), \(4x+3y+λz = μ\) have an infinite number of solutions. Then \(λ + 2μ\) is equal to
Solution and Explanation
Top Questions on types of differential equations
- If \( m_1 \) and \( m_2 \) are the slopes of the direct common tangents drawn to the circles \[ x^2 + y^2 - 2x - 8y + 8 = 0 \quad \text{and} \quad x^2 + y^2 - 8x + 15 = 0 \] then \( m_1 + m_2 \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If \( (2,3) \) is the focus and \( x - y + 3 = 0 \) is the directrix of a parabola, then the equation of the tangent drawn at the vertex of the parabola is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If the focus of an ellipse is \((-1,-1)\), equation of its directrix corresponding to this focus is \(x + y + 1 = 0\) and its eccentricity is \(\frac{1}{\sqrt{2}}\), then the length of its major axis is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The equation of the common tangent to the parabola \(y^2 = 8x\) and the circle \(x^2 + y^2 = 2\) is \(ax + by + 2 = 0\). If \(-\frac{a}{b}>0\), then \(3a^2 + 2b + 1 =\)
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The axis of a parabola is parallel to Y-axis. If this parabola passes through the points \( (1,0), (0,2), (-1,-1) \) and its equation is \( ax^2 + bx + cy + d = 0 \), then \( \frac{ad}{bc} \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
Questions Asked in JEE Main exam
- The electric field of an electromagnetic wave in free space is \[ \vec{E} = 57 \cos \left[7.5 \times 10^6 t - 5 \times 10^{-3} (3x + 4y)\right] \left( 4\hat{i} - 3\hat{j} \right) \, \text{N/C}. \] The associated magnetic field in Tesla is:
- JEE Main - 2025
- Electromagnetic Induction and Inductance
- Find the equivalent resistance between two ends of the following circuit:
- JEE Main - 2025
- Current electricity
Given below are two statements. One is labelled as Assertion (A) and the other is labelled as Reason (R):
Assertion (A): In an insulated container, a gas is adiabatically shrunk to half of its initial volume. The temperature of the gas decreases.
Reason (R): Free expansion of an ideal gas is an irreversible and an adiabatic process.In the light of the above statements, choose the correct answer from the options given below:
- JEE Main - 2025
- Current electricity
- Find the output voltage in the given circuit.
- JEE Main - 2025
- Current electricity
- A wire of length $ 25 \, \text{m} $ and cross-sectional area $ 5 \, \text{mm}^2 $ having resistivity $ 2 \times 10^{-6} \, \Omega \cdot \text{m} $ is bent into a complete circle. The resistance between diametrically opposite points will be:
- JEE Main - 2025
- Current electricity
Concepts Used:
Types of Differential Equations
There are various types of Differential Equation, such as:
Ordinary Differential Equations:
Ordinary Differential Equations is an equation that indicates the relation of having one independent variable x, and one dependent variable y, along with some of its other derivatives.
\(F(\frac{dy}{dt},y,t) = 0\)
Partial Differential Equations:
A partial differential equation is a type, in which the equation carries many unknown variables with their partial derivatives.
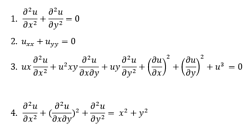
Linear Differential Equations:
It is the linear polynomial equation in which derivatives of different variables exist. Linear Partial Differential Equation derivatives are partial and function is dependent on the variable.
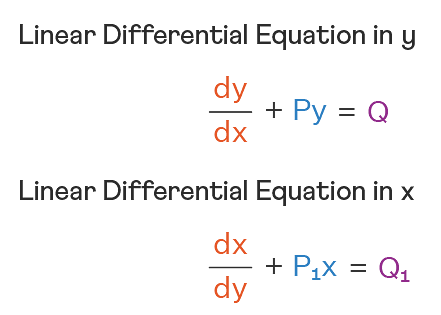
Homogeneous Differential Equations:
When the degree of f(x,y) and g(x,y) is the same, it is known to be a homogeneous differential equation.
\(\frac{dy}{dx} = \frac{a_1x + b_1y + c_1}{a_2x + b_2y + c_2}\)
Read More: Differential Equations