Question:
In a series LCR circuit connected to an AC source, at resonance, the current is maximum because:
In a series LCR circuit connected to an AC source, at resonance, the current is maximum because:
Show Hint
At resonance in a series LCR circuit, inductive and capacitive reactances cancel, minimizing impedance and maximizing current.
Updated On: Apr 14, 2025
- The inductive reactance is maximum
- The capacitive reactance cancels the inductive reactance
- The resistance is zero
- The reactances add up
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
In a series LCR circuit, the total impedance (\( Z \)) is given by:
\[ Z = \sqrt{R^2 + (X_L - X_C)^2} \]
where:
- \( R \) is the resistance
- \( X_L = \omega L \) is the inductive reactance
- \( X_C = \frac{1}{\omega C} \) is the capacitive reactance
At resonance, \( X_L = X_C \), so:
\[ Z = \sqrt{R^2 + (X_L - X_C)^2} = \sqrt{R^2} = R \]
The impedance is minimized, leading to maximum current as per Ohm's law:
\[ I = \frac{V}{Z} \]
Hence, the current is maximum because inductive and capacitive reactances cancel each other.
Was this answer helpful?
0
0
Top Questions on Amperes circuital law
- A coil has a resistance of \( 30 \, \Omega \) and an inductive reactance of \( 20 \, \Omega \) at 50 Hz frequency. If an AC source of 200 V and 100 Hz is connected across the coil, then how much current will flow through the coil?
- MHT CET - 2024
- Physics
- Amperes circuital law
Consider the circuit shown :
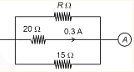 The ammeter reads 0.9 A. Value of R is
The ammeter reads 0.9 A. Value of R is- JEE Main - 2024
- Physics
- Amperes circuital law
- Among the following, Ampere’s circuital law is represented by:
- AP EAPCET - 2023
- Physics
- Amperes circuital law
- In the given circuit, find the voltage drop VL in the load resistance RL
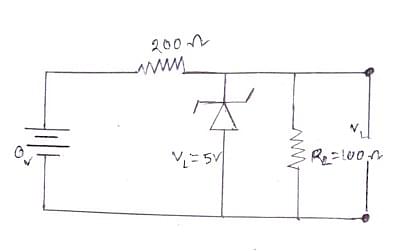
- WBJEE - 2023
- Physics
- Amperes circuital law
- A transistor is used in an amplifier circuit in common emitter mode. If the base current changes by 100μA, it brings a change of 10mA in collector current. If the load resistance is 2kΩ and input resistance is 1kΩ, the value of power gain is \(x×10^4\). The value of x is _______.
- JEE Main - 2022
- Physics
- Amperes circuital law
View More Questions
Questions Asked in MHT CET exam
- A die was thrown \( n \) times until the lowest number on the die appeared. If the mean is \( \frac{n}{g} \), then what is the value of \( n \)?
- MHT CET - 2025
- Probability
- How many molecules of carbon dioxide are formed when 0.6 g carbon is burnt in air?
- MHT CET - 2025
- Stoichiometry and Stoichiometric Calculations
- A wire has a resistance of \( 10 \, \Omega \) at \( 20^\circ \text{C} \). If the temperature coefficient of resistance of the material is \( 0.004 \, \text{per}^\circ \text{C} \), what is the resistance of the wire at \( 50^\circ \text{C} \)?
- MHT CET - 2025
- Current electricity
- A simple pendulum has a length of $ L = 2 \, \text{m} $. What is the time period of the pendulum? (Assume $ g = 9.8 \, \text{m/s}^2 $)
- MHT CET - 2025
- Waves and Oscillations
- In the Miller-Urey experiment, what was the ratio of gases used to simulate primitive Earth's atmosphere?
- MHT CET - 2025
- Origin Of Life
View More Questions