A transistor is used in an amplifier circuit in common emitter mode. If the base current changes by 100μA, it brings a change of 10mA in collector current. If the load resistance is 2kΩ and input resistance is 1kΩ, the value of power gain is \(x×10^4\). The value of x is _______.
Correct Answer: 2
Solution and Explanation
The correct answer is: 2
Power gain
\(=[\frac{\triangle{ic}}{\triangle{iB}}]×\frac{R_0}{R_i}\)
\(=[\frac{10^{-2}}{10^{-4}}]×\frac{2}{1}\)
= 2 × 104
\(⇒ x = 2\)
Top Questions on Amperes circuital law
- A coil has a resistance of \( 30 \, \Omega \) and an inductive reactance of \( 20 \, \Omega \) at 50 Hz frequency. If an AC source of 200 V and 100 Hz is connected across the coil, then how much current will flow through the coil?
- MHT CET - 2024
- Physics
- Amperes circuital law
- In a series LCR circuit connected to an AC source, at resonance, the current is maximum because:
- MHT CET - 2024
- Physics
- Amperes circuital law
Consider the circuit shown :
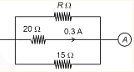The ammeter reads 0.9 A. Value of R is- JEE Main - 2024
- Physics
- Amperes circuital law
- Among the following, Ampere’s circuital law is represented by:
- AP EAPCET - 2023
- Physics
- Amperes circuital law
- In the given circuit, find the voltage drop VL in the load resistance RL
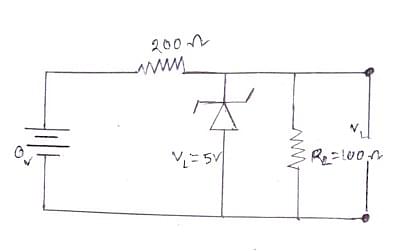
- WBJEE - 2023
- Physics
- Amperes circuital law
Questions Asked in JEE Main exam
- If the first term of an A.P. is 3 and the sum of its first four terms is equal to one-fifth of the sum of the next four terms, then the sum of the first 20 terms is equal to:
- JEE Main - 2025
- Arithmetic Progression
The number of 6-letter words, with or without meaning, that can be formed using the letters of the word MATHS such that any letter that appears in the word must appear at least twice, is $ 4 \_\_\_\_\_$.
- JEE Main - 2025
- permutations and combinations
Let \( S = \left\{ m \in \mathbb{Z} : A^m + A^m = 3I - A^{-6} \right\} \), where
\[ A = \begin{bmatrix} 2 & -1 \\ 1 & 0 \end{bmatrix} \]Then \( n(S) \) is equal to ______.
- JEE Main - 2025
- Matrix Algebra
Let \( A = \{-3, -2, -1, 0, 1, 2, 3\} \). A relation \( R \) is defined such that \( xRy \) if \( y = \max(x, 1) \). The number of elements required to make it reflexive is \( l \), the number of elements required to make it symmetric is \( m \), and the number of elements in the relation \( R \) is \( n \). Then the value of \( l + m + n \) is equal to:
- JEE Main - 2025
- Sets and Relations
- Let \( X = {R} \times {R} \). Define a relation \( R \) on \( X \) as: \[ (a_1, b_1) \, R \, (a_2, b_2) \iff b_1 = b_2. \] Statement-I: \( R \) is an equivalence relation. Statement-II: For some \( (a, b) \in X \), the set \( S = \{(x, y) \in X : (x, y) R (a, b)\ \) represents a line parallel to \( y = x \).}
- JEE Main - 2025
- Relations and Geometry
Concepts Used:
Amperes circuital law
According to Ampere’s law, magnetic fields are related to the electric current that is produced in them. This law specifies that the magnetic field is associated with a given current or vice-versa, provided that the electric field doesn’t change with time.
Ampere’s circuital law can be written as the line integral of the magnetic field surrounding the closed loop which is equal to the number of times the algebraic sum of currents passing through the loop.
According to the second equation, if the magnetic field is integrated along the blue path, then it is equal to the current enclosed, I.
The magnetic field doesn’t vary at a distance r because of symmetry. The path length (in blue) in figure 1 has to be equal to the circumference of a circle,2πr.
