If the orthocentre of the triangle, whose vertices are $(1,2),(2,3)$ and $(3,1)$ is $(\alpha, \beta)$, then the quadratic equation whose roots are $\alpha+4 \beta$ and $4 \alpha+\beta$, is
- $x^2-19 x+90=0$
- $x^2-20 x+99=0$
- $x^2-18 x+80=0$
- $x^2-22 x+120=0$
The Correct Option is B
Approach Solution - 1
The correct answer is option (B) : x2−20x+99=0

\((\frac{β−3}{α−2})(\frac{1}{−2})=−1\)
\(β−3=2α−4\)
\(β=2α−1\)
\(m_{AH}\times m_{BC}=−1\)
\(⇒(\frac{β−2}{α−1})(−2)=−1\)
\(⇒2β−4=α−1\)
\(⇒2(2α−1)=α+3\)
\(⇒3α=5\)
\(α=\frac{5}{3}, β=\frac{7}{3} \)
\(⇒H(\frac{5}{3}, \frac{7}{3})\)
\(α+4β= \frac{5}{3} + \frac{28}{3} = \frac{33}{3} =11\)
\(β+4α= \frac{7}{3} + \frac{20}{3} = \frac{27}{3} = 9\)
\(x^2 −20x+99=0\)
Approach Solution -2
Step 1: Finding slopes of perpendicular altitudes: \[ m = \frac{-1}{2} = -\frac{1}{2} \] \[ \text{Here } m_{BH} \times m_{AC} = -1 \] \[ \beta - 3 = 2\alpha - 4 \] \[ \beta = 2\alpha - 1 \] Step 2: Using midpoint formula and relations, we find: \[ \alpha = \frac{5}{3}, \quad \beta = \frac{7}{3} \]
Step 3: Now, computing roots: \[ \alpha + 4\beta = 11, \quad 4\alpha + \beta = 9 \] \[ x^2 - (11 + 9)x + 11 \times 9 = 0 \] \[ x^2 - 20x + 99 = 0 \]
Top Questions on Definite Integral
- Evaluate the definite integral: \( \int_{-2}^{2} |x^2 - x - 2| \, dx \)
- MHT CET - 2025
- Mathematics
- Definite Integral
The value \( 9 \int_{0}^{9} \left\lfloor \frac{10x}{x+1} \right\rfloor \, dx \), where \( \left\lfloor t \right\rfloor \) denotes the greatest integer less than or equal to \( t \), is ________.
- JEE Main - 2024
- Mathematics
- Definite Integral
- Evaluate the integral: \[ \int e^x \left( \frac{x + 2}{(x+4)} \right)^2 dx. \]
- AP EAMCET - 2024
- Mathematics
- Definite Integral
- Evaluate the integral $$ I = \int_1^5 \left( |x - 3| + |1 - x| \right) \, dx $$
- AP EAMCET - 2024
- Mathematics
- Definite Integral
- Evaluate the integral \[ \int_{0}^{\frac{\pi}{4}} \frac{x^2}{(x \sin x + \cos x)^2} dx. \]
- AP EAMCET - 2024
- Mathematics
- Definite Integral
Questions Asked in JEE Main exam
- Let \( f: \mathbb{R} \to \mathbb{R} \) be a function defined by \( f(x) = \left( 2 + 3a \right)x^2 + \left( \frac{a+2}{a-1} \right)x + b, a \neq 1 \). If \[ f(x + y) = f(x) + f(y) + 1 - \frac{2}{7}xy, \] then the value of \( 28 \sum_{i=1}^5 f(i) \) is:
- JEE Main - 2025
- Functions
Let $ f: \mathbb{R} \to \mathbb{R} $ be a twice differentiable function such that $$ f''(x)\sin\left(\frac{x}{2}\right) + f'(2x - 2y) = (\cos x)\sin(y + 2x) + f(2x - 2y) $$ for all $ x, y \in \mathbb{R} $. If $ f(0) = 1 $, then the value of $ 24f^{(4)}\left(\frac{5\pi}{3}\right) $ is:
- JEE Main - 2025
- Differential Calculus
- A 400 g solid cube having an edge of length \(10\) cm floats in water. How much volume of the cube is outside the water? (Given: density of water = \(1000 { kg/m}^3\))
- An infinite wire has a circular bend of radius \( a \), and carrying a current \( I \) as shown in the figure. The magnitude of the magnetic field at the origin \( O \) of the arc is given by:

- JEE Main - 2025
- Magnetic Field
- Let \( A = [a_{ij}] \) be a matrix of order 3 \(\times\) 3, with \(a_{ij} = (\sqrt{2})^{i+j}\). If the sum of all the elements in the third row of \( A^2 \) is \( \alpha + \beta\sqrt{2} \), where \(\alpha, \beta \in \mathbb{Z}\), then \(\alpha + \beta\) is equal to:
- JEE Main - 2025
- Matrices and Determinants
Concepts Used:
Definite Integral
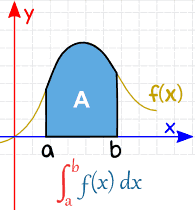
Definite integral is an operation on functions which approximates the sum of the values (of the function) weighted by the length (or measure) of the intervals for which the function takes that value.
Definite integrals - Important Formulae Handbook
A real valued function being evaluated (integrated) over the closed interval [a, b] is written as :
\(\int_{a}^{b}f(x)dx\)
Definite integrals have a lot of applications. Its main application is that it is used to find out the area under the curve of a function, as shown below: