If \(∠\) A and \(∠\) B are acute angles such that cos A = cos B, then show that
\(∠ A = ∠ B\).
\(∠ A = ∠ B\).
Solution and Explanation
Let us consider a triangle ABC in which CD \(⊥\) AB.

It is given that
cos A = cos B
\(⇒\frac{AD}{AC}=\frac{BD}{BC}.....(1)\)
We have to prove \(∠A = ∠B\).

To prove this, let us extend AC to P such that BC = CP.
From equation (1), we obtain
\(\frac{AD}{BD}=\frac{AC}{BC} \)
\(⇒\frac{AD}{BD}=\frac{AC}{CP}......(2)\)
By using the converse of B.P.T,
\(CD||BP \)
\(⇒∠ACD = ∠CPB\) (Corresponding angles) … (3)
And \(∠BCD = ∠CBP\) (Alternate interior angles) … (4)
By construction, we have BC = CP.
\(∴ ∠CBP = ∠CPB \) (Angle opposite to equal sides of a triangle) … (5)
From equations (3), (4), and (5), we obtain
\(∠ACD = ∠BCD … (6)\)
In\( ΔCAD\) and \(ΔCBD, \)
\(∠ACD = ∠BCD\) [Using equation (6)]
\(∠CDA = ∠CDB [Both\ 90°]\)
Therefore, the remaining angles should be equal.
\(∴∠CAD = ∠CBD \)
\(⇒ ∠A = ∠B\)
Alternatively,
Let us consider a triangle ABC in which \(CD ⊥ AB.\)

It is given that,
cos A = cos B
\(⇒\frac{AD}{AC}=\frac{BD}{BC}\)
\(⇒ \frac{AD}{BD}=\frac{AC}{BC}\)
Let\( \frac{AD}{BD}=\frac{AC}{BC}=k\)
\(⇒ AD = k BD … (1) \)
And,\( AC = k BC … (2) \)
Using Pythagoras theorem for triangles CAD and CBD, we obtain
\((\text{CD}) ^2 =(\text{ AC})^ 2 -(\text{ AD})^ 2 … (3) \)
And, \((\text{CD}) ^2 =(\text{ BC}) ^2 - (\text{BD})^ 2 … (4)\)
From equations (3) and (4), we obtain
\((AC)^ 2 -( AD)^ 2 = (BC)^ 2 - (BD )^2 \)
\(⇒ (k\ BC) ^2 - (k\ BD)^ 2 = (BC)^ 2 - (BD)^ 2 \)
\(⇒ k^ 2 (BC ^2 - BD ^2 ) = BC ^2 - BD^ 2 \)
\(⇒ k ^2 = 1\)
\(⇒ k = 1 \)
Putting this value in equation (2), we obtain
AC = BC
\(⇒ ∠A = ∠B\) (Angles opposite to equal sides of a triangle)
Top Questions on Trigonometric Ratios
If $\tan \theta = \tfrac{3}{4}$, then the value of $\cos \theta$ will be:
- UP Board X - 2025
- Mathematics
- Trigonometric Ratios
- Evaluate \( \dfrac{2 \tan 30^\circ}{1 + \tan^2 30^\circ} \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- If \( 15 \cot A = 8 \), then \( \sin A = \, ? \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- Evaluate \( (\sec A + \tan A)(1 - \sin A) = \ ? \)
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
- Which of the following is true?
- AP POLYCET - 2025
- Mathematics
- Trigonometric Ratios
Questions Asked in CBSE X exam
‘दीवार खड़ी करना’ मुहावरे का वाक्य में इस प्रकार प्रयोग करें कि अर्थ स्पष्ट हो जाए।
Select from the following a statement which is not true about the burning of magnesium ribbon in air:
- CBSE Class X - 2025
- Chemical Reactions
- Imagine you are a part of a 'Self Help Group' (SHG). Explain the working system of the Self Help Group (SHG) to the new member.
- CBSE Class X - 2025
- Money and Credit
Analyze the significant changes in printing technology during 19th century in the world.
- CBSE Class X - 2025
- Print Culture and the Modern World
निम्नलिखित विषय पर संकेत बिंदुओं के आधार पर लगभग 120 शब्दों में एक अनुच्छेद लिखिए |
डिजिटल इंडियासंकेत बिंदु -
डिजिटल इंडिया क्या है
डिजिटल होने के लाभ
सरकार द्वारा उठाए गए कदम- CBSE Class X - 2025
- पत्र लेखन
Concepts Used:
Trigonometric Functions
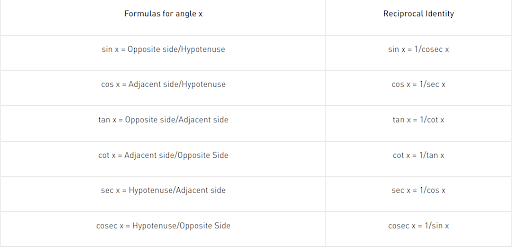
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions: