If \(\frac{1}{6}\)sinθ.cosθ.tanθ are in G.P, then the solution set θ is
- \(2n\pi\pm\frac{\pi}{6}\)
- \(2n\pi\pm\frac{\pi}{3}\)
- \(n\pi+(-1)^n\frac{\pi}{3}\)
- \(n\pi+\frac{\pi}{3}\)
The Correct Option is B
Approach Solution - 1
Correct answer: Option (B): $2n\pi \pm \frac{\pi}{3}$
Given that $a$, $b$, and $c$ are in GP, we know that:
$b^2 = ac$
Also given: $\tan\theta \cdot \sin\theta \cdot \frac{1}{6} = \cos^2\theta$
We use the identity: $\tan\theta = \frac{\sin\theta}{\cos\theta}$
$\Rightarrow \frac{\sin\theta}{\cos\theta} \cdot \sin\theta \cdot \frac{1}{6} = \cos^2\theta$
$\Rightarrow \frac{\sin^2\theta}{6\cos\theta} = \cos^2\theta$
Multiplying both sides by $6\cos\theta$:
$\Rightarrow \sin^2\theta = 6\cos^3\theta$
Now using the identity $\sin^2\theta = 1 - \cos^2\theta$:
$\Rightarrow 1 - \cos^2\theta = 6\cos^3\theta$
Rewriting:
$\Rightarrow 6\cos^3\theta + \cos^2\theta - 1 = 0$
Trying $\cos\theta = \frac{1}{2}$:
$6 \cdot \left(\frac{1}{2}\right)^3 + \left(\frac{1}{2}\right)^2 - 1 = \frac{3}{4} + \frac{1}{4} - 1 = 0$ ✅
So, $\cos\theta = \frac{1}{2}$ is a solution.
General solution: $\theta = 2n\pi \pm \frac{\pi}{3}$
Approach Solution -2
Given that $\sin \theta$, $\cos \theta$, and $\tan \theta$ form a geometric progression (G.P.), we start with:
$\cos^2 \theta = \frac{1}{6} \sin \theta \tan \theta$
$\Rightarrow \cos^2 \theta = \frac{1}{6} \cdot \sin^2 \theta \cdot \frac{1}{\cos \theta}$
$\Rightarrow \cos^3 \theta = \frac{\sin^2 \theta}{6}$
Now use the identity: $\sin^2 \theta = 1 - \cos^2 \theta$
$\Rightarrow 6 \cos^3 \theta = 1 - \cos^2 \theta$
$\Rightarrow 6 \cos^3 \theta + \cos^2 \theta - 1 = 0$
Factor the cubic:
$\Rightarrow (\cos \theta - \frac{1}{2})(6 \cos^2 \theta + 4 \cos \theta + 2) = 0$
$\Rightarrow 2 (\cos \theta - \frac{1}{2})(3 \cos^2 \theta + 2 \cos \theta + 1) = 0$
Solving: $\cos \theta = \frac{1}{2} \Rightarrow \cos \theta = \cos \frac{\pi}{3}$
Hence, general solution: $\theta = 2n\pi \pm \frac{\pi}{3}$
Top Questions on Trigonometric Functions
- Solve for \( x \), \[ 2 \tan^{-1} x + \sin^{-1} \left( \frac{2x}{1 + x^2} \right) = 4\sqrt{3} \]
- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
The graph shown below depicts:

- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
- Evaluate: \( \sin^{-1} \left( \sin \frac{3\pi}{5} \right) \).
- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
- Evaluate: $ \tan^{-1} \left[ 2 \sin \left( 2 \cos^{-1} \frac{\sqrt{3}}{2} \right) \right]$
- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
- The graph of a trigonometric function is as shown. Which of the following will represent the graph of its inverse?

- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
Questions Asked in WBJEE exam
- A force \( \mathbf{F} = ai + bj + ck \) is acting on a body of mass \( m \). The body was initially at rest at the origin. The co-ordinates of the body after time \( t \) will be:
- WBJEE - 2025
- Newtons Laws of Motion
A quantity \( X \) is given by: \[ X = \frac{\epsilon_0 L \Delta V}{\Delta t} \] where:
- \( \epsilon_0 \) is the permittivity of free space,
- \( L \) is the length,
- \( \Delta V \) is the potential difference,
- \( \Delta t \) is the time interval.
The dimension of \( X \) is the same as that of:- WBJEE - 2025
- Dimensional Analysis
- Ruma reached the metro station and found that the escalator was not working. She walked up the stationary escalator with velocity \( v_1 \) in time \( t_1 \). On another day, if she remains stationary on the escalator moving with velocity \( v_2 \), the escalator takes her up in time \( t_2 \). The time taken by her to walk up with velocity \( v_1 \) on the moving escalator will be:
- WBJEE - 2025
- Relative Motion
- Which logic gate is represented by the following combination of logic gates?

- WBJEE - 2025
- Logic gates
- If \( {}^9P_3 + 5 \cdot {}^9P_4 = {}^{10}P_r \), then the value of \( 'r' \) is:
- WBJEE - 2025
- permutations and combinations
Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
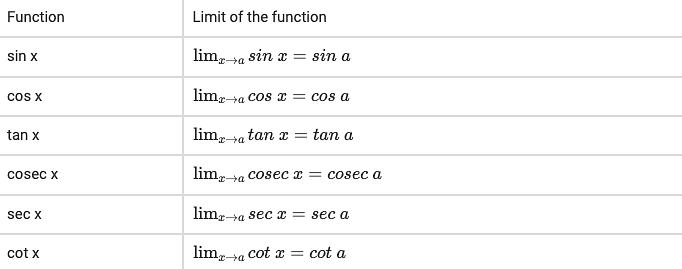
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:
