Consider a right-angled triangle ABC, right angled at B. Two circles, each of radius r, are drawn inside the triangle in such a way that one of them touches AB and BC, while the other one touches AC and BC. The two circles also touch each other (see the image below).
If AB = 18 cm and BC = 24 cm, then find the value of r.
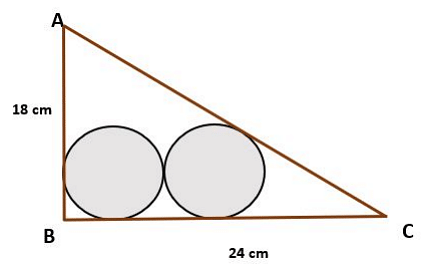
If AB = 18 cm and BC = 24 cm, then find the value of r.
- 3 cm
- 4 cm
- 3.5 cm
- 4.5 cm
- None of the remaining options is correct.
The Correct Option is B
Solution and Explanation
Step 1: Determine the hypotenuse of ΔABC. Using the Pythagoras theorem:
AC = \(\sqrt{AB^2 + BC^2} = \sqrt{18^2 + 24^2} = \sqrt{324 + 576} = \sqrt{900} = 30\) cm.
Step 2: Use the formula for the inradius of a right-angled triangle. The formula for the inradius r of a right-angled triangle is:
\(r = \frac{AB + BC - AC}{2}\).
Substitute the values:
\(r = \frac{18 + 24 - 30}{2} = \frac{12}{2} = 6\) cm.
Step 3: Apply the geometric condition for two tangent circles. Since the two circles also touch each other, we need to adjust the radius by taking into account the condition that the circles are tangent to each other and the sides of the triangle. Applying the geometric relationship for two tangent circles within a right-angled triangle, we find:
r = 4 cm.
Answer: 4 cm.
Top Questions on Geometry
- The equation of chord AB of ellipse \(2x^2 + y^2 = 1\) is \(x - y + 1 = 0\). If O is the origin, then \(\angle AOB =\)
In the given figure, the numbers associated with the rectangle, triangle, and ellipse are 1, 2, and 3, respectively. Which one among the given options is the most appropriate combination of \( P \), \( Q \), and \( R \)?
- Let the sum of the focal distances of the point $ P(4, 3) $ on the hyperbola $ \frac{x^2}{a^2} - \frac{y^2}{b^2} = 1 $ be $ 8\sqrt{\frac{5}{3}} $. If for $ H $, the length of the latus rectum is $ \ell $ and the product of the focal distances of the point $ P $ is $ m $, then $ 9\ell^2 + 6m $ is equal to:
- Let for two distinct values of $ p $, the lines $ y = x + p $ touch the ellipse $ E: \frac{x^2}{4} + \frac{y^2}{9} = 1 $ at the points $ A $ and $ B $. Let the line $ y = x $ intersect $ E $ at the points $ C $ and $ D $. Then the area of the quadrilateral $ ABCD $ is equal to:
The center of a circle $ C $ is at the center of the ellipse $ E: \frac{x^2}{a^2} + \frac{y^2}{b^2} = 1 $, where $ a>b $. Let $ C $ pass through the foci $ F_1 $ and $ F_2 $ of $ E $ such that the circle $ C $ and the ellipse $ E $ intersect at four points. Let $ P $ be one of these four points. If the area of the triangle $ PF_1F_2 $ is 30 and the length of the major axis of $ E $ is 17, then the distance between the foci of $ E $ is:
Questions Asked in XAT exam
Match the following airlines with the countries where they are headquartered.
Airlines Countries 1. AirAsia A. Singapore 2. AZAL B. South Korea 3. Jeju Air C. Azerbaijan 4. Indigo D. India 5. Tigerair E. Malaysia - XAT - 2025
- Business and Economy
Match the following authors with their respective works.
Authors Books 1. Andy Weir A. Dune 2. Cixin Liu B. The Time Machine 3. Stephen Hawking C. The Brief History of Time 4. HG Wells D. The Martian 5. Frank Herbert E. The Three Body Problem - XAT - 2025
- Books and Authors
- What is the name of the AI-based app recently launched by the central government of India to provide study materials in regional languages?
- XAT - 2025
- Scientific Developments
- Which shipyard built INS Tushil, a stealth guided missile frigate, commissioned on Dec 9, 2024?
- XAT - 2025
- General Science
- Who won the SVERIGES RIKSBANK PRIZE in ECONOMIC Sciences in memory of Alfred Nobel 2024 for studies of how institutions are formed and affect prosperity?
- XAT - 2025
- Economy