$AB$ and $CD$ are $2$ line segments ; where $A(2,3,0),B (6, 9, 0), C(-6, -9, 0). P $ and $Q$ are midpoint of $AB$ and $CD$, respectively and $L$ is the midpoint of $PQ$. Find the distance of $L$ from the plane $3x + 4z + 25 = 0$
- 25
- 15
- 5
- 40
The Correct Option is C
Approach Solution - 1
Using mid-point formula
$Q=\left(\frac{x-6}{2}, \frac{y-9}{2} , \frac{z+0}{2}\right) $
Also, $P=\left(\frac{2+6}{2}, \frac{3+9}{2}, \frac{0+0}{2}\right) =\left(4,6,0\right)$
Since, $AC||PQ$
$ \therefore$ D.r's of line $AC $ = D.r's of line $PQ$
$\Rightarrow \:\: \left(-8 ,12,0\right)=\left(\frac{x-14}{2}, \frac{y-21}{2} , \frac{z}{2}\right) $
$\Rightarrow\:\: x = -2, y = -3, z = 0$
$\Rightarrow\:\:\: D (-2, -3,0) \:\:\: \Rightarrow \:\: Q(-4, - 6,0)$
If $L$ is midpoint of $PQ$ then
$L\left(\frac{4-4}{2}, \frac{6-6}{2}, 0\right)=\left(0,0,0\right)$
$ \therefore$ $ \perp$ distance of $L(0,0,0)$ from the plane $3x + 4z + 25 = 0$ is
$=\left|\frac{3\left(0\right)+4\left(0\right)+25}{\sqrt{\left(3\right)^{2}+\left(4\right)^{2}}}\right|= \left|\frac{25}{\sqrt{25}}\right| =\sqrt{25} =5$
Approach Solution -2
Ans. The word "midpoint" has been used to refer to the exact "center" of anything since the early 14th century. The center point is where the two ends meet. It may also be referred to as the line segment's centroid and its ends.
The midpoint theorem states that "The line segment in a triangle joining the midpoint of two sides of the triangle is said to be parallel to its third side and is also half of the length of the third side."
The midpoint theorem came to be invented by Rene Descartes, the most popular mathematician of all time. It was a basic geometrical idea at first in Rene’s mind, which represented an ordered pair of numbers. This was later made into a method to help combine arithmetic and geometry. Apart from the cartesian plane, the midpoint has great uses in geometrical figures like triangles, circles, ellipses, hyperbolas, quadrilaterals, and general polygons.
Let’s suppose that a line connects two points (2,6) and (4,2), then the coordinates of the midpoint of the line joining these two points are, [(2+4)/2, (6+2)/2] which gives us (3,4).

Top Questions on Three Dimensional Geometry
- If A(2,1,-1), B(6,-3,2), C(-3,12,4) are the vertices of a triangle ABC and the equation of the plane containing the triangle ABC is $53x+by+cz+d=0$, then $\frac{d}{b+c}=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- If $(\alpha, \beta, \gamma)$ is the foot of the perpendicular drawn from a point $(-1,2,-1)$ to the line joining the points $(2,-1,1)$ and $(1,1,-2)$, then $\alpha+\beta+\gamma=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- If m:n is the ratio in which the point $\left(\frac{8}{5}, \frac{1}{5}, \frac{8}{5}\right)$ divides the line segment joining the points (2,p,2) and (p,-2,p) where p is an integer then $\frac{3m+n}{3n}=$
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
- Let $\pi_1$ be the plane determined by the vectors $\hat{i}+\hat{j}, \hat{i}+\hat{k}$ and $\pi_2$ be the plane determined by the vectors $\hat{j}-\hat{k}, \hat{k}-\hat{i}$. Let $\vec{a}$ be a non-zero vector parallel to the line of intersection of the planes $\pi_1$ and $\pi_2$. If $\vec{b} = \hat{i}+\hat{j}-\hat{k}$ then the angle between the vectors $\vec{a}$ and $\vec{b}$ is
- TS EAMCET - 2025
- Mathematics
- Three Dimensional Geometry
Show that the following lines intersect. Also, find their point of intersection:
Line 1: \[ \frac{x - 1}{2} = \frac{y - 2}{3} = \frac{z - 3}{4} \]
Line 2: \[ \frac{x - 4}{5} = \frac{y - 1}{2} = z \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
Questions Asked in COMEDK UGET exam
- A satellite is revolving around the earth in a circular orbit with kinetic energy of $1.69 \times 10^{10}$ J. The additional kinetic energy required for just escaping into the outer space is
- COMEDK UGET - 2024
- Escape Speed
A solid cylinder of mass 2 kg and radius 0.2 m is rotating about its own axis without friction with angular velocity 5 rad/s. A particle of mass 1 kg moving with a velocity of 5 m/s strikes the cylinder and sticks to it as shown in figure.

The angular velocity of the system after the particle sticks to it will be:- COMEDK UGET - 2024
- The Angular Momentum
- In a given semiconductor, the ratio of the number density of electron to number density of hole is 2 : 1. If $ \frac{1}{7} $th of the total current is due to the hole and the remaining is due to the electrons, the ratio of the drift velocity of holes to the drift velocity of electrons is :
- COMEDK UGET - 2024
- Semiconductors
- A transformer which steps down 330 V to 33 V is to operate a device having impedance 110 $\Omega$. The current drawn by the primary coil of the transformer is:
- COMEDK UGET - 2024
- Transformers
- In the Young's double slit experiment, the 2nd bright fringe for red light coincides with the 3rd bright fringe for violet light. Then the value of 'n' is: (Given: wavelength of red light = 6300 $\text{Å}$ and wavelength of violet light = 4200 $\text{Å}$)
- COMEDK UGET - 2024
- Youngs double slit experiment
Concepts Used:
Three Dimensional Geometry
Mathematically, Geometry is one of the most important topics. The concepts of Geometry are derived w.r.t. the planes. So, Geometry is divided into three major categories based on its dimensions which are one-dimensional geometry, two-dimensional geometry, and three-dimensional geometry.
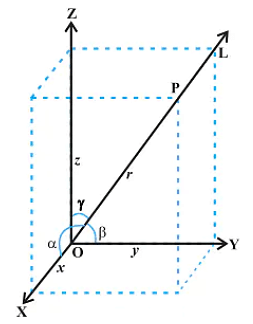
Direction Cosines and Direction Ratios of Line:
Consider a line L that is passing through the three-dimensional plane. Now, x,y and z are the axes of the plane and α,β, and γ are the three angles the line makes with these axes. These are commonly known as the direction angles of the plane. So, appropriately, we can say that cosα, cosβ, and cosγ are the direction cosines of the given line L.