A metallic rod of length 1 m held along east-west direction is allowed to fall down freely. Given horizontal component of earth’s magnetic field BH = 3 × 10-5 T. The emf induced in the rod at an instant t = 2s after it is released is ( Take g = 10 ms-2 )
- 3 × 10-3 V
- 3 × 10-4 V
- 6 × 10-3 V
- 6 × 10-4 V
The Correct Option is D
Approach Solution - 1
Given Information:
Length of rod, \( l = 1\,m \)
Horizontal component of Earth's magnetic field, \( B_H = 3 \times 10^{-5}\,T \)
Acceleration due to gravity, \( g = 10\,ms^{-2} \)
Time after release, \( t = 2\,s \)
Step-by-Step Explanation:
Step 1: Find velocity (\(v\)) of the rod after time \(t\):
Rod is falling freely under gravity, thus initial velocity \( u = 0 \), so velocity after \( t \) seconds is:
\[ v = u + gt = 0 + 10 \times 2 = 20\,m/s \]
Step 2: Calculate induced emf (\(E\)):
The induced emf in a rod moving perpendicular to a magnetic field is given by:
\[ E = B_H \times l \times v \]
Substitute values into this formula:
\[ E = (3 \times 10^{-5}\,T) \times (1\,m) \times (20\,m/s) \]
Simplify:
\[ E = 6 \times 10^{-4}\,V \]
Final Conclusion:
The induced emf in the rod at \( t = 2\,s \) is \(6 \times 10^{-4}\,V\).
Approach Solution -2
The emf induced in a falling rod is given by the formula: \[ \text{emf} = B \cdot L \cdot v \] Where: - \( B \) is the magnetic field strength, - \( L \) is the length of the rod, - \( v \) is the velocity of the rod at time \( t \). The velocity \( v \) of the rod after time \( t \) due to gravity is given by: \[ v = g \cdot t \] Where: - \( g = 10 \, \text{m/s}^2 \) is the acceleration due to gravity, - \( t = 2 \, \text{s} \) is the time. Substitute \( v = g \cdot t \) into the emf formula: \[ \text{emf} = B \cdot L \cdot (g \cdot t) \] Substitute the given values: \[ \text{emf} = (3 \times 10^{-5} \, \text{T}) \cdot (1 \, \text{m}) \cdot (10 \, \text{m/s}^2 \cdot 2 \, \text{s}) \] \[ \text{emf} = 6 \times 10^{-4} \, \text{V} \] Thus, the emf induced in the rod at \( t = 2 \, \text{s} \) is \( 6 \times 10^{-4} \, \text{V} \).
Top Questions on Faradays laws of induction
- The magnetic flux \(\phi\) (in weber) linked with a closed circuit of resistance \(8 \, \Omega\) varies with time (in seconds) as \(\phi = 5t^2 - 36t + 1\). The induced current in the circuit at \(t = 2 \, \text{s}\) is ______ A.
- JEE Main - 2024
- Physics
- Faradays laws of induction
- In a coil, the current changes form –2 A to +2A in 0.2 s and induces an emf of 0.1 V. The self-inductance of the coil is :
- JEE Main - 2024
- Physics
- Faradays laws of induction
- A square loop of side 2 cm enters a magnetic field with a constant speed of 2 cm s-1 as shown. The front edge enters the field at t = 0s. Which of the following graph correctly depicts the induced emf in the loop?
( Take clockwise direction positive )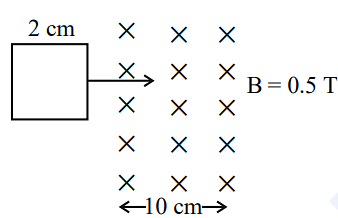
- KCET - 2023
- Physics
- Faradays laws of induction
- The current following through an inductance coil of self inductance 6 mH at different time instants is as shown. The emf induced between t = 20s and t = 40s is nearly
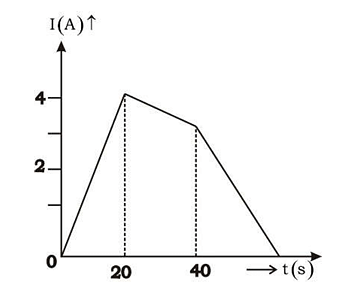
- KCET - 2021
- Physics
- Faradays laws of induction
- A wheel with $20$ metallic spokes each $1\, m$ long is rotated with a speed of $120\, rpm$ in a plane perpendicular to a magnetic field of $0.4\, G$. The induced emf between the axle and rim of the wheel will be, $(1 G = 10^{-4}\, T)$
- NEET (UG) - 2020
- Physics
- Faradays laws of induction
Questions Asked in KCET exam
- If \( A = \{ x : x \text{ is an integer and } x^2 - 9 \geq 0 \} \), \[ B = \{ x : x \text{ is a natural number and } 2 \leq x \leq 5 \}, \quad C = \{ x : x \text{ is a prime number} \leq 4 \} \] Then \( (B - C) \cup A \) is:
- KCET - 2025
- Set Theory
- If the number of terms in the binomial expansion of \((2x + 3)^n\) is 22, then the value of \(n\) is:
- KCET - 2025
- Binomial theorem
- A particle is in uniform circular motion. The equation of its trajectory is given by \( x = 2t^2 - 3t + 5 \), where \( x \) and \( y \) are in meters. The speed of the particle is 2 m/s. When the particle attains the lowest \( y \)-coordinate, the acceleration of the particle is (in \( \text{m/s}^2 \)):
- KCET - 2025
- Friction
- If \( A \) is a square matrix of order \( 3 \times 3 \), \( \det A = 3 \), then the value of \( \det(3A^{-1}) \) is:
- KCET - 2025
- Matrices
- A potential at a point A is 3 V and that at another point B is 5 V. What is the work done in carrying a charge of 5 mC from B to A?
- KCET - 2025
- Electric Field