\(∫\dfrac{x+5}{x^{2}+1}dx=\)
\( 3ln|x-1|-2ln|x+1|+C\)
\(2ln|x-1|-3ln|x+1|+C\)
\(ln|x-2|-ln|x+1|+C\)
\( ln|x+2|+ln|x+1|+C\)
\(2ln|x-1|+3ln|x-1|+C\)
The Correct Option is A
Approach Solution - 1
Given: \(∫\dfrac{x+5}{x^{2}+1}dx=\)
So to solve this question, we can again use partial fraction decomposition.
Step 1:
Factorize the denominator. \((x^2 - 1)\) can be factored as\((x - 1)(x + 1).\)
Step 2:
Partial fraction decomposition. The expression \(\dfrac{x+5}{x^2 - 1}\) can be rewritten as the sum of two fractions with unknown constants \(A\) and \(B\):
\(\dfrac{x+5}{x^2 - 1} = \dfrac{A}{x +1}+\dfrac{B}{x-1}\)
Step 3:
Now to find the values of A and B, we need to find a common denominator, which is \((x- 1)(x + 1)\), and then equate the numerators:
\(x + 5 = A(x+ 1) + B(x- 1)\)
Now, solve for A and B by comparing coefficients: \(A + B = 1\)
(by comparing the coefficients of \(x\)) \(A - B = 5\) ⇢(by comparing the constant terms)
Adding the two equations: \(2A = 6\)
⇒\(A=3\)
Substituting the value of \(A\) one of the equations to find we get
\(B = -2\)
Step 4:
Now we can re-write the parent expression as,
\(∫\dfrac{x + 5}{x^2 - 1} dx = ∫(\dfrac{A}{x - 1}+\dfrac{B}{x+ 1}) dx\)
\(=∫(\dfrac{3}{x-1}) - (\dfrac{2}{x+1})dx\)
\(=∫(\dfrac{3}{x-1}) - (\dfrac{2}{x+1})dx\)
\(=∫(\dfrac{3}{x-1})dx - ∫(\dfrac{2}{x+1})dx\)
\(= 3ln|x-1|-2ln|x+1|+C\) (Ans.)
Approach Solution -2
\(∫\dfrac{x+5}{x^{2}+1}dx\)
So to solve this question, we can use partial fraction decomposition.
Step 1 :- $\int (\frac{3}{x-1} + \frac{-2}{x+1}) dx $
Step 2 :- $\int \frac{3}{x-1} dx+ \int \frac{-2}{x+1} dx $
Step 3 :- $3ln|x-1| + \int \frac{-2}{x+1} dx $
Step 4 :- $3ln|x-1| - 2 ln |x+1| +C$
So, the correct option is (A) : $3ln|x-1| - 2 ln |x+1| +C$
Top Questions on Integral Calculus
- If $$ \alpha = \int_{\frac{1}{2}}^{2} \frac{\tan^{-1} x}{2x^2 - 3x + 2} \, dx, $$ then the value of $ \sqrt{7} \tan \left( \frac{2\alpha \sqrt{7}}{\pi} \right) $ is.
(Here, the inverse trigonometric function $ \tan^{-1} x $ assumes values in $ \left( -\frac{\pi}{2}, \frac{\pi}{2} \right) $.)- JEE Advanced - 2025
- Mathematics
- Integral Calculus
- Maximum value of the function \( f(r) = r^2 e^{-r} \), when \( 0<r<\infty \) is
- IIT JAM CY - 2025
- Mathematics
- Integral Calculus
- The value of the derivative of the function \( y = x e^x \) at \( x = 1 \) is ........... (rounded off to two decimal places).
- IIT JAM EN - 2025
- Mathematics
- Integral Calculus
- The value of the integral \( \int_{3}^{6} \frac{\sqrt{x}}{\sqrt{9 - x} + \sqrt{x}} \, dx \) is:
- WBJEE - 2025
- Mathematics
- Integral Calculus
- If \( x = \int_0^y \frac{1}{\sqrt{1+9t^2}} \, dt \) and \( \frac{d^2y}{dx^2} = ay \), then the value of \( a \) is:
- WBJEE - 2025
- Mathematics
- Integral Calculus
Questions Asked in KEAM exam
- Find the limit: \[ \lim_{x \to 0} \frac{\sin[x]}{[x]}, \text{ where } [x] \text{ represents greatest integer function} \]
- KEAM - 2025
- Continuity
- Benzene when treated with Br\(_2\) in the presence of FeBr\(_3\), gives 1,4-dibromobenzene and 1,2-dibromobenzene. Which type of reaction is this?
- KEAM - 2025
- Haloalkanes and Haloarenes
- Given that \( \vec{a} \parallel \vec{b} \), \( \vec{a} \cdot \vec{b} = \frac{49}{2} \), and \( |\vec{a}| = 7 \), find \( |\vec{b}| \).
- KEAM - 2025
- Vector Algebra
- Evaluate the integral: \[ \int \frac{\sin(2x)}{\sin(x)} \, dx \]
- KEAM - 2025
- integral
- If \( A \) is a \( 3 \times 3 \) matrix and \( |B| = 3|A| \) and \( |A| = 5 \), then find \( \left| \frac{\text{adj} B}{|A|} \right| \).
- KEAM - 2025
- Matrix Operations
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
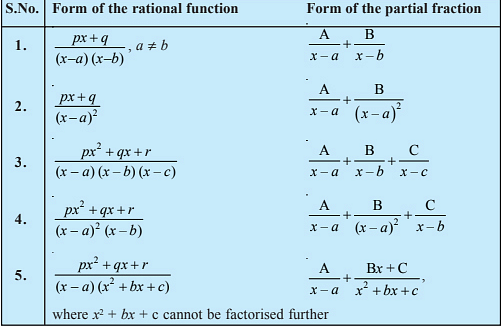
For examples,
