Question:
Integrate the function: \(\frac{(x-3)e^x}{(x-1)^3}\)
Integrate the function: \(\frac{(x-3)e^x}{(x-1)^3}\)
Updated On: Oct 4, 2023
Hide Solution
Verified By Collegedunia
Solution and Explanation
The correct answer is: \(∫e^x[\frac{(x-3)}{(x-1)^2}]dx=\frac{e^x}{(x-1)^2}+C\)
\(∫e^x=[\frac{x-3}{(x-1)^3}]dx=∫e^x[\frac{x-1-2}{(x-1)^3}]dx\)
\(=∫e^x[\frac{1}{(x-1)^2}-\frac{2}{(x-1)^3}]dx\)
Let \(ƒ(x)=\frac{1}{(x-1)^2}\,\,ƒ'(x)=\frac{-2}{(x-1)^3}\)
It is known that,\(∫e^x[ƒ(x)+ƒ'(x)]dx=e^x ƒ(x)+C\)
\(∴∫e^x[\frac{(x-3)}{(x-1)^2}]dx=\frac{e^x}{(x-1)^2}+C\)
\(∫e^x=[\frac{x-3}{(x-1)^3}]dx=∫e^x[\frac{x-1-2}{(x-1)^3}]dx\)
\(=∫e^x[\frac{1}{(x-1)^2}-\frac{2}{(x-1)^3}]dx\)
Let \(ƒ(x)=\frac{1}{(x-1)^2}\,\,ƒ'(x)=\frac{-2}{(x-1)^3}\)
It is known that,\(∫e^x[ƒ(x)+ƒ'(x)]dx=e^x ƒ(x)+C\)
\(∴∫e^x[\frac{(x-3)}{(x-1)^2}]dx=\frac{e^x}{(x-1)^2}+C\)
Was this answer helpful?
0
0
Top Questions on integral
- Let \( y = f(x) \) be a thrice differentiable function in \( (-5, 5) \). Let the tangents to the curve \( y = f(x) \) at \( (1, f(1)) \) and \( (3, f(3)) \) make angles \( \frac{\pi}{6} \) and \( \frac{\pi}{4} \), respectively, with the positive x-axis. If \(2 \int_{\frac{1}{\sqrt{3}}}^{1} \left( \left( f'(t) \right)^2 + 1 \right) f''(t) \, dt = \alpha + \beta \sqrt{3}\) where \( \alpha \), \( \beta \) are integers, then the value of \( \alpha + \beta \) equals
- Let $f : (0, \infty) \to \mathbb{R}$ and $F(x) = \int_{0}^{x} tf(t) \, dt$. If $F(x^2) = x^4 + x^5$, then \[\sum_{r=1}^{12} f(r^2)\]is equal to:
- If \[\int_{0}^{\frac{\pi}{3}} \cos^4 x \, dx = a\pi + b\sqrt{3},\]where $a$ and $b$ are rational numbers, then $9a + 8b$ is equal to:
- The value of \[\int_{0}^{1} \left(2x^3 - 3x^2 - x + 1\right)^{\frac{1}{3}} \, dx\]is equal to:
- Let \( r_k = \frac{\int_{0}^{1} (1 - x^7)^k \, dx}{\int_{0}^{1} (1 - x^7)^{k+1} \, dx}, \, k \in \mathbb{N} \). Then the value of \[ \sum_{k=1}^{10} \frac{1}{7(r_k - 1)}\]is equal to ______.
View More Questions
Questions Asked in CBSE CLASS XII exam
- Find the inverse of each of the matrices, if it exists. \(\begin{bmatrix} 1 & 3\\ 2 & 7\end{bmatrix}\)
- For what values of x,\(\begin{bmatrix} 1 & 2 & 1 \end{bmatrix}\)\(\begin{bmatrix} 1 & 2 & 0\\ 2 & 0 & 1 \\1&0&2 \end{bmatrix}\)\(\begin{bmatrix} 0 \\2\\x\end{bmatrix}\)=O?
- Find the inverse of each of the matrices,if it exists \(\begin{bmatrix} 2 & 1 \\ 7 & 4 \end{bmatrix}\)
What is the Planning Process?
- CBSE CLASS XII - 2023
- Planning process steps
- Find the inverse of each of the matrices,if it exists. \(\begin{bmatrix} 2 & 3\\ 5 & 7 \end{bmatrix}\)
View More Questions
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
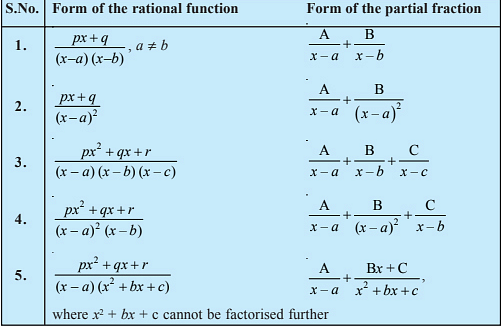
For examples,
