Question:
The variation of stopping potential (V0) as a function of the frequency (v) of the incident light for a metal is shown in figure. The work function of the surface is
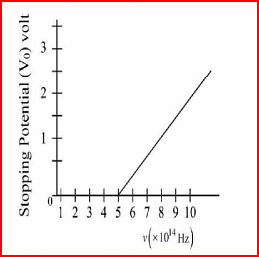
The variation of stopping potential (V0) as a function of the frequency (v) of the incident light for a metal is shown in figure. The work function of the surface is


Updated On: Mar 21, 2025
- 1.36 eV
- 2.98 eV
- 2.07 eV
- 18.6 eV
Hide Solution
Verified By Collegedunia
The Correct Option is C
Solution and Explanation
Step 1: Understanding the photoelectric equation.
In the photoelectric effect, the stopping potential (\( V_0 \)) is related to the frequency (\( v \)) of the incident light by the equation: \[ eV_0 = h(v - v_{\text{th}}) \] Where:
\( V_0 \) is the stopping potential (in volts).
\( v \) is the frequency of the incident light (in Hz).
\( v_{\text{th}} \) is the threshold frequency (below which no photoelectric emission occurs).
\( e \) is the charge of the electron (\( 1.6 \times 10^{-19} \, \text{C} \)).
\( h \) is Planck's constant (\( 6.6 \times 10^{-34} \, \text{J} \cdot \text{s} \)).
Step 2: Identifying the threshold frequency.
From the graph, we can observe that the stopping potential becomes non-zero at a frequency of approximately \( 5 \times 10^{14} \, \text{Hz} \). This is the threshold frequency \( v_{\text{th}} \).
Step 3: Calculating the work function.
At the threshold frequency, the stopping potential is zero. We use the equation: \[ \phi = h v_{\text{th}} \] Substituting the values: \[ \phi = (6.6 \times 10^{-34}) \times (5 \times 10^{14}) = 33 \times 10^{-20} \, \text{J} \] \[ \phi = 3.3 \times 10^{-19} \, \text{J} \] To convert this to eV, divide by the charge of the electron: \[ \phi = \frac{3.3 \times 10^{-19}}{1.6 \times 10^{-19}} \, \text{eV} = 2.07 \, \text{eV} \] Thus, the work function is \( \phi = 2.07 \, \text{eV} \).
In the photoelectric effect, the stopping potential (\( V_0 \)) is related to the frequency (\( v \)) of the incident light by the equation: \[ eV_0 = h(v - v_{\text{th}}) \] Where:
\( V_0 \) is the stopping potential (in volts).
\( v \) is the frequency of the incident light (in Hz).
\( v_{\text{th}} \) is the threshold frequency (below which no photoelectric emission occurs).
\( e \) is the charge of the electron (\( 1.6 \times 10^{-19} \, \text{C} \)).
\( h \) is Planck's constant (\( 6.6 \times 10^{-34} \, \text{J} \cdot \text{s} \)).
Step 2: Identifying the threshold frequency.
From the graph, we can observe that the stopping potential becomes non-zero at a frequency of approximately \( 5 \times 10^{14} \, \text{Hz} \). This is the threshold frequency \( v_{\text{th}} \).
Step 3: Calculating the work function.
At the threshold frequency, the stopping potential is zero. We use the equation: \[ \phi = h v_{\text{th}} \] Substituting the values: \[ \phi = (6.6 \times 10^{-34}) \times (5 \times 10^{14}) = 33 \times 10^{-20} \, \text{J} \] \[ \phi = 3.3 \times 10^{-19} \, \text{J} \] To convert this to eV, divide by the charge of the electron: \[ \phi = \frac{3.3 \times 10^{-19}}{1.6 \times 10^{-19}} \, \text{eV} = 2.07 \, \text{eV} \] Thus, the work function is \( \phi = 2.07 \, \text{eV} \).
Was this answer helpful?
0
1
Top Questions on work, energy and power
- A body of mass 10 kg is moving with a speed of 4 m/s. It is brought to rest by a force in 5 seconds. Calculate the work done by the force.
- MHT CET - 2025
- Physics
- work, energy and power
- A force of 10 N is applied to move a body of mass 5 kg over a distance of 3 meters. Find the work done by the force.
- MHT CET - 2025
- Physics
- work, energy and power
- If a force F = (3i - 2j) N acting on a body displaces it from point (1 m, 2 m) to point (2 m, 0 m), then work done by the force is
- AP EAPCET - 2025
- Physics
- work, energy and power
- Two bodies A and B of masses 1.5 kg and 3 kg are moving with velocities 20 m/s and 15 m/s respectively. If the same retarding force is applied on the two bodies, then the ratio of the distances travelled by the bodies A and B before they come to rest is
- AP EAPCET - 2025
- Physics
- work, energy and power
- If a position dependent force $(3x^2 - 2x + 7)N$ acting on a body of mass 2 kg displaces it from $x = 0\, m$ to $x = 5\, m$, then the work done by the force is
- AP EAPCET - 2025
- Physics
- work, energy and power
View More Questions
Questions Asked in JEE Main exam
- The center of mass of a thin rectangular plate (fig - x) with sides of length \( a \) and \( b \), whose mass per unit area (\( \sigma \)) varies as \( \sigma = \sigma_0 \frac{x}{ab} \) (where \( \sigma_0 \) is a constant), would be

- JEE Main - 2025
- System of Particles & Rotational Motion
Electrolysis of 600 mL aqueous solution of NaCl for 5 min changes the pH of the solution to 12. The current in Amperes used for the given electrolysis is ….. (Nearest integer).
- JEE Main - 2025
- Electrolysis
If the system of equations \[ x + 2y - 3z = 2, \quad 2x + \lambda y + 5z = 5, \quad 14x + 3y + \mu z = 33 \] has infinitely many solutions, then \( \lambda + \mu \) is equal to:}
- JEE Main - 2025
- Calculus
- The value of \( (\sin 70^\circ)(\cot 10^\circ \cot 70^\circ - 1) \) is:
- JEE Main - 2025
- Trigonometric Identities
- Arrange the following in increasing order of solubility product: \[ {Ca(OH)}_2, {AgBr}, {PbS}, {HgS} \]
- JEE Main - 2025
- Solutions
View More Questions