Question:
The value of b > 3 for which \(12∫_3^b \frac{1}{(x^2-1)(x^2-4)}dx=log_e(\frac{40}{40}) \) is equal to.
The value of b > 3 for which \(12∫_3^b \frac{1}{(x^2-1)(x^2-4)}dx=log_e(\frac{40}{40}) \) is equal to.
Updated On: Mar 2, 2024
Hide Solution
Verified By Collegedunia
Correct Answer: 6
Solution and Explanation
The correct answer is: 6
\(l=∫_3^b \frac{1}{(x^2-1)(x^2-4)}dx=\frac{1}{3}(\frac{1}{x^2-4}-\frac{1}{x^2-1})dx\)
\(=ln((\frac{b-2}{b+2})\frac{(b+1)^2}{b-1}^2)-(In\,\frac{4}{5})\)
After simplification ,
\(\frac{49}{40}=\frac{(b-2)}{(b+2)}\frac{(b+1)^2}{(b-1)^2}.\frac{5}{4}\)
⇒ b = 6
Was this answer helpful?
0
0
Top Questions on types of differential equations
- If \( (2,3) \) is the focus and \( x - y + 3 = 0 \) is the directrix of a parabola, then the equation of the tangent drawn at the vertex of the parabola is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If the focus of an ellipse is \((-1,-1)\), equation of its directrix corresponding to this focus is \(x + y + 1 = 0\) and its eccentricity is \(\frac{1}{\sqrt{2}}\), then the length of its major axis is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If \( m_1 \) and \( m_2 \) are the slopes of the direct common tangents drawn to the circles \[ x^2 + y^2 - 2x - 8y + 8 = 0 \quad \text{and} \quad x^2 + y^2 - 8x + 15 = 0 \] then \( m_1 + m_2 \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The equation of the common tangent to the parabola \(y^2 = 8x\) and the circle \(x^2 + y^2 = 2\) is \(ax + by + 2 = 0\). If \(-\frac{a}{b}>0\), then \(3a^2 + 2b + 1 =\)
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The axis of a parabola is parallel to Y-axis. If this parabola passes through the points \( (1,0), (0,2), (-1,-1) \) and its equation is \( ax^2 + bx + cy + d = 0 \), then \( \frac{ad}{bc} \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
View More Questions
Questions Asked in JEE Main exam
- A force \( \mathbf{F} = 2\hat{i} + b\hat{j} + \hat{k} \) is applied on a particle and it undergoes a displacement \( \mathbf{r} = \hat{i} - 2\hat{j} - \hat{k} \). What will be the value of \( b \), if the work done on the particle is zero?
- JEE Main - 2025
- Work and Energy
- A body of mass \(4\) kg is placed at a point \(P\) having coordinates \( (3,4) \) m. Under the action of force \( \mathbf{F} = (2\hat{i} + 3\hat{j}) \) N, it moves to a new point \(Q\) having coordinates \( (6,10) \) m in \(4\) sec. The average power and instantaneous power at the end of \(4\) sec are in the ratio:
- If the equation of the parabola with vertex $\left(\frac{3}{2},\, 3\right)$ and the directrix $x + 2y = 0$ is
$$ ax^2 + by^2 - cxy - 30x - 60y + 225 = 0, $$ then $\alpha + \beta + \gamma$ is equal to:- JEE Main - 2025
- Calculus
- An AC current is represented as: $ i = 5\sqrt{2} + 10 \cos\left(650\pi t + \frac{\pi}{6}\right) \text{ Amp} $ The RMS value of the current is:
- JEE Main - 2025
- AC Circuits
- HA $ (aq) \rightleftharpoons H^+ (aq) + A^- (aq) $ The freezing point depression of a 0.1 m aqueous solution of a monobasic weak acid HA is 0.20 °C. The dissociation constant for the acid is Given: $ K_f(H_2O) = 1.8 \, \text{K kg mol}^{-1} $, molality ≡ molarity
- JEE Main - 2025
- Colligative Properties
View More Questions
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
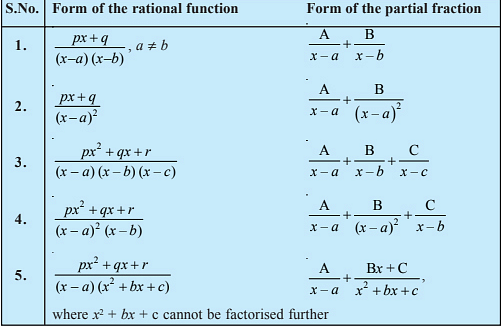
For examples,
