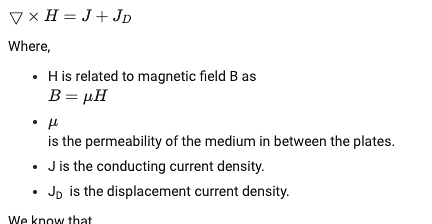
Question:
The rms value of the conduction current in a parallel plate capacitor is 6.9 μA. The capacity of this capacitor, if it is connected to 230 V ac supply with an angular frequency of 600 rad/s, will be :
The rms value of the conduction current in a parallel plate capacitor is 6.9 μA. The capacity of this capacitor, if it is connected to 230 V ac supply with an angular frequency of 600 rad/s, will be :
Updated On: Mar 19, 2025
5 pF
50 pF
100 pF
200 pF
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
Current in capacitor I=\(\frac{V}{X}\)C
I=(V)×(ωC)
C=\(\frac{I}{V\omega}\)=\(\frac{6.9×10^{−6}}{230×600}\)
=50 pF
Was this answer helpful?
0
0
Top Questions on Displacement current
- A current of 2 A flows through a resistor for 10 minutes. What is the total charge that flows through the resistor?
- VITEEE - 2025
- Physics
- Displacement current
- What is the resistance of a conductor if the potential difference across it is 12 V and the current flowing through it is 3 A?
- VITEEE - 2025
- Physics
- Displacement current
- A parallel plate capacitor of area \( A = 16 \, \text{cm}^2 \) and separation between the plates \( 10 \, \text{cm} \), is charged by a DC current. Consider a hypothetical plane surface of area \( A_0 = 3.2 \, \text{cm}^2 \) inside the capacitor and parallel to the plates. At an instant, the current through the circuit is 6A. At the same instant the displacement current through \( A_0 \) is \(\_\_\_\_\_ \)mA.
- JEE Main - 2025
- Physics
- Displacement current
- A parallel plate capacitor of area \( A = 16 \, \text{cm}^2 \) and separation between the plates \( 10 \, \text{cm} \), is charged by a DC current. Consider a hypothetical plane surface of area \( A_0 = 3.2 \, \text{cm}^2 \) inside the capacitor and parallel to the plates. At an instant, the current through the circuit is 6A. At the same instant the displacement current through \( A_0 \) is \_\_\_\_\_ mA.
- JEE Main - 2025
- Physics
- Displacement current
- The variation of charge \( q \) with time \( t \) on a parallel plate capacitor is given by \( q = q_0 \cos(\omega t) \). The displacement current through the capacitor is:
- AP EAPCET - 2023
- Physics
- Displacement current
View More Questions
Questions Asked in JEE Main exam
- If the equation of the parabola with vertex $\left(\frac{3}{2},\, 3\right)$ and the directrix $x + 2y = 0$ is
$$ ax^2 + by^2 - cxy - 30x - 60y + 225 = 0, $$ then $\alpha + \beta + \gamma$ is equal to:- JEE Main - 2025
- Calculus
- In a first order decomposition reaction, the time taken for the decomposition of reactant to one fourth and one eighth of its initial concentration are $ t_1 $ and $ t_2 $ (s), respectively. The ratio $ t_1 / t_2 $ will be:
- JEE Main - 2025
- Chemical Kinetics
- HA $ (aq) \rightleftharpoons H^+ (aq) + A^- (aq) $ The freezing point depression of a 0.1 m aqueous solution of a monobasic weak acid HA is 0.20 °C. The dissociation constant for the acid is Given: $ K_f(H_2O) = 1.8 \, \text{K kg mol}^{-1} $, molality ≡ molarity
- JEE Main - 2025
- Colligative Properties
20 mL of sodium iodide solution gave 4.74 g silver iodide when treated with excess of silver nitrate solution. The molarity of the sodium iodide solution is _____ M. (Nearest Integer value) (Given : Na = 23, I = 127, Ag = 108, N = 14, O = 16 g mol$^{-1}$)
- JEE Main - 2025
- Stoichiometry and Stoichiometric Calculations
- Which of the following binary mixture does not show the behavior of minimum boiling azeotropes?
- JEE Main - 2025
- Solutions
View More Questions
Concepts Used:
Displacement Current
Displacement current is a quantity appearing in Maxwell’s equations. Displacement current definition is defined in terms of the rate of change of the electric displacement field (D). It can be explained by the phenomenon observed in a capacitor.