Question:
A current carrying rectangular loop $P Q R S$ is made of uniform wire The length $P R=Q S=5 \,cm$ and $P Q=R S=100\, cm$ If ammeter current reading changes from $I$ to $2 I$, the ratio of magnetic forces per unit length on the wire $P Q$ due to wire $R S$ in the two cases respectively $\left(f_{P Q}^I: f_{P Q}^{2 I}\right)$ is:
A current carrying rectangular loop $P Q R S$ is made of uniform wire The length $P R=Q S=5 \,cm$ and $P Q=R S=100\, cm$ If ammeter current reading changes from $I$ to $2 I$, the ratio of magnetic forces per unit length on the wire $P Q$ due to wire $R S$ in the two cases respectively $\left(f_{P Q}^I: f_{P Q}^{2 I}\right)$ is:
Updated On: Jul 15, 2024
- $1: 5$
- $1: 3$
- $1: 2$
- $1: 4$
Hide Solution
Verified By Collegedunia
The Correct Option is D
Solution and Explanation
Therefore, The correct answer is option (D): 1:4
Was this answer helpful?
0
0
Top Questions on Displacement current
- A parallel plate capacitor of area \( A = 16 \, \text{cm}^2 \) and separation between the plates \( 10 \, \text{cm} \), is charged by a DC current. Consider a hypothetical plane surface of area \( A_0 = 3.2 \, \text{cm}^2 \) inside the capacitor and parallel to the plates. At an instant, the current through the circuit is 6A. At the same instant the displacement current through \( A_0 \) is \(\_\_\_\_\_ \)mA.
- JEE Main - 2025
- Physics
- Displacement current
- The ratio of average electric energy density and total average energy density of electromagnetic wave is:
- JEE Main - 2023
- Physics
- Displacement current
- The rms value of the conduction current in a parallel plate capacitor is 6.9 μA. The capacity of this capacitor, if it is connected to 230 V ac supply with an angular frequency of 600 rad/s, will be :
- JEE Main - 2022
- Physics
- Displacement current
- Displacement current is caused due to
- UPSEE - 2019
- Physics
- Displacement current
- A plane electromagnetic wave of frequency $20\, MHz$ travels through a space along x- direction. If the electric field vector at a certain point in space is $6\, V$ $m^{-1}$, what is the magnetic field vector at that point ?
- KCET - 2014
- Physics
- Displacement current
View More Questions
Questions Asked in JEE Main exam
- Suppose that the number of terms in an A.P. is \( 2k, k \in \mathbb{N} \). If the sum of all odd terms of the A.P. is 40, the sum of all even terms is 55, and the last term of the A.P. exceeds the first term by 27, then \( k \) is equal to:
- JEE Main - 2025
- Arithmetic Progression
- Two iron solid discs of negligible thickness have radii \( R_1 \) and \( R_2 \) and moment of inertia \( I_1 \) and \( I_2 \), respectively. For \( R_2 = 2R_1 \), the ratio of \( I_1 \) and \( I_2 \) would be \( \frac{1}{x} \), where \( x \) is:
- JEE Main - 2025
- System of Particles & Rotational Motion
- Given below are two statements:
Statement (I): Corrosion is an electrochemical phenomenon in which pure metal acts as an anode and impure metal as a cathode.
Statement (II): The rate of corrosion is more in alkaline medium than in acidic medium.
In the light of the above statements, choose the correct answer from the options given below:- JEE Main - 2025
- Electrochemistry
- The number of solutions of the equation \[ \left( \frac{9}{x} - \frac{9}{\sqrt{x}} + 2 \right) \left( \frac{2}{x} - \frac{7}{\sqrt{x}} + 3 \right) = 0 \] is:
- JEE Main - 2025
- permutations and combinations
- Let \( (2, 3) \) be the largest open interval in which the function \( f(x) = 2 \log_e (x - 2) - x^2 + ax + 1 \) is strictly increasing, and \( (b, c) \) be the largest open interval, in which the function \( g(x) = (x - 1)^3 (x + 2 - a)^2 \) is strictly decreasing. Then \( 100(a + b - c) \) is equal to:
- JEE Main - 2025
- Quadratic Equations
View More Questions
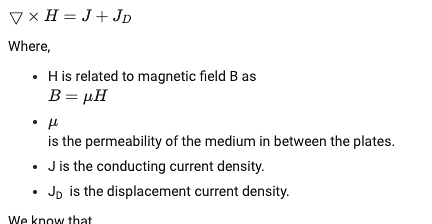
Concepts Used:
Displacement Current
Displacement current is a quantity appearing in Maxwell’s equations. Displacement current definition is defined in terms of the rate of change of the electric displacement field (D). It can be explained by the phenomenon observed in a capacitor.
