Let α, β(α > β) be the roots of the quadratic equation x2 – x – 4 = 0.
If \(P_n=α^n–β^n, n∈N\) then \(\frac{P_{15}P_{16}–P_{14}P_{16}–P_{15}^2+P_{14}P_{15}}{P_{13}P_{14}}\)
is equal to _______.
Let α, β(α > β) be the roots of the quadratic equation x2 – x – 4 = 0.
If \(P_n=α^n–β^n, n∈N\) then \(\frac{P_{15}P_{16}–P_{14}P_{16}–P_{15}^2+P_{14}P_{15}}{P_{13}P_{14}}\)
is equal to _______.
Correct Answer: 16
Solution and Explanation
α, β are the roots of x2 – x – 4 = 0 and
\(P_n=α^n–β^n,\)
\(\therefore I = \frac{(P_{15} - P_{14})P_{16} - P_{15}(P_{15} - P_{14})}{P_{13}P_{14}}\)
\(I = \frac{(P_{16} - P_{15})(P_{15} - P_{14})}{P_{13}P_{14}}\)
\(⇒\)\(I = \frac{(\alpha^{16} - \beta^{16} - \alpha^{15} + \beta^{15})(\alpha^{15} - \beta^{15} - \alpha^{14} + \beta^{14})}{(\alpha^{13} - \beta^{13})(\alpha^{14} - \beta^{14})}\)
\(⇒\)\(I = \frac{\alpha^{15}(\alpha - 1) - \beta^{15}(\beta - 1))(\alpha^{14}(\alpha - 1) - \beta^{14}(\beta - 1))}{(\alpha^{13} - \beta^{13})(\alpha^{14} - \beta^{14})}\)
As \(α^2–α=4\)
\(⇒\)\(α−1=\frac{4}{α}\) and \(β−1=\frac{4}{β}\)
\(⇒\)\(I = \frac{(\alpha^{15} \cdot \frac{4}{\alpha} - \beta^{15} \cdot \frac{4}{\beta})(\alpha^{14} \cdot \frac{4}{\alpha} - \beta^{14} \cdot \frac{4}{\beta})}{(\alpha^{13} - \beta^{13})(\alpha^{14} - \beta^{14})}\)
\(I = \frac{16(\alpha^{14} - \beta^{14})(\alpha^{13} - \beta^{13})}{(\alpha^{14} - \beta^{14})(\alpha^{13} - \beta^{13})}\)
=16
So, the answer is 16.
Top Questions on types of differential equations
- If \( m_1 \) and \( m_2 \) are the slopes of the direct common tangents drawn to the circles \[ x^2 + y^2 - 2x - 8y + 8 = 0 \quad \text{and} \quad x^2 + y^2 - 8x + 15 = 0 \] then \( m_1 + m_2 \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If \( (2,3) \) is the focus and \( x - y + 3 = 0 \) is the directrix of a parabola, then the equation of the tangent drawn at the vertex of the parabola is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- If the focus of an ellipse is \((-1,-1)\), equation of its directrix corresponding to this focus is \(x + y + 1 = 0\) and its eccentricity is \(\frac{1}{\sqrt{2}}\), then the length of its major axis is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The equation of the common tangent to the parabola \(y^2 = 8x\) and the circle \(x^2 + y^2 = 2\) is \(ax + by + 2 = 0\). If \(-\frac{a}{b}>0\), then \(3a^2 + 2b + 1 =\)
- TS EAMCET - 2024
- Mathematics
- types of differential equations
- The axis of a parabola is parallel to Y-axis. If this parabola passes through the points \( (1,0), (0,2), (-1,-1) \) and its equation is \( ax^2 + bx + cy + d = 0 \), then \( \frac{ad}{bc} \) is:
- TS EAMCET - 2024
- Mathematics
- types of differential equations
Questions Asked in JEE Main exam
Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A): The density of the copper ($^{64}Cu$) nucleus is greater than that of the carbon ($^{12}C$) nucleus.
Reason (R): The nucleus of mass number A has a radius proportional to $A^{1/3}$.
In the light of the above statements, choose the most appropriate answer from the options given below:- JEE Main - 2025
- Nuclear physics
- The roots of the quadratic equation \( 3x^2 - px + q = 0 \) are the 10th and 11th terms of an arithmetic progression with common difference \( \frac{3}{2} \). If the sum of the first 11 terms of this arithmetic progression is 88, then \( q - 2q \) is equal to:
- JEE Main - 2025
- Arithmetic Progression and Quadratic Equations
- The type of oxide formed by the element among Li, Na, Be, Mg, B and Al that has the least atomic radius is:
- JEE Main - 2025
- Periodicity of Elements
- The number of real solution(s) of the equation \( x^2 + 3x + 2 = \min \left( |x - 3|, |x + 2| \right) \) is:
- JEE Main - 2025
- Coordinate Geometry
- Two iron solid discs of negligible thickness have radii \( R_1 \) and \( R_2 \) and moment of inertia \( I_1 \) and \( I_2 \), respectively. For \( R_2 = 2R_1 \), the ratio of \( I_1 \) and \( I_2 \) would be \( \frac{1}{x} \), where \( x \) is:
- JEE Main - 2025
- System of Particles & Rotational Motion
Concepts Used:
Types of Differential Equations
There are various types of Differential Equation, such as:
Ordinary Differential Equations:
Ordinary Differential Equations is an equation that indicates the relation of having one independent variable x, and one dependent variable y, along with some of its other derivatives.
\(F(\frac{dy}{dt},y,t) = 0\)
Partial Differential Equations:
A partial differential equation is a type, in which the equation carries many unknown variables with their partial derivatives.
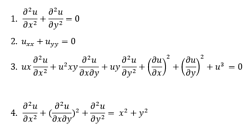
Linear Differential Equations:
It is the linear polynomial equation in which derivatives of different variables exist. Linear Partial Differential Equation derivatives are partial and function is dependent on the variable.
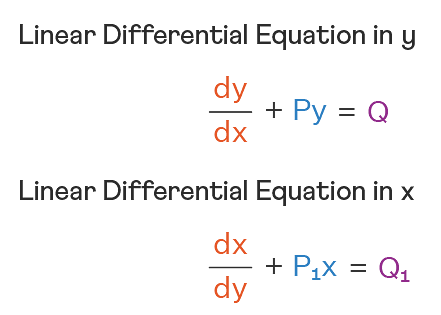
Homogeneous Differential Equations:
When the degree of f(x,y) and g(x,y) is the same, it is known to be a homogeneous differential equation.
\(\frac{dy}{dx} = \frac{a_1x + b_1y + c_1}{a_2x + b_2y + c_2}\)
Read More: Differential Equations