Consider a series of steps as shown. A ball is thrown from 0. Find the minimum speed to directly jump to 5th step
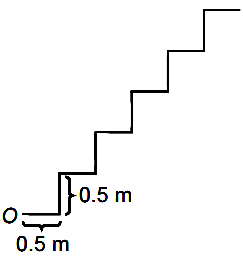
Consider a series of steps as shown. A ball is thrown from 0. Find the minimum speed to directly jump to 5th step
- \(5(\sqrt2+1) m/s\)
- \(5(\sqrt2) m/s\)
- \(5(\sqrt{(\sqrt2+1))} m/s\)
- \(6(\sqrt3+1) m/s\)
The Correct Option is C
Solution and Explanation
The Correct option is (C): \(5(\sqrt{(\sqrt2+1))} m/s\)
\(y=x\tan\theta-\frac{gx^2}{2v^2\cos^2\theta}\)
(2.5,2.5) must lie on this
\(⇒1=\tan\theta-\frac{g\times2.5}{2v^2\cos^2\theta}\)
\(⇒ \frac{25}{2v^2\cos^2\theta}=\tan\theta-1\)
\(⇒ v^2=\frac{25}{2}\left\{\frac{1+\tan^2\theta}{\tan\theta-1}\right\}\)
\(⇒ v_{min}=5\sqrt{\sqrt2+1}\)
[ Happens when \(\tan\theta=\sqrt2+1\) ]
Top Questions on Speed and velocity
- A fluid flows through a pipe with varying cross-section. If the velocity at the narrow section is 3 m/s and the cross-sectional area is half of the wider section, what is the velocity in the wider section?
- BITSAT - 2025
- Physics
- Speed and velocity
- Earth has mass 8 times and radius 2 times that of a planet. If the escape velocity from the earth is 11.2 km/s, the escape velocity in km/s from the planet will be:
- JEE Main - 2025
- Physics
- Speed and velocity
The velocity (v) - time (t) plot of the motion of a body is shown below :

The acceleration (a) - time(t) graph that best suits this motion is :
- NEET (UG) - 2024
- Physics
- Speed and velocity
A wheel of a bullock cart is rolling on a level road, as shown in the figure below. If its linear speed is v in the direction shown, which one of the following options is correct (P and Q are any highest and lowest points on the wheel, respectively) ?

- NEET (UG) - 2024
- Physics
- Speed and velocity
- A particle of mass 2 g and charge 6 $\mu$C is accelerated from rest through a potential difference of 60 V. The speed acquired by the particle is:
- AP EAMCET - 2024
- Physics
- Speed and velocity
Questions Asked in JEE Main exam
- An AC current is represented as: $ i = 5\sqrt{2} + 10 \cos\left(650\pi t + \frac{\pi}{6}\right) \text{ Amp} $ The RMS value of the current is:
- JEE Main - 2025
- AC Circuits
- HA $ (aq) \rightleftharpoons H^+ (aq) + A^- (aq) $ The freezing point depression of a 0.1 m aqueous solution of a monobasic weak acid HA is 0.20 °C. The dissociation constant for the acid is Given: $ K_f(H_2O) = 1.8 \, \text{K kg mol}^{-1} $, molality ≡ molarity
- JEE Main - 2025
- Colligative Properties
Let a line passing through the point $ (4,1,0) $ intersect the line $ L_1: \frac{x - 1}{2} = \frac{y - 2}{3} = \frac{z - 3}{4} $ at the point $ A(\alpha, \beta, \gamma) $ and the line $ L_2: x - 6 = y = -z + 4 $ at the point $ B(a, b, c) $. Then $ \begin{vmatrix} 1 & 0 & 1 \\ \alpha & \beta & \gamma \\ a & b & c \end{vmatrix} \text{ is equal to} $
- JEE Main - 2025
- 3D Geometry
- If the equation of the parabola with vertex $\left(\frac{3}{2},\, 3\right)$ and the directrix $x + 2y = 0$ is
$$ ax^2 + by^2 - cxy - 30x - 60y + 225 = 0, $$ then $\alpha + \beta + \gamma$ is equal to:- JEE Main - 2025
- Calculus
Resonance in X$_2$Y can be represented as

The enthalpy of formation of X$_2$Y is 80 kJ mol$^{-1}$, and the magnitude of resonance energy of X$_2$Y is:- JEE Main - 2025
- Chemical bonding and molecular structure
Concepts Used:
Speed and Velocity
The rate at which an object covers a certain distance is commonly known as speed.
The rate at which an object changes position in a certain direction is called velocity.
Difference Between Speed and Velocity:

Read More: Difference Between Speed and Velocity