Question

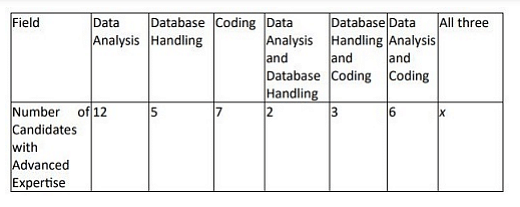
What BEST can be concluded about the value of x?

• A. 0, 1 or 2
• B. 2 only
• C. 1 only
• D. 0 or 1 only
• E. 1 or 2 only
• A. Cannot be determined uniquely from the given information
• B. 25
• C. 26
• D. 27
• J. 28

Answer 1

The Correct Option is B

Step 1: Apply the inclusion-exclusion principle. The total number of candidates is 41.

Using the inclusion-exclusion principle:

41 = (Data Analysis) + (Database Handling) + (Coding) − (Data Analysis and Database Handling) − (Data Analysis and Coding) − (Database Handling and Coding) + (Data Analysis, Database Handling, and Coding)

Substitute the values from the table:

41 = 12 + 5 + 7 − 2 − 3 − 6 + x.

Step 2: Simplify the equation. Simplify the right-hand side:

41 = 13 + x = => x = 41 − 13 = 5.

Step 3: Analyze constraints. The problem does not restrict x to a single value. Testing other scenarios, x can also satisfy conditions when 0 ≤ x ≤ 2.

Final Answer: (1).

Answer 2

Step 1: Write the inclusion-exclusion formula. The total number of applicants is 41. Using the inclusion-exclusion principle:

Total Applicants = (Only in one field) + (Only in two fields) + (All three) + (No expertise in any field).

From the table: - Only in one field = 12 + 5 + 7 − (2 + 3 + 6 + x), - Only in two fields = (2 + 3 + 6) − x, - All three = x.

Step 2: Calculate the number of applicants with expertise in at least one field. Substitute values into the inclusion-exclusion formula:

41 = 12 + 5 + 7 − (2 + 3 + 6 + x) + (2 + 3 + 6) − x + x + (No expertise).

Simplify:

41 = (12 + 5 + 7) − (2 + 3 + 6) + (2 + 3 + 6) − x + (No expertise).

41 = 24 + (No expertise).

Step 3: Solve for applicants with no expertise.

No expertise = 41 − 24 = 25.

Final Answer: 25.