Question:
A bat emitting an ultrasonic wave of frequency \( 4.5 \times 10^4 \, \text{Hz} \) at speed of \( 6 \, \text{m/s} \) between two parallel walls. The two frequencies heard by the bat will be
A bat emitting an ultrasonic wave of frequency \( 4.5 \times 10^4 \, \text{Hz} \) at speed of \( 6 \, \text{m/s} \) between two parallel walls. The two frequencies heard by the bat will be
Show Hint
In Doppler effect problems, remember to adjust the frequency based on whether the source is moving towards or away from the observer.
Updated On: Apr 15, 2025
- \( 4.67 \times 10^4 \, \text{Hz}, 4.34 \times 10^4 \, \text{Hz} \)
- \( 4.34 \times 10^4 \, \text{Hz}, 4.67 \times 10^4 \, \text{Hz} \)
- \( 4.5 \times 10^4 \, \text{Hz}, 5.4 \times 10^4 \, \text{Hz} \)
- \( 4.67 \times 10^3 \, \text{Hz}, 4.34 \times 10^4 \, \text{Hz} \)
Hide Solution
Verified By Collegedunia
The Correct Option is A
Solution and Explanation
The phenomenon described is the Doppler effect, where the frequency of the sound heard by the bat will be altered due to the motion of the bat relative to the walls. - The frequency heard by the bat when the sound is approaching the wall is increased. This is given by: \[ f' = f \left( \frac{v + v_{\text{source}}}{v} \right) \] where: - \( f = 4.5 \times 10^4 \, \text{Hz} \),
- \( v = 6 \, \text{m/s} \) (the speed of sound),
- \( v_{\text{source}} = 6 \, \text{m/s} \) (the speed of the bat). Thus, the frequency heard by the bat when the sound is approaching the wall is: \[ f' = 4.5 \times 10^4 \times \left( \frac{6 + 6}{6} \right) = 4.67 \times 10^4 \, \text{Hz} \] - The frequency heard by the bat when the sound is reflected from the wall is decreased. This is given by: \[ f'' = f \left( \frac{v - v_{\text{source}}}{v} \right) \] Thus: \[ f'' = 4.5 \times 10^4 \times \left( \frac{6 - 6}{6} \right) = 4.34 \times 10^4 \, \text{Hz} \] Therefore, the two frequencies heard by the bat are \( 4.67 \times 10^4 \, \text{Hz} \) and \( 4.34 \times 10^4 \, \text{Hz} \), so the correct answer is (A).
Was this answer helpful?
0
0
Top Questions on doppler effect
- When both the source of sound and observer approach each other with a speed equal to 10% of the speed of sound, then the percentage change in frequency heard by the observer is nearly
- AP EAPCET - 2025
- Physics
- doppler effect
- A passenger is sitting in a fast moving train. The engine of the train blows a whistle of frequency N. If the apparent frequency of sound heard by the passengers is \( N' \), then:
- MHT CET - 2025
- Physics
- doppler effect
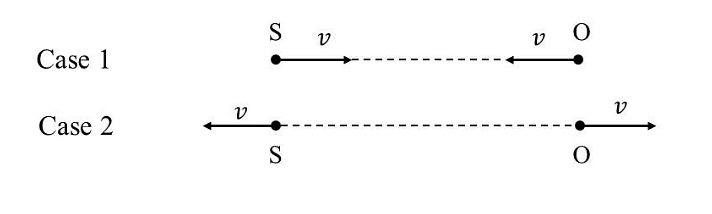
- A source (S) of sound has frequency\( 240 Hz\). When the observer (O) and the source move towards each other at a speed \(v\) with respect to the ground (as shown in Case 1 in the figure), the observer measures the frequency of the sound to be \(288 Hz\). However, when the observer and the source move away from each other at the same speed v with respect to the ground (as shown in Case 2 in the figure), the observer measures the frequency of sound to be \(n\) Hz. The value of \(n\) is _____.
- JEE Advanced - 2024
- Physics
- doppler effect
- A galaxy is moving away from the Earth so that a spectral line at 6000 Å is observed at 6300 Å. Then the speed of the galaxy with respect to the Earth is
- KCET - 2024
- Physics
- doppler effect
- A train is moving with a speed of \(10 m/s\) towards a platform and blows a horn with frequency \(400 Hz\). Find the frequency heard by a passenger standing on the platform. Take speed of sound = \(310 m/s\).
- JEE Main - 2023
- Physics
- doppler effect
View More Questions
Questions Asked in COMEDK UGET exam
- A satellite is revolving around the earth in a circular orbit with kinetic energy of $1.69 \times 10^{10}$ J. The additional kinetic energy required for just escaping into the outer space is
- COMEDK UGET - 2024
- Escape Speed
A solid cylinder of mass 2 kg and radius 0.2 m is rotating about its own axis without friction with angular velocity 5 rad/s. A particle of mass 1 kg moving with a velocity of 5 m/s strikes the cylinder and sticks to it as shown in figure.

The angular velocity of the system after the particle sticks to it will be:- COMEDK UGET - 2024
- The Angular Momentum
- In a given semiconductor, the ratio of the number density of electron to number density of hole is 2 : 1. If $ \frac{1}{7} $th of the total current is due to the hole and the remaining is due to the electrons, the ratio of the drift velocity of holes to the drift velocity of electrons is :
- COMEDK UGET - 2024
- Semiconductors
- A transformer which steps down 330 V to 33 V is to operate a device having impedance 110 $\Omega$. The current drawn by the primary coil of the transformer is:
- COMEDK UGET - 2024
- Transformers
- In the Young's double slit experiment, the 2nd bright fringe for red light coincides with the 3rd bright fringe for violet light. Then the value of 'n' is: (Given: wavelength of red light = 6300 $\text{Å}$ and wavelength of violet light = 4200 $\text{Å}$)
- COMEDK UGET - 2024
- Youngs double slit experiment
View More Questions