Integrate the rational function: \(\frac{x}{(x+1)(x+2)}\)
Solution and Explanation
Let \(\frac{x}{(x+1)(x-+2)} = \frac{A}{(x+1)}+\frac{B}{(x+2)}\)
\(\Rightarrow x = A(x+2)+B(x+1)\)
Equating the coefficients of x and constant term, we obtain
A + B = 1
2A + B = 0
On solving, we obtain
A = −1 and B = 2
∴ \(\frac{x}{(x+1)(x+2)}=\frac{-1}{(x+1)}+\frac{2}{(x+2)}\)
\(\Rightarrow \int \frac{x}{(x+1)(x+2)}dx = \int \frac{-1}{(x+1)}+\frac{2}{(x+2)}dx\)
= \(- \log \mid x+1 \mid +2\log \mid x+2 \mid+C\)
= \(\log(x+2)^2 -\log \mid x+1 \mid+C\)
= \(\log(\frac{x+2)^2}{ (x+1)}+C\)
Top Questions on integral
- Evaluate the integral: \[ \int \frac{\sin(2x)}{\sin(x)} \, dx \]
- Evaluate the integral: \[ \int \sqrt{x^2 + 3x} \, dx \]
Let \( f : (0, \infty) \to \mathbb{R} \) be a twice differentiable function. If for some \( a \neq 0 \), } \[ \int_0^a f(x) \, dx = f(a), \quad f(1) = 1, \quad f(16) = \frac{1}{8}, \quad \text{then } 16 - f^{-1}\left( \frac{1}{16} \right) \text{ is equal to:}\]
- Evaluate the following integral: \[ \int e^x \left[\frac{1}{1+x(1+x)^2}\right] dx \]
- Find the integral: \[ \int \left( \sin^{-1} \sqrt{x} + \cos^{-1} \sqrt{x} \right) \, dx \]
Questions Asked in CBSE CLASS XII exam
- Find: \( \int \frac{5x}{(x + 1)(x^2 + 9)} dx \).
- CBSE CLASS XII - 2025
- Integration
- If A denotes the set of continuous functions and B denotes the set of differentiable functions, then which of the following depicts the correct relation between set A and B?
- CBSE CLASS XII - 2025
- Functions
- f and g are continuous functions on interval \( [0, a] \). Given that \( f(a - x) = f(x) \) and \( g(x) + g(a - x) = a \), show that \( \int_{0}^{a} f(x) g(x) dx = \frac{a}{2} \int_{0}^{a} f(x) dx \).
- CBSE CLASS XII - 2025
- Functions
- Let \( \vec{p} = 2\hat{i} - 3\hat{j} - \hat{k} \), \( \vec{q} = -3\hat{i} + 4\hat{j} + \hat{k} \) and \( \vec{r} = \hat{i} + \hat{j} + 2\hat{k} \). Express \( \vec{r} \) in the form of \( \vec{r} = \lambda \vec{p} + \mu \vec{q} \) and hence find the values of \( \lambda \) and \( \mu \).
- Evaluate: \( \int_{0}^{\pi} \frac{\sin 2px}{\sin x} dx \), \( p \in N \).
- CBSE CLASS XII - 2025
- Integration
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
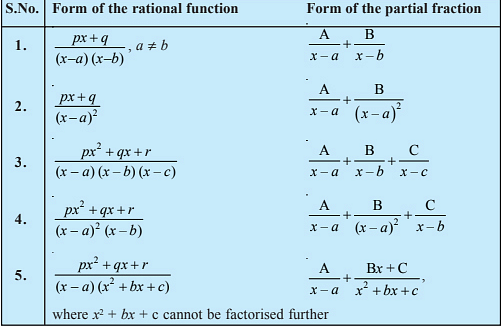
For examples,
