Question:
Integrate the function: \(x(logx)^2\)
Integrate the function: \(x(logx)^2\)
Updated On: Oct 4, 2023
Hide Solution
Verified By Collegedunia
Solution and Explanation
The correct answer is: \(\frac{x^2}{2}(logx)^2-\frac{x^2}{2}logx+\frac{x^2}{4}+C\)
Let \(I=∫x(logx)^2 dx\)
Taking\((logx)^2\) as first function and 1 as second function and integrating by parts,we
obtain
\(I=(logx)^2∫x.dx-∫[{\frac{d}{dx}logx)^2}∫x dx]dx\)
\(=\frac{x^2}{2}(logx)^2-[∫2logx.\frac{1}{x}.\frac{x^2}{2}.dx]\)
\(=\frac{x^2}{2}(logx)^2-∫xlogx. dx\)
Again integrating by parts,we obtain
\(I=\frac{x^2}{2}(logx)^2-[logx∫x dx-∫[{(\frac{d}{dx}logx)∫x dx}]dx]\)
\(=\frac{x^2}{2}(logx)^2-[\frac{x^2}{2}-logx-∫\frac{1}{x}.\frac{x^2}{2}dx]\)
\(=\frac{x^2}{2}(logx)^2-\frac{x^2}{2}logx+\frac{1}{2}∫x dx\)
\(=\frac{x^2}{2}(logx)^2-\frac{x^2}{2}logx+\frac{x^2}{4}+C\)
Let \(I=∫x(logx)^2 dx\)
Taking\((logx)^2\) as first function and 1 as second function and integrating by parts,we
obtain
\(I=(logx)^2∫x.dx-∫[{\frac{d}{dx}logx)^2}∫x dx]dx\)
\(=\frac{x^2}{2}(logx)^2-[∫2logx.\frac{1}{x}.\frac{x^2}{2}.dx]\)
\(=\frac{x^2}{2}(logx)^2-∫xlogx. dx\)
Again integrating by parts,we obtain
\(I=\frac{x^2}{2}(logx)^2-[logx∫x dx-∫[{(\frac{d}{dx}logx)∫x dx}]dx]\)
\(=\frac{x^2}{2}(logx)^2-[\frac{x^2}{2}-logx-∫\frac{1}{x}.\frac{x^2}{2}dx]\)
\(=\frac{x^2}{2}(logx)^2-\frac{x^2}{2}logx+\frac{1}{2}∫x dx\)
\(=\frac{x^2}{2}(logx)^2-\frac{x^2}{2}logx+\frac{x^2}{4}+C\)
Was this answer helpful?
0
0
Top Questions on integral
- Find the value of the integral: \[ \int_0^\pi \sin^2(x) \, dx. \]
- Evaluate the integral: $$ \int_0^{\pi/4} \frac{\ln(1 + \tan x)}{\cos x \sin x} \, dx $$
- Evaluate the integral \( \int_0^1 \frac{\ln(1 + x)}{1 + x^2} \, dx \)
- Let $ f(x) $ be a positive function and $I_1 = \int_{-\frac{1}{2}}^1 2x \, f\left(2x(1-2x)\right) dx$ and $I_2 = \int_{-1}^2 f\left(x(1-x)\right) dx.$ Then the value of $\frac{I_2}{I_1}$ is equal to ____
- Evaluate the integral: $$ \int_0^1 \frac{\ln (1+x)}{1+x^2} \, dx $$
View More Questions
Questions Asked in CBSE CLASS XII exam
If \(\begin{vmatrix} 2x & 3 \\ x & -8 \\ \end{vmatrix} = 0\), then the value of \(x\) is:
- ‘What I want should not be confused.’ What clarification does Pablo Neruda give to his readers? (Keeping Quiet)
- CBSE CLASS XII - 2025
- Literature
- A ladder 13 m long is leaning against the wall. The bottom of the ladder is pulled along the ground away from the wall at the rate of 2 m/s. How fast is the height on the wall decreasing when the foot of the ladder is 12 m away from the wall?
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Application of derivatives
- If $P(A) = \frac{1}{7}$, $P(B) = \frac{5}{7}$ and $P(A \cap B) = \frac{4}{7}$, then $P(A | B)$ is:
- CBSE CLASS XII - 2025
- Probability
- You are Susheel of 10, R.K. Marg, New Delhi. You come across a report stating that a number of people in cities suffer from respiratory diseases, lung cancer, and heart diseases due to air pollution. Write a letter to the editor of a national daily expressing your concern and suggestions and ways to improve the situation.
\includegraphics[width=0.75\linewidth]5b.JPG- CBSE CLASS XII - 2025
- Letter Writing
View More Questions
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
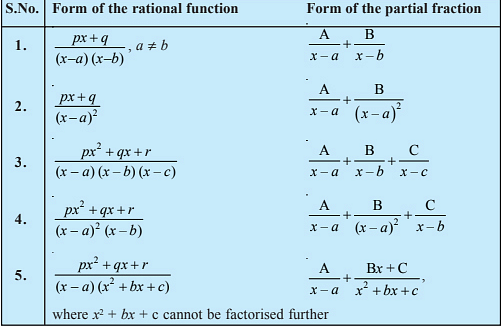
For examples,
