Two solids dissociate as follows
$ {A(s) <=> B(g) + C(g) ; K_{p_1} = x \; atm^2}$
$ {D(s) <=> C(g) + E(g) ; K_{p_2} = y \; atm^2}$
The total pressure when both the solids dissociate simultaneously is :
- $x^2 + y^2 \; atm$
- $ 2 x^2 + y^2 \; atm$
- $2 (\sqrt{x + y} ) atm$
- $\sqrt{x + y} \,atm$
The Correct Option is C
Solution and Explanation
Top Questions on Law Of Chemical Equilibrium And Equilibrium Constant
- Consider the equilibrium: \[ \text{CO(g)} + \text{3H}_2\text{(g)} \rightleftharpoons \text{CH}_4\text{(g)} + \text{H}_2\text{O(g)} \] If the pressure applied over the system increases by two fold at constant temperature then:
- JEE Main - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- For the reaction: \[ A_2(g) \rightleftharpoons B_2(g) \] The equilibrium constant \( K_c \) is given as 99.0. In a 1 L closed flask, two moles of \( B_2(g) \) is heated to T(K). What is the concentration of \( B_2(g) \) (in mol L\(^{-1}\)) at equilibrium?
- AP EAPCET - 2024
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- At equilibrium for the reaction \[ A_2 (g) + B_2 (g) \rightleftharpoons 2AB (g) \] The concentrations of \( A_2 \), \( B_2 \), and \( AB \) respectively are \( 1.5 \times 10^{-3}M \), \( 2.1 \times 10^{-3}M \), and \( 1.4 \times 10^{-3}M \). What will be \( K_p \) for the decomposition of AB at the same temperature?
- AP EAMCET - 2024
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- 10 g of a metal (M) reacts with oxygen to form 11.6 g of oxide. What is the equivalent weight of \( M \)?
- AP EAMCET - 2024
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- The equilibrium constant for the dissociation of HI at 773 K is:
- AP EAPCET - 2024
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
Questions Asked in JEE Main exam
- In YDSE, light of intensity \( 4I \) and \( 9I \) passes through two slits respectively. Difference of maximum and minimum intensity of interference pattern is:
- JEE Main - 2025
- Wave optics
Let \( y = f(x) \) be the solution of the differential equation
\[ \frac{dy}{dx} + 3y \tan^2 x + 3y = \sec^2 x \]
such that \( f(0) = \frac{e^3}{3} + 1 \), then \( f\left( \frac{\pi}{4} \right) \) is equal to:
- JEE Main - 2025
- Differential Equations
Find the IUPAC name of the compound.
- JEE Main - 2025
- Aromaticity & chemistry of aromatic compounds
If \( \lim_{x \to 0} \left( \frac{\tan x}{x} \right)^{\frac{1}{x^2}} = p \), then \( 96 \ln p \) is: 32
- JEE Main - 2025
- Limits and Exponential Functions
- The integral \[ \int_0^\pi \frac{8x}{4\cos^2 x + \sin^2 x} \, dx \text{ is equal to:} \]
- JEE Main - 2025
- Trigonometric Functions
Concepts Used:
Law of Chemical Equilibrium
Law of Chemical Equilibrium states that at a constant temperature, the rate of a chemical reaction is directly proportional to the product of the molar concentrations of the reactants each raised to a power equal to the corresponding stoichiometric coefficients as represented by the balanced chemical equation.
Let us consider a general reversible reaction;
A+B ↔ C+D
After some time, there is a reduction in reactants A and B and an accumulation of the products C and D. As a result, the rate of the forward reaction decreases and that of backward reaction increases.
Eventually, the two reactions occur at the same rate and a state of equilibrium is attained.
By applying the Law of Mass Action;
The rate of forward reaction;
Rf = Kf [A]a [B]b
The rate of backward reaction;
Rb = Kb [C]c [D]d
Where,
[A], [B], [C] and [D] are the concentrations of A, B, C and D at equilibrium respectively.
a, b, c, and d are the stoichiometric coefficients of A, B, C and D respectively.
Kf and Kb are the rate constants of forward and backward reactions.
However, at equilibrium,
Rate of forward reaction = Rate of backward reaction.
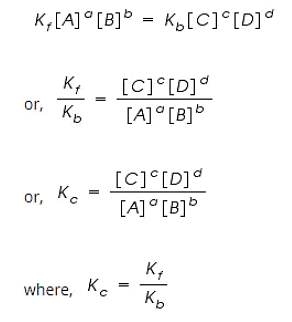
Kc is called the equilibrium constant expressed in terms of molar concentrations.
The above equation is known as the equation of Law of Chemical Equilibrium.