Two coherent monochromatic light beams of intensities ratio 1 : 4 are superposed. The ratio of maximum and minimum intensities in the resulting beam will be
- 9:01
- 5:03
- 25:09:00
- 9:25
The Correct Option is A
Solution and Explanation
As, we know
$I_{max} =\left(\sqrt{I_{1}} +\sqrt{I_{2}}\right)^{2} $
and $I_{min} =\left(\sqrt{I_{1}} -\sqrt{I_{2}}\right)^{2}$
Hence, the ratio
$\frac{I_{max}}{I_{min}} =\left[\frac{\sqrt{I_{1}} +\sqrt{I_{2}}}{\sqrt{I_{1}} -\sqrt{I_{2}}}\right] = \left[\frac{\sqrt{\frac{I_{1}}{I_{2}}}+1}{\sqrt{\frac{I_{1}}{I_{2}}}-1}\right]^{2}$
$\Rightarrow \frac{I_{max}}{I_{min}} = \left[\frac{\sqrt{\frac{1}{4}}+1}{\sqrt{\frac{1}{4}}-1}\right] =\left[\frac{\frac{1+2}{2}}{\frac{1-2}{2}}\right]^{2} = \left[\frac{3}{-1}\right]^{2} = 9 : 1$
$\Rightarrow \frac{I_{max}}{I_{min}} = \frac{9}{1}$
Hence, the ratio of maximum and minimum intensities in the resulting beam is $9 : 1$.
Top Questions on doppler effect
- When both the source of sound and observer approach each other with a speed equal to 10% of the speed of sound, then the percentage change in frequency heard by the observer is nearly
- AP EAPCET - 2025
- Physics
- doppler effect
- A passenger is sitting in a fast moving train. The engine of the train blows a whistle of frequency N. If the apparent frequency of sound heard by the passengers is \( N' \), then:
- MHT CET - 2025
- Physics
- doppler effect
- A source (S) of sound has frequency\( 240 Hz\). When the observer (O) and the source move towards each other at a speed \(v\) with respect to the ground (as shown in Case 1 in the figure), the observer measures the frequency of the sound to be \(288 Hz\). However, when the observer and the source move away from each other at the same speed v with respect to the ground (as shown in Case 2 in the figure), the observer measures the frequency of sound to be \(n\) Hz. The value of \(n\) is _____.
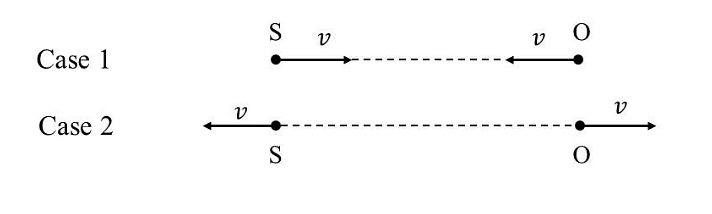
- JEE Advanced - 2024
- Physics
- doppler effect
- A galaxy is moving away from the Earth so that a spectral line at 6000 Å is observed at 6300 Å. Then the speed of the galaxy with respect to the Earth is
- KCET - 2024
- Physics
- doppler effect
- A train is moving with a speed of \(10 m/s\) towards a platform and blows a horn with frequency \(400 Hz\). Find the frequency heard by a passenger standing on the platform. Take speed of sound = \(310 m/s\).
- JEE Main - 2023
- Physics
- doppler effect
Questions Asked in UPSEE exam
Acetic acid dissociates 1.3%. What will be the pH of \(\frac {N}{10}\) solution of the acid.
- UPSEE - 2019
- Acids and Bases
Let z = x + iy be a complex number satisfying the following equation |z - (2 + i)| = |Re(z) - 4 | Which of the following options describes the above equation?
- UPSEE - 2019
- Complex Numbers and Quadratic Equations
- Let x be an $n \times 1$ matrix. Let O and I be the zero, and identity matrices of order n, respectively. Define $P = - \frac{xx^T}{x^Tx}$ is the transpose of x. Then which of the following options is always CORRECT?
- UPSEE - 2019
- Transpose of a Matrix
- Let z = x + iy be a complex number. The equation arg $\left( \frac{z + 1}{z}\right) = \frac{\pi}{4}$ represents
- UPSEE - 2019
- Complex Numbers and Quadratic Equations
- Let $a_1, a_2, a_3$, ... be an arithmetic progression with nonzero common difference. It is given that $\sum^{12}_{i = 4} a_i = 63$ and $a_k = 7 $ for some k . Then the value of k is
- UPSEE - 2019
- Arithmetic Progression
Concepts Used:
Doppler Effect
The Doppler effect is a phenomenon caused by a moving wave source that causes an apparent upward shift in frequency for observers who are approaching the source and a visible downward change in frequency for observers who are retreating from the source. It's crucial to note that the impact isn't caused by a change in the source's frequency.

The Doppler effect may be seen in any wave type, including water waves, sound waves, and light waves. We are most familiar with the Doppler effect because of our encounters with sound waves