The shortest distance between the straight lines through the points $A_1 = (6, 2, 2)$ and
$A_2 = (-4, 0, -1)$, in the directions of $(1, -2, 2)$ and $(3, -2, -2)$ is
- 6
- 8
- 12
- 9
The Correct Option is D
Solution and Explanation
$ \frac{x-6}{1}=\frac{y-2}{2}=\frac{z-2}{2}=k\left(say\right)$
$\therefore x = k + 6, y = -2k + 2, z = 2k + 2$
Hence, general point on the first line,
$P \equiv \left(k + 6, - 2k + 2, 2k + 2\right)$
Equation of second line,
$\frac{x+4}{3}=\frac{y}{-2}=\frac{Z+1}{-2}=l\quad\left(say\right)$
$\therefore x = 3l - 4, y = -2l, z = -2l -1$
Hence, general point on the second line,
$Q \equiv \left(3l - 4, - 2l, -2l - 1\right)$
Direction ratios of PQ are
$3l - 4 - k -6, -2l + 2k -2, - 2l -1 -2k - 2$
i.e. $3l - k - 10, -2l + 2k- 2, - 2l -2k - 3$
Now |PQ| will be the shortest distance
between the two lines if PQ is perpendicular
to both the lines. Hence,
$1\left(3l - k-10\right)+ \left(-2\right)$
$\left(-2l + 2k - 2\right) + 2\left(-2l - 2k - 3\right)= 0$
and $3 \left(3l - k - 10\right) + \left(-2\right)\left(-2l+2k-2\right)+\left(-2\right)\left(-21-2k-3\right)=0$
$i.e. 3l - 9k = 12 or l - 3k = 4\quad\quad\quad ...\left(i\right)$
and $17l - 3k = 20 \quad\quad\quad\quad\quad\quad\quad\quad... \left(ii\right)$
Subtracting equation $\left(i\right)$ from$ \left(ii\right)$, we get
$161 = 16 \quad\quad\quad\quad\therefore l = 1$
Putting this value of l in equation \left(i\right), we get
$- 3k = 3, \therefore k = - 1$
$\therefore P \equiv \left(-1 + 6, - 2 \left(- 1\right) + 2, 2 \left(- 1\right) + 2\right)$
$\quad\quad\equiv \left(5, 4, 0\right)$
Similarly, $Q = \left(-1, -2, -3\right)$
Hence, shortest distance, PQ,
=$\sqrt{\left(-1-5\right)^{2}+\left(-2-4\right)^{2}+\left(-3-0\right)^{2}}$
$=\sqrt{\left(-6\right)^{2}+\left(-6\right)^{2}+\left(-3\right)^{2}}=\sqrt{36+36+9}$
=9 units
Top Questions on Three Dimensional Geometry
Show that the following lines intersect. Also, find their point of intersection:
Line 1: \[ \frac{x - 1}{2} = \frac{y - 2}{3} = \frac{z - 3}{4} \]
Line 2: \[ \frac{x - 4}{5} = \frac{y - 1}{2} = z \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
- Find the value of λ, if the points A(−1,−1,2), B(2,8,λ), C(3,11,6) are collinear.
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
The vector equations of two lines are given as:
Line 1: \[ \vec{r}_1 = \hat{i} + 2\hat{j} - 4\hat{k} + \lambda(4\hat{i} + 6\hat{j} + 12\hat{k}) \]
Line 2: \[ \vec{r}_2 = 3\hat{i} + 3\hat{j} - 5\hat{k} + \mu(6\hat{i} + 9\hat{j} + 18\hat{k}) \]
Determine whether the lines are parallel, intersecting, skew, or coincident. If they are not coincident, find the shortest distance between them.
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
Determine the vector equation of the line that passes through the point \( (1, 2, -3) \) and is perpendicular to both of the following lines:
\[ \frac{x - 8}{3} = \frac{y + 16}{7} = \frac{z - 10}{-16} \quad \text{and} \quad \frac{x - 15}{3} = \frac{y - 29}{-8} = \frac{z - 5}{-5} \]
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Mathematics
- Three Dimensional Geometry
- Find the length of the diagonal of a rectangle with length 6 cm and breadth 8 cm.
- MHT CET - 2025
- Mathematics
- Three Dimensional Geometry
Questions Asked in VITEEE exam
- Find the value of \( x \) in the equation \( 4(x - 2) = 3(x + 5) \).
- VITEEE - 2025
- Linear Equations
- A ball is thrown vertically upwards with a speed of \( 20 \, \text{m/s} \). What is the maximum height reached by the ball? Assume the acceleration due to gravity is \( g = 9.8 \, \text{m/s}^2 \).
- VITEEE - 2025
- Gravitational Potential Energy
- A capacitor is charged with a voltage of 100 V. If the capacitance of the capacitor is \( 10 \, \mu\text{F} \), what is the charge on the capacitor?
- VITEEE - 2025
- Electrostatics
- TUV : VYB :: PRA : ?
- VITEEE - 2025
- Odd one Out
- A ball is dropped from a height of 20 m. What is its velocity just before hitting the ground? (Take \( g = 9.8 \, \text{m/s}^2 \))
- VITEEE - 2025
- Motion in a straight line
Concepts Used:
Three Dimensional Geometry
Mathematically, Geometry is one of the most important topics. The concepts of Geometry are derived w.r.t. the planes. So, Geometry is divided into three major categories based on its dimensions which are one-dimensional geometry, two-dimensional geometry, and three-dimensional geometry.
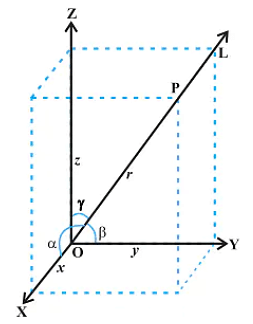
Direction Cosines and Direction Ratios of Line:
Consider a line L that is passing through the three-dimensional plane. Now, x,y and z are the axes of the plane and α,β, and γ are the three angles the line makes with these axes. These are commonly known as the direction angles of the plane. So, appropriately, we can say that cosα, cosβ, and cosγ are the direction cosines of the given line L.