Question:
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.

The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.


Updated On: Nov 30, 2023
Hide Solution
Verified By Collegedunia
Solution and Explanation

It is given that,
Length of the diagonal, d = 24 m
Length of the perpendiculars, h1 and h2, from the opposite vertices to the diagonal are h1 = 8 m and h2 = 13 m
Area of the quadrilateral
\(= \frac{1}{2}(\text{ dh}_1)+ \frac{1}{2}(\text{dh}_2)\)
\(= \frac{1}{2}\) \(\times\)\(\text{d(h}_1+\text{h}_2)\)
\(= \frac{1}{2}\)×24×(13+8)
\(= \frac{1}{2}\)×24×21 = 252
Hence, the required area of the field is 252 m2
Was this answer helpful?
0
0
Top Questions on Area of a Polygon
- Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The area of a trapezium is 34 cm2 and the length of one of the parallel sides is 10 cm and its height is 4 cm. Find the length of the other parallel side.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
- CBSE Class VIII
- Mathematics
- Area of a Polygon
- Diagram of the adjacent picture frame has outer dimensions = 24 cm x 28 cm and inner dimensions 16 cm x 20 cm. Find the area of each section of the frame, if the width of each section is same.
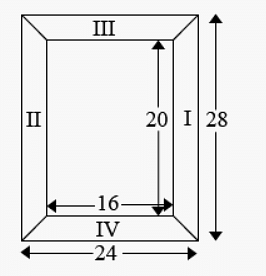
- CBSE Class VIII
- Mathematics
- Area of a Polygon
- There is a pentagonal shaped park as shown in the figure.
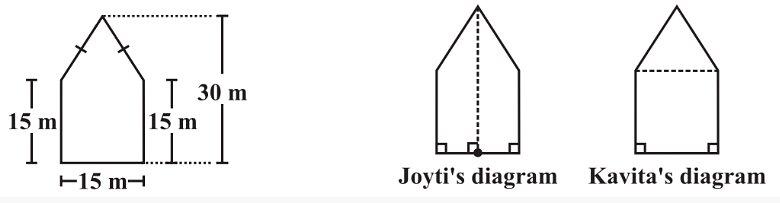
For finding its area Jyoti and Kavita divided it in two different ways. Find the area of this park using both ways. Can you suggest some other way of finding its area?- CBSE Class VIII
- Mathematics
- Area of a Polygon
View More Questions
Questions Asked in CBSE Class VIII exam
- Solve the linear equation: \(\frac{3t-2}{4}-\frac{2t+3}{3}=\frac{2}{3}-t\)
- CBSE Class VIII
- Reducing Equations to Simpler Form
- Add the following.2p2q2 – 3pq + 4, 5 + 7pq – 3p2q.
- CBSE Class VIII
- Addition and Subtraction of Algebraic Expressions
- Factorise the following expressions
- \(7x - 42\)
- \(6p - 12q\)
- \(7a ^2 + 14a\)
- \(-16z + 20z ^3\)
- \(20l^ 2m + 30\, alm\)
- \(5x^ 2 y - 15xy^2\)
- \(10a^ 2 - 15b^ 2 + 20c^ 2 \)
- \(-4a^ 2 + 4ab - 4 ca\)
- \(x ^2 yz + xy^2 z + xyz^2\)
- \(ax^2 y + bxy^2 + cxyz\)
- CBSE Class VIII
- What is Factorisation?
- Draw a pie chart showing the following information. The table shows the colours preferred by a group of people.
Colours Number of people Blue 18 Green 9 Red 6 Yellow 3 Total 36 - CBSE Class VIII
- Circle Graph or Pie Chart
- When he felt the earthquake, do you think Ignesious immediately worried about a tsunami? Give reasons for your answer. Which sentence in the text tells you that the Ignesious family did not have any time to discuss and plan their course of action after the tsunami struck?
- CBSE Class VIII
- The Tsunami
View More Questions