There is a pentagonal shaped park as shown in the figure.
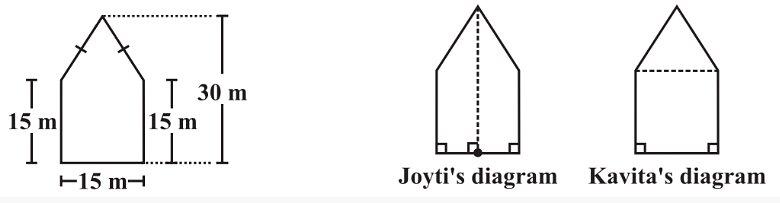
For finding its area Jyoti and Kavita divided it in two different ways. Find the area of this park using both ways. Can you suggest some other way of finding its area?

For finding its area Jyoti and Kavita divided it in two different ways. Find the area of this park using both ways. Can you suggest some other way of finding its area?
Solution and Explanation
(i) Jyoti’s way of finding the area is as follows:
Area of pentagon ABCDE = Area of trapezium ABCF + Area of trapezium AEDF
\(= \frac{1}{2} × (AF + BC) × FC + \frac{1}{2} × (AF + ED) × DF\)
\(= \frac{1}{2} × (30 m + 15 m) × \frac{15}{2} m + \frac{1}{2} × (30 + 15 m) × \frac{15}{2} m\)
\(= 2 × \frac{1}{2} (30 m + 15 m) × \frac{15}{2}\)
\(= 45 m × 7.5 m\)
\(= 337.5 m²\)
Thus, the area of the pentagonal shaped park according to Jyoti’s way is 337.5 m²
(ii) Kavitha’s way of finding the area is as follows:
Area of pentagon ABCDE = Area of triangle ABE + Area of square EBCD
\(= \frac{1}{2} × BE × (AF − OF) + FC × BC \) [Since, AO = AF - OF]
\(= \frac{1}{2} × 15 × (30 − 15) + (15 × 15 )\)
\(= (\frac{1}{2} × 15 × 15) m² + 225 m²\)
\(= 112.5 m² + 225 m²\)
\(= 337.5 m²\)
Thus, the area of the pentagonal shaped park according to Kavitha’s way is 337.5 m²
Top Questions on Area of a Polygon
- Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The area of a trapezium is 34 cm2 and the length of one of the parallel sides is 10 cm and its height is 4 cm. Find the length of the other parallel side.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
- CBSE Class VIII
- Mathematics
- Area of a Polygon
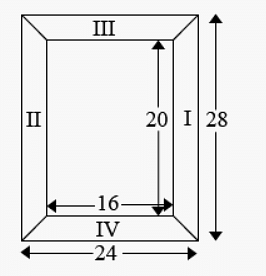
- Diagram of the adjacent picture frame has outer dimensions = 24 cm x 28 cm and inner dimensions 16 cm x 20 cm. Find the area of each section of the frame, if the width of each section is same.
- CBSE Class VIII
- Mathematics
- Area of a Polygon
- Mohan wants to buy a trapezium shaped field. Its side along the river is parallel to and twice the side along the road. It the area of this field is 10500 m2 and the perpendicular distance between the two parallel sides is 100 m, find the length of the side along the river.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
Questions Asked in CBSE Class VIII exam
- Find the number of digits in the square root of each of the following numbers (without any calculation).
- 64
- 144
- 4489
- 27225
- 390625
- CBSE Class VIII
- Square Roots of Decimals
- Fill in the blanks with suitable words.
- Time taken by an object to complete one oscillation is called ___ .
- Loudness is determined by the ___ of vibration.
- The unit of frequency is ___.
- Unwanted sound is called __________ .
- Shrillness of a sound is determined by the ___ of vibration.
- CBSE Class VIII
- Sound Produced by Humans
- Subtract 4a – 7ab + 3b + 12 from 12a – 9ab + 5b – 3
- CBSE Class VIII
- Addition and Subtraction of Algebraic Expressions
- Add the following. a – b + ab, b – c + bc, c – a + ac.
- CBSE Class VIII
- Addition and Subtraction of Algebraic Expressions
- Subtract 4p2q – 3pq + 5pq2 – 8p + 7q – 10 from 18 – 3p – 11q + 5pq – 2pq2 + 5p2q
- CBSE Class VIII
- Addition and Subtraction of Algebraic Expressions