Question:
The coordinates of a moving particle at any time t are given by $ x=\alpha {{t}^{3}} $ and $ y={{t}^{3}}. $ The speed of the particle at time t is given by
The coordinates of a moving particle at any time t are given by $ x=\alpha {{t}^{3}} $ and $ y={{t}^{3}}. $ The speed of the particle at time t is given by
Updated On: Jul 29, 2022
- $ 3t\sqrt{{{\alpha }^{2}}+{{\beta }^{2}}} $
- $ 3{{t}^{2}}\sqrt{{{\alpha }^{2}}+{{\beta }^{2}}} $
- $ {{t}^{2}}\sqrt{{{\alpha }^{2}}+{{\beta }^{2}}} $
- $ \sqrt{{{\alpha }^{2}}+{{\beta }^{2}}} $
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
$ x=\alpha \,\,{{t}^{3}},\,\,y=\beta {{t}^{3}} $ $ {{v}_{x}}=\frac{dx}{dt}=3\alpha {{t}^{2}} $ $ {{v}_{y}}=\frac{dy}{dt}=3\beta {{t}^{2}} $ Resultant velocity, $ v=\sqrt{{{v}_{x}}^{2}+{{v}_{y}}^{2}} $ $ =\sqrt{9{{\alpha }^{2}}{{t}^{4}}+9{{\beta }^{2}}{{t}^{4}}} $ $ =3{{t}^{2}}\sqrt{{{\alpha }^{2}}+{{\beta }^{2}}} $
Was this answer helpful?
0
0
Top Questions on kinetics equations
Find the time required to complete a reaction 90% if the reaction is completed 50% in 15 minutes.
- MHT CET - 2024
- Chemistry
- kinetics equations
- Magnetic Moment of \( \text{Mn}^{2+} \) is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- IUPAC Name of Glyceraldehyde is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- IUPAC Name of Acetone is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- The half-life period of a first order reaction is 1000 seconds. Its rate constant is:
- KEAM - 2024
- Chemistry
- kinetics equations
View More Questions
Questions Asked in Rajasthan PMT exam
- 300 cal of heat is supplied to raise the temperature of 50 g of air from $ 20{}^\circ C $ to $ 30{}^\circ C $ without any change in its volume. Change in internal energy per gram of air is
- Rajasthan PMT - 2011
- internal energy
- The velocity of water flowing in a non-uniform tube is 20 cm/s at a point where the tube radius is 0.2 cm. The velocity at another point, where the radius is 0.1 cm, is
- Rajasthan PMT - 2011
- mechanical properties of fluid
- Block A of mass 4 kg and block B of mass 6 kg are resting on a horizontal surface as shown in the figure. There is no friction between the block B and the horizontal surface. The coefficient of friction between the blocks is 0.2. If the value of $ g=10\,m/{{s}^{2}}, $ the maximum horizontal force F that can be applied on block B without any relative motion between A and B is
- The excess pressure inside a soap bubble A is twice that in another soap bubble B. The ratio of volumes of A and B is
- Rajasthan PMT - 2011
- Surface Tension
- The unit of surface tension is
- Rajasthan PMT - 2011
- Surface Tension
View More Questions
Concepts Used:
Kinetics Equations
It is branch of physics that defines motion with respect to space and time is known as kinematics.
Inverse Kinematics: Inverse Kinematics do the reverse of kinematics.
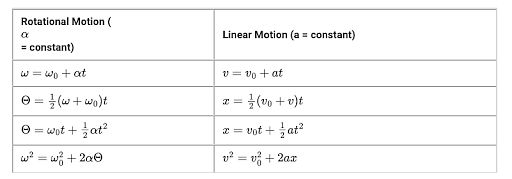
There are four basic kinematics equations:
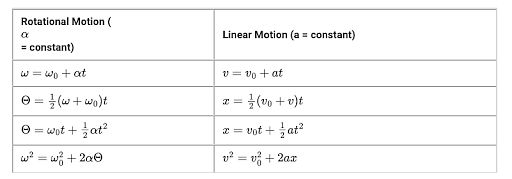
Rotational Kinematics Equations
Another branch of kinematics equations which deals with the rotational motion of anybody.