The angle of minimum deviation for a prism of apex angle 60° and refractive index of \(\sqrt{2}\) is:
The angle of minimum deviation for a prism of apex angle 60° and refractive index of \(\sqrt{2}\) is:
45°
90°
30°
60°
15°
The Correct Option is C
Approach Solution - 1
Given:
- Apex angle of prism, \( A = 60^\circ \)
- Refractive index, \( \mu = \sqrt{2} \)
Step 1: Formula for Minimum Deviation (\( \delta_{\text{min}} \))
The angle of minimum deviation is given by:
\[ \mu = \frac{\sin\left(\frac{A + \delta_{\text{min}}}{2}\right)}{\sin\left(\frac{A}{2}\right)} \]
Step 2: Substitute Known Values
\[ \sqrt{2} = \frac{\sin\left(\frac{60^\circ + \delta_{\text{min}}}{2}\right)}{\sin\left(30^\circ\right)} \]
Since \( \sin 30^\circ = 0.5 \):
\[ \sqrt{2} = \frac{\sin\left(30^\circ + \frac{\delta_{\text{min}}}{2}\right)}{0.5} \]
\[ \sin\left(30^\circ + \frac{\delta_{\text{min}}}{2}\right) = \frac{\sqrt{2}}{2} = \sin 45^\circ \]
Step 3: Solve for \( \delta_{\text{min}} \)
\[ 30^\circ + \frac{\delta_{\text{min}}}{2} = 45^\circ \]
\[ \frac{\delta_{\text{min}}}{2} = 15^\circ \]
\[ \delta_{\text{min}} = 30^\circ \]
Conclusion:
The angle of minimum deviation is \( 30^\circ \).
Answer: \(\boxed{C}\)
Approach Solution -2
1. Define variables and given information:
- A = 60° (apex angle of the prism)
- n = √2 (refractive index)
- δm = ? (angle of minimum deviation)
2. Recall the formula for the angle of minimum deviation:
The formula relating the angle of minimum deviation (δm), apex angle (A), and refractive index (n) is:
\[n = \frac{\sin(\frac{A + \delta_m}{2})}{\sin(\frac{A}{2})}\]
3. Substitute the given values and solve for δm:
\[\sqrt{2} = \frac{\sin(\frac{60^\circ + \delta_m}{2})}{\sin(\frac{60^\circ}{2})}\]
\[\sqrt{2} = \frac{\sin(\frac{60^\circ + \delta_m}{2})}{\sin(30^\circ)}\]
Since \(\sin(30^\circ) = \frac{1}{2}\):
\[\sqrt{2} = 2\sin(\frac{60^\circ + \delta_m}{2})\]
\[\frac{\sqrt{2}}{2} = \sin(\frac{60^\circ + \delta_m}{2})\]
\[\frac{1}{\sqrt{2}} = \sin(\frac{60^\circ + \delta_m}{2})\]
Since \(\sin(45^\circ) = \frac{1}{\sqrt{2}}\):
\[45^\circ = \frac{60^\circ + \delta_m}{2}\]
\[90^\circ = 60^\circ + \delta_m\]
\[\delta_m = 90^\circ - 60^\circ = 30^\circ\]
Top Questions on Ray optics and optical instruments
- Explain the transmission of optical signal through an optical fiber with a diagram.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- With the help of a ray diagram, show that a straw appears bent when it is partly dipped in water and explain it.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- When a parallel beam of light enters water surface obliquely at some angle, what is the effect on the width of the beam?
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- Two convex lenses A and B, each of focal length 10.0 cm, are mounted on an optical bench at 50.0 cm and 70.0 cm respectively. An object is mounted at 20.0 cm. Find the nature and position of the final image formed by the combination.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- The focal length of a concave mirror in air is \( f \). When the mirror is immersed in a liquid of refractive index \( \frac{3}{5} \), its focal length will become:
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
Questions Asked in KEAM exam
- The element that has the highest melting point in the 3d series is:
- KEAM - 2025
- d -and f -Block Elements
- 10 g of (90 percent pure) \( \text{CaCO}_3 \), treated with excess of HCl, gives what mass of \( \text{CO}_2 \)?
- KEAM - 2025
- Stoichiometry and Stoichiometric Calculations
- If \( 5 \sin^{-1} \alpha + 3 \cos^{-1} \alpha = \pi \), then \( \alpha = ? \)
- KEAM - 2025
- Inverse Trigonometric Functions
- If \( -5<x \leq -1 \), then \( -21 \leq 5x + 4 \leq b \). Find \( b \).
- KEAM - 2025
- Algebra
- Benzene when treated with Br\(_2\) in the presence of FeBr\(_3\), gives 1,4-dibromobenzene and 1,2-dibromobenzene. Which type of reaction is this?
- KEAM - 2025
- Haloalkanes and Haloarenes
Concepts Used:
Prism
A homogeneous solid transparent and refracting medium bounded by 2 plane surfaces inclined at an angle called prism. The prism has two triangular faces that are parallel to each other and 3 rectangular surfaces. They are made from glass or other transparent material cut with accurate angles. Out of three, two rectangular faces are polished and are called refracting surfaces. The angle between these two rectangular faces is known as the refractive angle of the prism.
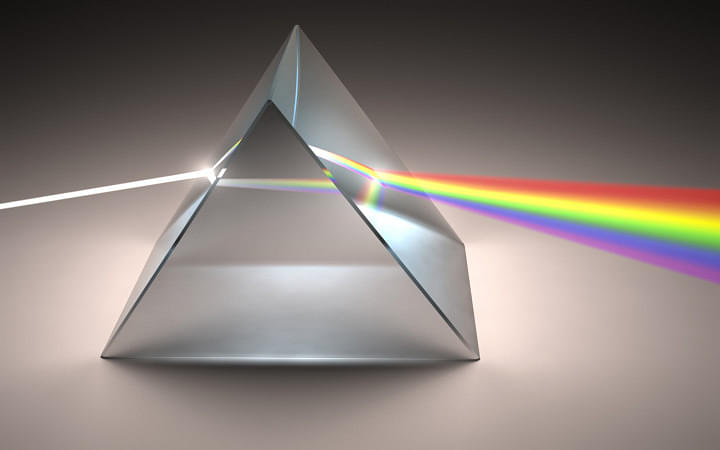
Depending on the application, prisms are made in different shapes. Triangle is the traditional shape of the prism. The triangular prism, when passed through the prism, separates the white light into constituent colors.
Five types of Prism are:
- Dispersive Prism
- Reflective Prism
- Polarizing Prism
- Beam-Splitting Prism
- Deflecting Prism