Let \(\alpha\ and\ \beta\) be real numbers such that \(-\frac{\pi}{4}<\beta<0<\alpha<\frac{\pi}{4}\). If \(\sin (\alpha+\beta)=\frac{1}{3}\ and\ \cos (\alpha-\beta)=\frac{2}{3}\), then the greatest integer less than or equal to
\(\left(\frac{\sin \alpha}{\cos \beta}+\frac{\cos \beta}{\sin \alpha}+\frac{\cos \alpha}{\sin \beta}+\frac{\sin \beta}{\cos \alpha}\right)^2\) is ____
Let \(\alpha\ and\ \beta\) be real numbers such that \(-\frac{\pi}{4}<\beta<0<\alpha<\frac{\pi}{4}\). If \(\sin (\alpha+\beta)=\frac{1}{3}\ and\ \cos (\alpha-\beta)=\frac{2}{3}\), then the greatest integer less than or equal to
\(\left(\frac{\sin \alpha}{\cos \beta}+\frac{\cos \beta}{\sin \alpha}+\frac{\cos \alpha}{\sin \beta}+\frac{\sin \beta}{\cos \alpha}\right)^2\) is ____
Correct Answer: 1
Solution and Explanation
\(\sin(\alpha + \beta) = \frac{1}{3}\) and \(\cos(\alpha - \beta) = \frac{2}{3}\)
The expression to simplify is:
\(\left(\frac{\sin \alpha}{\cos \beta} + \frac{\cos \beta}{\sin \alpha} + \frac{\cos \alpha}{\sin \beta} + \frac{\sin \beta}{\cos \alpha}\right)^2\)
This expression is equal to:
\(\left(\frac{\cos(\alpha - \beta)}{\sin \beta \cos \beta} + \frac{\cos(\alpha - \beta)}{\sin \alpha \cos \alpha}\right)^2\)
This can be simplified further to:
\(= \left(\frac{4}{3} \left\{\frac{1}{\sin 2\beta} + \frac{1}{\sin 2\alpha}\right\}\right)^2\)
Next, simplifying more:
\(= \frac{16}{9}\left(\frac{2 \sin(\alpha + \beta) \cos(\alpha - \beta)}{\sin 2\alpha \sin 2\beta}\right)^2\)
This becomes:
\(= \frac{16}{9} \left(\frac{4 \times \frac{1}{2} \times \frac{2}{3}}{\cos(2\alpha - 2\beta) - \cos(2\alpha + 2\beta)}\right)^2\)
We simplify the denominator further:
\(= \frac{16}{9} \left(\frac{\frac{8}{9}}{2 \cos^2(\alpha - \beta) - 1 - 1 + 2 \sin^2(\alpha + \beta)}\right)^2\)
This simplifies to:
\(= \frac{16}{9} \left(\frac{\frac{8}{9}}{\frac{8}{9} + 2 + \frac{2}{9}}\right)\)
Finally, we get:
\(= \frac{16}{9} = 1\)
Therefore, the correct answer is 1.
Top Questions on Some Applications of Trigonometry
- A ladder 7 m long makes an angle of 30\(^\circ\) with the wall. Find the height of the point on the wall where the ladder touches the wall.
- Bihar Class X Board - 2025
- Mathematics
- Some Applications of Trigonometry
- A kite is at a height of 30 m from the earth and its string makes an angle 60\(^\circ\) with the earth. Then the length of the string is
- Bihar Class X Board - 2025
- Mathematics
- Some Applications of Trigonometry
- The angle of elevation of the top of a tower at a distance of 10 m from its base is 60\(^\circ\); then the height of the tower is
- Bihar Class X Board - 2025
- Mathematics
- Some Applications of Trigonometry
- The shadow of a tower, when the angle of elevation of the sun is $30^\circ$, is $50$ m longer than when the angle of elevation was $60^\circ$ on the plane ground. Find the height of the tower.
- UP Board X - 2025
- Mathematics
- Some Applications of Trigonometry
- Let $$ \alpha = \frac{1}{\sin 60^\circ \sin 61^\circ} + \frac{1}{\sin 62^\circ \sin 63^\circ} + \cdots + \frac{1}{\sin 118^\circ \sin 119^\circ}. $$ Then the value of $$ \left( \frac{\csc 1^\circ}{\alpha} \right)^2 $$ is \rule{1cm}{0.15mm}.
- JEE Advanced - 2025
- Mathematics
- Some Applications of Trigonometry
Questions Asked in JEE Advanced exam
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
A temperature difference can generate e.m.f. in some materials. Let $ S $ be the e.m.f. produced per unit temperature difference between the ends of a wire, $ \sigma $ the electrical conductivity and $ \kappa $ the thermal conductivity of the material of the wire. Taking $ M, L, T, I $ and $ K $ as dimensions of mass, length, time, current and temperature, respectively, the dimensional formula of the quantity $ Z = \frac{S^2 \sigma}{\kappa} $ is:
- JEE Advanced - 2025
- Dimensional Analysis
Let $ y(x) $ be the solution of the differential equation $$ x^2 \frac{dy}{dx} + xy = x^2 + y^2, \quad x > \frac{1}{e}, $$ satisfying $ y(1) = 0 $. Then the value of $ 2 \cdot \frac{(y(e))^2}{y(e^2)} $ is ________.
- JEE Advanced - 2025
- Differential equations
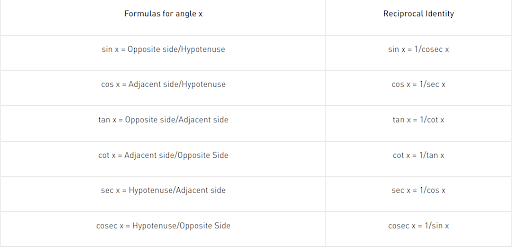
Concepts Used:
Trigonometric Functions
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions: