In a lactic acid solution at pH 4.8, the concentrations of lactic acid and lactate are 0.01 M and 0.087 M, respectively. The calculated pKa of lactic acid is _______. (Round off to one decimal place)
Show Hint
Solution and Explanation
Given:
- \(\text{pH} = 4.8\)
- \([\text{HA}] = 0.01 \, M\) (concentration of lactic acid)
- \([\text{A}^-] = 0.087 \, M\) (concentration of lactate)
Rounding off to one decimal place, the \( \text{p}K_a \) of lactic acid is:
\[ \text{p}K_a = 3.9 \] Conclusion:This calculation demonstrates how the dissociation constant for lactic acid can be deduced from the equilibrium concentrations of the acid and its conjugate base at a specific pH.
Top Questions on Nucleic acid: replication, transcription, splicing, translation
- Calculate the λmax of the following molecule:
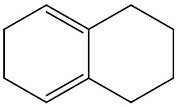
- GPAT - 2024
- Biochemistry
- Nucleic acid: replication, transcription, splicing, translation
- Calculate the \( \lambda_{\text{max}} \) of the following molecule:
- GPAT - 2024
- Biochemistry
- Nucleic acid: replication, transcription, splicing, translation
- DNA and RNA contain the following two major purine bases:
- GPAT - 2023
- Biochemistry
- Nucleic acid: replication, transcription, splicing, translation
- Given below are two statements
Statement I: Sequencing of DNA is much easier than RNA sequencing due to greater stability
Statement II: The chemical method of DNA sequencing (Maxam & Gilbert) works for only single stranded DNA
In light of the above statements, choose the correct answer from the options given below- GPAT - 2022
- Biochemistry
- Nucleic acid: replication, transcription, splicing, translation
- RNA molecules having intrinsic catalytic activity are called as___
- GPAT - 2019
- Biochemistry
- Nucleic acid: replication, transcription, splicing, translation
Questions Asked in GATE XL exam
An electricity utility company charges ₹7 per kWh. If a 40-watt desk light is left on for 10 hours each night for 180 days, what would be the cost of energy consumption? If the desk light is on for 2 more hours each night for the 180 days, what would be the percentage-increase in the cost of energy consumption?
The \( F_{121} \) value of a known microorganism with \( Z \) value of \( 11^\circ C \) is 2.4 min for 99.9999% inactivation. For a 12D inactivation of the said microorganism at \( 143^\circ C \), the \( F \) value (in min) is .......... (rounded off to 3 decimal places)
- GATE XL - 2025
- Food Technology
- Consider a five-digit number PQRST that has distinct digits P, Q, R, S, and T, and satisfies the following conditions:
1. \( P<Q \)
2. \( S>P>T \)
3. \( R<T \)
If integers 1 through 5 are used to construct such a number, the value of P is:- GATE ST - 2025
- GATE CS - 2025
- GATE MN - 2025
- GATE XE - 2025
- GATE XL - 2025
- mathematical reasoning
Three villages P, Q, and R are located in such a way that the distance PQ = 13 km, QR = 14 km, and RP = 15 km, as shown in the figure. A straight road joins Q and R. It is proposed to connect P to this road QR by constructing another road. What is the minimum possible length (in km) of this connecting road?
Note: The figure shown is representative.
- A business person buys potatoes of two different varieties P and Q, mixes them in a certain ratio and sells them at ₹192 per kg.
The cost of the variety P is ₹800 for 5 kg.
The cost of the variety Q is ₹800 for 4 kg.
If the person gets 8% profit, what is the P : Q ratio (by weight)?