Question:
If a substance with half-life $3$ days is taken at other place in $12$ days, what amount of substance is left now ?
If a substance with half-life $3$ days is taken at other place in $12$ days, what amount of substance is left now ?
Updated On: Jul 2, 2022
- 44565
- 44569
- 44577
- Jan-32
Hide Solution
Verified By Collegedunia
The Correct Option is C
Solution and Explanation
The time in which mass of a radioactive substance remains half of its initial mass is known as its half life $\left(t_{1 / 2}\right)$.
$t_{1 / 2}=\frac{0.693}{\lambda}$ (disintegration constant)
Half-life is independent of temperature, pressure and number of atoms present initially.
$T_{1 / 2}$ of a non radioactive substance is infinity
Half-life $t_{1 / 2}=3$ days
Total time $=12$ days
$N=N_{0}\left(\frac{1}{2}\right)^{n}$ where
$N_{0}=$ Initial amount
$N=$ Amount left after disintegration
$n=\frac{\text { Total time }}{\text { Half-life }}$
$n=$ number of half life
$=\frac{12}{3} =4$
$N =\left(\frac{1}{2}\right)^{4}$
$=\frac{1}{16}$
Was this answer helpful?
0
0
Top Questions on kinetics equations
Find the time required to complete a reaction 90% if the reaction is completed 50% in 15 minutes.
- MHT CET - 2024
- Chemistry
- kinetics equations
- Magnetic Moment of \( \text{Mn}^{2+} \) is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- IUPAC Name of Glyceraldehyde is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- IUPAC Name of Acetone is:
- MHT CET - 2024
- Chemistry
- kinetics equations
- The half-life period of a first order reaction is 1000 seconds. Its rate constant is:
- KEAM - 2024
- Chemistry
- kinetics equations
View More Questions
Questions Asked in AFMC exam
- 1200 ml of air remaining in lungs even after forceful expiration is
- AFMC - 2012
- Exchange Of Gases
- A dicot plant in which scattered vascular bundles are present in stem is
- AFMC - 2012
- Anatomy of Flowering Plants
- Which plays an important role in the dispersal of spores in Funaria?
- AFMC - 2012
- Plant Kingdom
- Sexual reproduction in eubacteria takes place by
- AFMC - 2012
- Kingdom Monera
- Which of the following acid is also a vitamin?
- AFMC - 2012
- Digestion and absorption
View More Questions
Concepts Used:
Kinetics Equations
It is branch of physics that defines motion with respect to space and time is known as kinematics.
Inverse Kinematics: Inverse Kinematics do the reverse of kinematics.
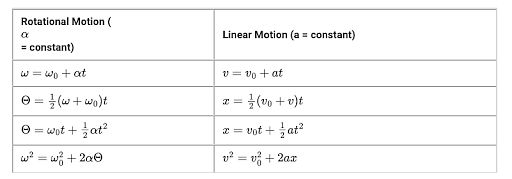
There are four basic kinematics equations:
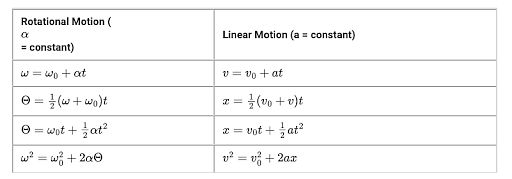
Rotational Kinematics Equations
Another branch of kinematics equations which deals with the rotational motion of anybody.