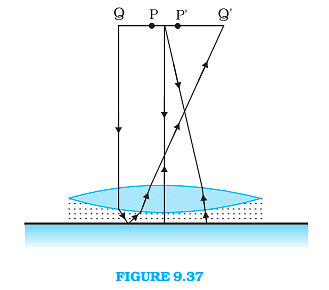
Figure 9.37 shows an equiconvex lens (of refractive index 1.50) in contact with a liquid layer on top of a plane mirror. A small needle with its tip on the principal axis is moved along the axis until its inverted image is found at the position of the needle.

Solution and Explanation
STEP 1:-
In order to find the focal length of the concave lens of liquid:
The formula used will be: \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}\)
In the absence of the liquid, for the combination of the convex lens and plane mirror, the inverted image coincides with the object only when the object is placed at the focus of the convex lens. The same principle applies when the liquid is introduced between the lens and the mirror. Therefore, the given object or image distance can be considered as the focal length of the respective combinations.
When the liquid is present, the system acts as a combination of a convex lens and a concave lens. Hence, the focal length of the combination is denoted as f (let's say): f = 45 cm
When the liquid is removed, the image is formed at 30 cm.
Therefore, the focal length of the convex lens (f1) is: f1 = 30 cm
Let the focal length of the concave lens with the liquid be f2.
For a pair of optical systems in contact, the equivalent focal length is given by:
\(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}\)
Putting the values, we get,
\(\frac{1}{45}=\frac{1}{30}+\frac{1}{f_2}\)
\(\frac{1}{f_2}=\frac{1}{45}-\frac{1}{30}\)
\(\frac{1}{f_2}=\frac{2-3}{90}=-\frac{1}{90}\)
\(f_2=-90\text{ cm}\)
STEP 2:-
In order to find the value of radius of curvature:
Lens makers formula: \(\frac{1}{f}=(n_{21}−1)(\frac{1}{R_1}−\frac{1}{R_2})\)
Let the refractive index of the lens be n1,
and the radius of curvature of one surface be R1=R.
The radius of curvature of the other surface is R2=-R.
Using the lens makers formula,
\(\frac{1}{f}=(n_{21}−1)(\frac{1}{R_1}−\frac{1}{R_2})\)
\(\frac{1}{30}=(1.5−1)(\frac{1}{R}−\frac{1}{-R})\)
\(\frac{1}{30}=0.5(\frac{2}{R})\)
\(\frac{1}{30}=\frac{1}{R}\)
\(R=30\text{ cm}\)
STEP 3:-
In order to find the refractive index of the liquid:
Lens makers formula: \(\frac{1}{f}=(n_{21}−1)(\frac{1}{R_1}−\frac{1}{R_2})\)
The radius of curvature of the liquid lens, R'1=-30 cm.
The other side of the liquid is a plane, so R'2=∞.
Let n2 be the refractive index of the liquid.
Using the lens maker's formula,
\(\frac{1}{f_2}=(n_2−1)(\frac{1}{R_1}−\frac{1}{R_2})\)
\(−\frac{1}{90}=(n_2−1)(\frac{1}{-30}−\frac{1}{∞})\)
\(−\frac{1}{90}=−\frac{1}{30}(n_2−1)\)
\(n_2−1=\frac{1}{3}\)
\(n_2=1.33\)
Hence, the refractive index of the liquid is 1.33.
Therefore, the final Answer: n=1.33
Top Questions on Ray optics and optical instruments
- Explain the transmission of optical signal through an optical fiber with a diagram.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- With the help of a ray diagram, show that a straw appears bent when it is partly dipped in water and explain it.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- When a parallel beam of light enters water surface obliquely at some angle, what is the effect on the width of the beam?
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- Two convex lenses A and B, each of focal length 10.0 cm, are mounted on an optical bench at 50.0 cm and 70.0 cm respectively. An object is mounted at 20.0 cm. Find the nature and position of the final image formed by the combination.
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
- The focal length of a concave mirror in air is \( f \). When the mirror is immersed in a liquid of refractive index \( \frac{3}{5} \), its focal length will become:
- CBSE CLASS XII - 2025
- Physics
- Ray optics and optical instruments
Questions Asked in CBSE CLASS XII exam
- Find the maximum slope of the curve \( y = x^3 + 3x^2 + 9x - 30 \).
- CBSE CLASS XII - 2025
- CBSE Compartment XII - 2025
- Application of derivatives
- Differentiate $2\cos^2 x$ w.r.t. $\cos^2 x$.
- CBSE CLASS XII - 2025
- Derivatives
- Arushi, Vivaan and Mitali were partners in a firm. On 31st March 2024, the firm was dissolved. On that date, the firm had debtors of \( ₹ 60,000 \) and provision for doubtful debts of \( ₹ 3,000 \) were existing in the books. Debtors of \( ₹ 8,000 \) proved bad and full amount was realised from the remaining debtors. The amount realised from debtors was:
- CBSE CLASS XII - 2025
- Dissolution of Partnership Firms
- A proton moving with velocity \( V \) in a non-uniform magnetic field traces a path as shown in the figure. The path followed by the proton is always in the plane of the paper. What is the direction of the magnetic field in the region near points P, Q, and R? What can you say about relative magnitude of magnetic fields at these points?

- CBSE CLASS XII - 2025
- Motion of charged particles in a magnetic field
- A conductor of length \( l \) is connected across an ideal cell of emf E. Keeping the cell connected, the length of the conductor is increased to \( 2l \) by gradually stretching it. If R and \( R' \) are initial and final values of resistance and \( v_d \) and \( v_d' \) are initial and final values of drift velocity, find the relation between:
\( R' \) and \( R \)
\( R' = 4R \)- CBSE CLASS XII - 2025
- Current electricity
Concepts Used:
Spherical Mirrors
A spherical mirror is a mirror which has been cut out of a spherical surface.
There are two kinds of spherical mirrors:
- Convex Mirror
- Concave Mirror
Concave Mirror
Concave mirrors are also called converging mirrors, because in these types of mirrors, light rays converge at a point after impact and reflect back from the reflective surface of the mirror.
Convex Mirror
The convex mirror has a reflective surface that is curved outward. Regardless of the distance between the subject and the mirrors, these mirrors are "always" virtual, upright and reduced.