Question:
\(∫e^x secx(1+tanx)dx\) equals
\(∫e^x secx(1+tanx)dx\) equals
Updated On: Mar 1, 2024
\(e^xcosx+C\)
\(e^xsecx+C\)
\(e^xsinx+C\)
\(e^xtanx+C\)
Hide Solution
Verified By Collegedunia
The Correct Option is A
Solution and Explanation
The correct answer isB: \(I=e^xsecx+C\)
\(∫e^x secx(1+tanx)dx\)
Let \(I=∫e^xsecx(1+tanx)dx=∫e^x(secx+secx\,tanx)dx\)
Also,let \(secx=ƒ(x)\,\,secx\,tanx=ƒ'(x)\)
It is known that,\(∫e^x[ƒ(x)+ƒ'(x)]dx=e^xƒ(x)+C\)
\(∴I=e^xsecx+C\)
Hence,the correct answer is B.
\(∫e^x secx(1+tanx)dx\)
Let \(I=∫e^xsecx(1+tanx)dx=∫e^x(secx+secx\,tanx)dx\)
Also,let \(secx=ƒ(x)\,\,secx\,tanx=ƒ'(x)\)
It is known that,\(∫e^x[ƒ(x)+ƒ'(x)]dx=e^xƒ(x)+C\)
\(∴I=e^xsecx+C\)
Hence,the correct answer is B.
Was this answer helpful?
1
0
Top Questions on integral
- Evaluate the integral: \[ \int \frac{\sin(2x)}{\sin(x)} \, dx \]
- Find the value of the integral: \[ \int_0^\pi \sin^2(x) \, dx. \]
- Evaluate the integral: $$ \int_0^{\pi/4} \frac{\ln(1 + \tan x)}{\cos x \sin x} \, dx $$
- Evaluate the integral \( \int_0^1 \frac{\ln(1 + x)}{1 + x^2} \, dx \)
- Let $ f(x) $ be a positive function and $I_1 = \int_{-\frac{1}{2}}^1 2x \, f\left(2x(1-2x)\right) dx$ and $I_2 = \int_{-1}^2 f\left(x(1-x)\right) dx.$ Then the value of $\frac{I_2}{I_1}$ is equal to ____
View More Questions
Questions Asked in CBSE CLASS XII exam
- Why is a solution of Ni(H₂O)₆²⁺ green while a solution of Ni(CN)₄²⁻ is colourless? (At. No. of Ni = 28)
- CBSE CLASS XII - 2025
- Organic Chemistry
- Define the term mutual inductance. Deduce the expression for the mutual inductance of two long coaxial solenoids of the same length having different radii and different number of turns.
- CBSE CLASS XII - 2025
- Self and Mutual inductance of simple configurations
परसेवा का आनंद — 120 शब्दों में रचनात्मक लेख लिखिए:
- CBSE CLASS XII - 2025
- प्रश्न उत्तर
- In the post-independence era, the policy makers of India pushed for ‘self-reliance’ for the first _______ Five Year Plans. (Choose the correct option to fill in the blank)
- CBSE CLASS XII - 2025
- Indian Economy
Answer the following questions:
[(i)] Explain the structure of a mature embryo sac of a typical flowering plant.
[(ii)] How is triple fusion achieved in these plants?
OR
[(i)] Describe the changes in the ovary and the uterus as induced by the changes in the level of pituitary and ovarian hormones during menstrual cycle in a human female.
- CBSE CLASS XII - 2025
- sexual reproduction in flowering plants
View More Questions
Concepts Used:
Integration by Partial Fractions
The number of formulas used to decompose the given improper rational functions is given below. By using the given expressions, we can quickly write the integrand as a sum of proper rational functions.
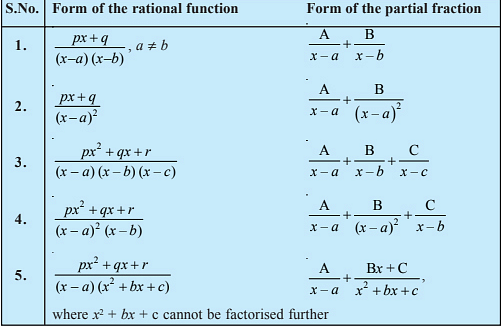
For examples,
