A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force F, its length increases by 5 cm. Another wire of the same material of length 4L and radius 4r is pulled by a force 4F under same conditions. The increase in length of this wire is ___ cm.
Correct Answer: 5
Solution and Explanation
\(\frac{\frac{F}{A}}{\frac{\Delta L}{L}}\)=Y ⇒△L=\(\frac{FL}{AY}\) \(\frac{△L_2}{△L_1}\)=(\(\frac{F_2}{F_1}\))×(\(\frac{L_2}{L_1}\))×(\(\frac{A_1}{A_2}\))
=\(\frac{4×4×1}{16}\)=1 △L2=△L1=5cm
Top Questions on elastic moduli
- The slope of the stress-strain curve in the elastic deformation region is
- JKCET - 2024
- Physics
- elastic moduli
- The law which states that within elastic limits strain produced is proportional to the stress producing it is known as
- JKCET - 2024
- Physics
- elastic moduli
The elastic behavior of material for linear stress and linear strain, is shown in the figure. The energy density for a linear strain of 5×10–4 is ____ kJ/m3. Assume that material is elastic up to the linear strain of 5×10–4
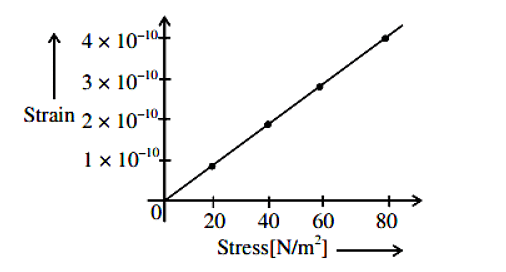
- JEE Main - 2022
- Physics
- elastic moduli
- If the length of a wire is made double and radius is halved of its respective values. Then, the Young’s modulus of the material of the wire will :
- JEE Main - 2022
- Physics
- elastic moduli
- A screw gauge gives the following readings when used to measure the diameter of a wire Main scale reading : $0\, mm$ Circular scale reading : $52$ divisions Given that $1\, mm$ on main scale corresponds to $100$ divisions on the circular scale. The diameter of the wire from the above data is :
- NEET (UG) - 2021
- Physics
- elastic moduli
Questions Asked in JEE Main exam
- Let \( P_n = \alpha^n + \beta^n \), \( P_{10} = 123 \), \( P_9 = 76 \), \( P_8 = 47 \), and \( P_1 = 1 \). The quadratic equation whose roots are \( \frac{1}{\alpha} \) and \( \frac{1}{\beta} \) is:
- JEE Main - 2025
- Sequences and Series
For the thermal decomposition of \( N_2O_5(g) \) at constant volume, the following table can be formed, for the reaction mentioned below: \[ 2 N_2O_5(g) \rightarrow 2 N_2O_4(g) + O_2(g) \] Given: Rate constant for the reaction is \( 4.606 \times 10^{-2} \text{ s}^{-1} \).
- JEE Main - 2025
- Organic Chemistry
O\(_2\) gas will be evolved as a product of electrolysis of:
(A) an aqueous solution of AgNO3 using silver electrodes.
(B) an aqueous solution of AgNO3 using platinum electrodes.
(C) a dilute solution of H2SO4 using platinum electrodes.
(D) a high concentration solution of H2SO4 using platinum electrodes.
Choose the correct answer from the options given below :- JEE Main - 2025
- Electrolysis
Let A be a 3 × 3 matrix such that \(\text{det}(A) = 5\). If \(\text{det}(3 \, \text{adj}(2A)) = 2^{\alpha \cdot 3^{\beta} \cdot 5^{\gamma}}\), then \( (\alpha + \beta + \gamma) \) is equal to:
- JEE Main - 2025
- Matrix Operations
- The number of complex numbers \( z \), satisfying \( |z| = 1 \) and \[ \left| \frac{z}{\overline{z}} + \frac{\overline{z}}{z} \right| = 1, \] is:
- JEE Main - 2025
- complex numbers
Concepts Used:
Elastic Moduli
Elastic moduli, also known as modulus of elasticity, are mechanical properties of materials that describe their ability to resist deformation under an applied load. They are used to quantify the stress-strain relationship of a material.
There are three main types of elastic moduli: Young's modulus (E), shear modulus (G), and bulk modulus (K). Young's modulus is a measure of a material's stiffness in tension or compression, while shear modulus is a measure of its resistance to shear deformation. Bulk modulus is a measure of its resistance to compression.
These moduli are important in many areas of engineering and physics, as they govern the behavior of materials under different types of loading conditions. For example, Young's modulus is used to design and analyze structures such as bridges and buildings, while shear modulus is important in the study of earthquakes and the behavior of soils.
The elastic moduli of a material are dependent on its microstructure, such as its crystal structure, grain size, and defects. They are also influenced by temperature, pressure, and other environmental factors.
The study and measurement of elastic moduli are essential for understanding the mechanical properties of materials, as well as for the design and analysis of structures and devices in engineering and physics.