A thin convex lens is made of two materials with refractive indices $n_1$ and $n_2$, as shown in figure. The radius of curvature of the left and right spherical surfaces are equal. / is the focal length of the lens when $n_1$ = $n_2$ = n. The focal length is $f+\Delta f$ when $n_1$ = n and $n_2 = n + \Delta n$. Assuming $\Delta n << (n ? 1)$ and $1 < n < 2$, the correct statement(s) is/are,
- $\left|\frac{\Delta f}{f}\right|
- For $n = 1.5, \Delta n = 10^{-3}$ and $f = 20$ cm, the value of $\left|\Delta f\right|$ will be 0.02 cm (round off to $2^{nd}$ decimal place)
- If $\frac{\Delta n}{n}<0$ then $\frac{\Delta f}{f}>0$
- The relation between $ \frac{\Delta f}{f}$ and $\frac{\Delta n}{n}$ remains unchanged if both the convex surfaces are replaced by concave surfaces of the same radius of curvature
The Correct Option is D
Solution and Explanation
$\frac{1}{f}=\frac{\left(n_{1}-1\right)}{R}+\frac{n_{2}-1}{R}=\frac{\left(n_{1}+n_{2}-2\right)}{R}$
Now $\frac{\Delta f}{f^{2}}=\frac{\Delta n}{R}$
$\frac{\Delta f}{f}=\frac{\Delta n}{\left(n_{1}+n_{2}-2\right)}=\frac{\Delta n}{\left[2n+\Delta n-2\right]}$
For $n_{1}=n_{2}=1.5\quad\Delta n=10^{-3}, f=20cm then R = 20 cm$
and $\Delta f=\frac{10^{-3}\times20}{\left(2\times1.5-2+10^{-3}\right)}=0.02\,cm.$
If $\frac{\Delta n}{n}<0\left(Diversing nature increases\right) \,\therefore\frac{\Delta f}{f}>0$
If the surfaces are replaced by concave surfaces of same radius, focal length changes the sign withsame magnitude.
$\therefore\frac{\Delta f}{f}=\frac{\Delta n}{\left(2n+\Delta n-2\right)}$ (remain unchanged).
Top Questions on Spherical Mirrors
- A concave mirror produces an image of an object such that the distance between the object and image is 20 cm. If the magnification of the image is \( -3 \), then the magnitude of the radius of curvature of the mirror is:
- JEE Main - 2025
- Physics
- Spherical Mirrors
- (ii) An object at a distance of 16 cm from a spherical mirror forms a virtual image at a distance of 12 cm behind the mirror. Determine the magnification of the image and type of the mirror.
- UP Board X - 2025
- Science
- Spherical Mirrors
- Image of an object formed by a concave mirror is real and of the size of the object. The object is placed -
- UP Board X - 2025
- Science
- Spherical Mirrors
- With the help of a suitable ray diagram, derive the formula \( \frac{1}{v} + \frac{1}{u} = \frac{1}{f} \) for a concave mirror.
- UP Board XII - 2025
- Physics
- Spherical Mirrors
- The length of the image formed by a concave mirror:
- UP Board XII - 2025
- Physics
- Spherical Mirrors
Questions Asked in JEE Advanced exam
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
A temperature difference can generate e.m.f. in some materials. Let $ S $ be the e.m.f. produced per unit temperature difference between the ends of a wire, $ \sigma $ the electrical conductivity and $ \kappa $ the thermal conductivity of the material of the wire. Taking $ M, L, T, I $ and $ K $ as dimensions of mass, length, time, current and temperature, respectively, the dimensional formula of the quantity $ Z = \frac{S^2 \sigma}{\kappa} $ is:
- JEE Advanced - 2025
- Dimensional Analysis
- Let $$ \alpha = \frac{1}{\sin 60^\circ \sin 61^\circ} + \frac{1}{\sin 62^\circ \sin 63^\circ} + \cdots + \frac{1}{\sin 118^\circ \sin 119^\circ}. $$ Then the value of $$ \left( \frac{\csc 1^\circ}{\alpha} \right)^2 $$ is \rule{1cm}{0.15mm}.
- JEE Advanced - 2025
- Some Applications of Trigonometry
Concepts Used:
Spherical Mirrors
A spherical mirror is a mirror which has been cut out of a spherical surface.
There are two kinds of spherical mirrors:
- Convex Mirror
- Concave Mirror
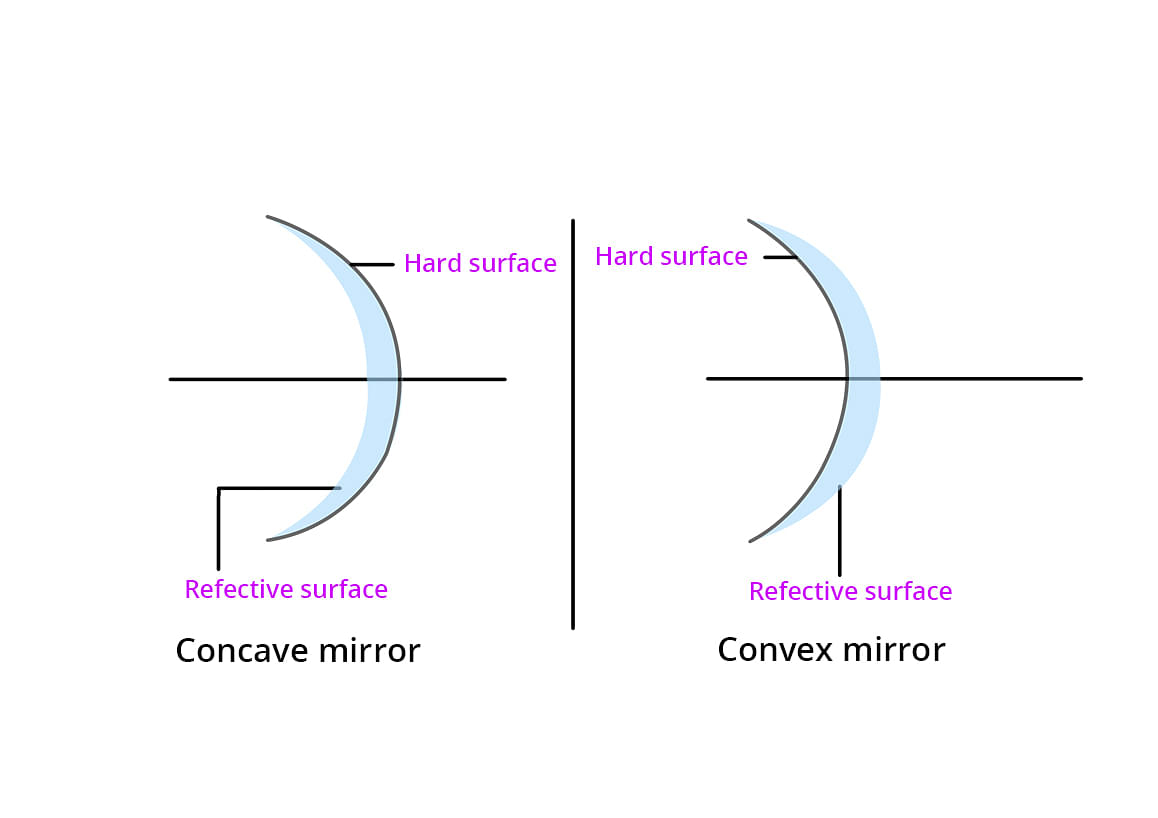
Concave Mirror
Concave mirrors are also called converging mirrors, because in these types of mirrors, light rays converge at a point after impact and reflect back from the reflective surface of the mirror.
Convex Mirror
The convex mirror has a reflective surface that is curved outward. Regardless of the distance between the subject and the mirrors, these mirrors are "always" virtual, upright and reduced.