Question:
A solid copper cube of $7 \,cm$ edge is subjected to a hydraulic pressure of $8000\, kPa$. The volume contraction of the copper cube is (Bulk modulus of copper = $140 \,GPa$ )
A solid copper cube of $7 \,cm$ edge is subjected to a hydraulic pressure of $8000\, kPa$. The volume contraction of the copper cube is (Bulk modulus of copper = $140 \,GPa$ )
Updated On: Apr 4, 2024
- $ 196 \times 10^{-3} cm^3$
- $ 19.6 \times 10^{-6} cm^3$
- $ 19.6 \times 10^{-3} cm^3$
- $ 196 \times 10^{3} cm^3$
Hide Solution
Verified By Collegedunia
The Correct Option is C
Solution and Explanation
Given,
edge of solid copper cube, $l=7 \,cm$
hydraulic pressure, $p=8000 \,kPa =8000 \times 10^{3}\, Pa$
and Bulk modulus of copper, $\beta=140\, GPa$
$=140 \times 10^{9} \,Pa$
As we know that,
Bulk modulus, $\beta=\frac{p}{\left(\frac{\Delta V}{V}\right)}$
or $\beta=\frac{p V}{\Delta V}$
$\therefore \Delta V=\frac{p V}{\beta} =\frac{8000 \times 10^{3} \times(l)^{3}}{140 \times 10^{9}} $
$=\frac{8000 \times 10^{3} \times(7)^{3}}{140 \times 10^{9}}$
$=19.6 \times 10^{-3} \,cm ^{3} $
edge of solid copper cube, $l=7 \,cm$
hydraulic pressure, $p=8000 \,kPa =8000 \times 10^{3}\, Pa$
and Bulk modulus of copper, $\beta=140\, GPa$
$=140 \times 10^{9} \,Pa$
As we know that,
Bulk modulus, $\beta=\frac{p}{\left(\frac{\Delta V}{V}\right)}$
or $\beta=\frac{p V}{\Delta V}$
$\therefore \Delta V=\frac{p V}{\beta} =\frac{8000 \times 10^{3} \times(l)^{3}}{140 \times 10^{9}} $
$=\frac{8000 \times 10^{3} \times(7)^{3}}{140 \times 10^{9}}$
$=19.6 \times 10^{-3} \,cm ^{3} $
Was this answer helpful?
18
0
Top Questions on elastic moduli
- The slope of the stress-strain curve in the elastic deformation region is
- JKCET - 2024
- Physics
- elastic moduli
- The law which states that within elastic limits strain produced is proportional to the stress producing it is known as
- JKCET - 2024
- Physics
- elastic moduli
- A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force F, its length increases by 5 cm. Another wire of the same material of length 4L and radius 4r is pulled by a force 4F under same conditions. The increase in length of this wire is ___ cm.
- JEE Main - 2022
- Physics
- elastic moduli
The elastic behavior of material for linear stress and linear strain, is shown in the figure. The energy density for a linear strain of 5×10–4 is ____ kJ/m3. Assume that material is elastic up to the linear strain of 5×10–4
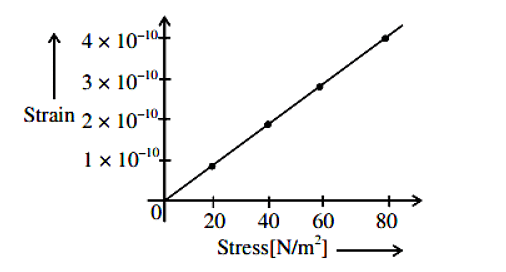
- JEE Main - 2022
- Physics
- elastic moduli
- If the length of a wire is made double and radius is halved of its respective values. Then, the Young’s modulus of the material of the wire will :
- JEE Main - 2022
- Physics
- elastic moduli
View More Questions
Questions Asked in AP EAPCET exam
- The domain and range of a real valued function \( f(x) = \cos (x-3) \) are respectively.
- AP EAPCET - 2025
- Functions
- If the line $$ 4x - 3y + 7 = 0 $$ touches the circle $$ x^2 + y^2 - 6x + 4y - 12 = 0 $$ at $ (\alpha, \beta) $, then find $ \alpha + 2\beta $.
- The number of ways of selecting 3 numbers that are in Arithmetic Progression (A.P.) from the set \(\{1, 2, 3, \ldots, 100\}\) is:
- AP EAPCET - 2025
- Arithmetic Progressions
- The numerically greatest term in the expansion of $ (x + 3y)^{13} $, when $ x = \frac{1}{2},\ y = \frac{1}{3} $, is
- AP EAPCET - 2025
- Binomial theorem
- The general solution of the differential equation \(\frac{dy}{dx} + xy = 4x - 2y + 8\) is
- AP EAPCET - 2025
- Differential Equations
View More Questions