Question:
$28.0\, L$ of $CO _2$ is produced on complete combustion of $168 \, L$ gaseous mixture of ethene and methane at $25^{\circ} C$ and $1 \, atm$ Heat evolved during the combustion process is ______$kJ$ Given : $\Delta H _{ c }\left( CH _4\right)=-900 \, kJ\, mol ^{-1}$ $\Delta H _{ c }\left( C _2 H _4\right)=-1400\, kJ \, mol ^{-1}$
$28.0\, L$ of $CO _2$ is produced on complete combustion of $168 \, L$ gaseous mixture of ethene and methane at $25^{\circ} C$ and $1 \, atm$ Heat evolved during the combustion process is ______$kJ$ Given : $\Delta H _{ c }\left( CH _4\right)=-900 \, kJ\, mol ^{-1}$ $\Delta H _{ c }\left( C _2 H _4\right)=-1400\, kJ \, mol ^{-1}$
Updated On: Mar 19, 2025
Hide Solution
Verified By Collegedunia
Correct Answer: 847 - 848
Approach Solution - 1
The correct answer is 925.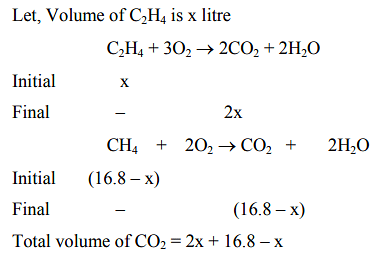
Was this answer helpful?
0
0
Hide Solution
Verified By Collegedunia
Approach Solution -2
The volume of \(\mathrm{CO_2}\) produced enables us to calculate the moles of \(\mathrm{CH_4}\) and \(\mathrm{C_2H_4}\) combusted, from which we can determine the total heat evolved using their respective combustion enthalpies.
Let, the volume of \[ C_2H_4 \text{ be } x \text{ litres.} \]
\[ C_2H_4 + 3O_2 \rightarrow 2CO_2 + 2H_2O \]
Initial volume: \(x\)
Final volume: \(2x\)
For \(\mathrm{CH_4}\), the reaction is \[ CH_4 + 2O_2 \rightarrow CO_2 + 2H_2O \]
Initial volume: \(16.8 - x\)
Final volume: \(16.8 - x\)
The total volume of \(\mathrm{CO_2}\) produced is: \[ \text{Total } CO_2 = 2x + (16.8 - x) \] Thus, \[ 28 = 16.8 + x \] Solving for \(x\), we get \[ x = 11.2 \text{ L} \] Now, calculate the moles of the gases: \[ n_{CH_4} = \frac{PV}{RT} = \frac{1 \times 5.6}{0.082 \times 298} = 0.229 \text{ moles} \] \[ n_{C_2H_4} = \frac{11.2}{0.082 \times 298} = 0.458 \text{ moles} \] Therefore, the heat evolved is: \[ \text{Heat evolved} = 0.229 \times 900 + 0.458 \times 1400 \] \[ = 206.1 + 641.2 = 847.3 \text{ kJ} \]
Let, the volume of \[ C_2H_4 \text{ be } x \text{ litres.} \]
\[ C_2H_4 + 3O_2 \rightarrow 2CO_2 + 2H_2O \]
Initial volume: \(x\)
Final volume: \(2x\)
For \(\mathrm{CH_4}\), the reaction is \[ CH_4 + 2O_2 \rightarrow CO_2 + 2H_2O \]
Initial volume: \(16.8 - x\)
Final volume: \(16.8 - x\)
The total volume of \(\mathrm{CO_2}\) produced is: \[ \text{Total } CO_2 = 2x + (16.8 - x) \] Thus, \[ 28 = 16.8 + x \] Solving for \(x\), we get \[ x = 11.2 \text{ L} \] Now, calculate the moles of the gases: \[ n_{CH_4} = \frac{PV}{RT} = \frac{1 \times 5.6}{0.082 \times 298} = 0.229 \text{ moles} \] \[ n_{C_2H_4} = \frac{11.2}{0.082 \times 298} = 0.458 \text{ moles} \] Therefore, the heat evolved is: \[ \text{Heat evolved} = 0.229 \times 900 + 0.458 \times 1400 \] \[ = 206.1 + 641.2 = 847.3 \text{ kJ} \]
Was this answer helpful?
0
0
Top Questions on Thermodynamics
- Match List-I with List-II
\[ \begin{array}{|l|l|} \hline \textbf{List-I} & \textbf{List-II} \\ \hline (A) \; \text{Classius Clapeyron equation} & (I) \; PV^\gamma = \text{constant} \\ (B) \; \text{Gibbs Function} & (II) \; U + PV \\ (C) \; \text{Enthalpy} & (III) \; U - TS + PV \\ (D) \; \text{Adiabatic change in Perfect Gas} & (IV) \; \dfrac{dP}{dT} = \dfrac{L}{T (V_2 - V_1)} \\ \hline \end{array} \]
Choose the correct answer from the options given below:
- CUET (PG) - 2025
- Geophysics
- Thermodynamics
- The energy radiated per minute from the filament of an incandescent lamp at 2000 K, surface area \( 4 \times 10^{-5} \) m² and relative emittance is 0.85, will be (Given Stefan's constant \( \sigma = 5.7 \times 10^{-8} \) Jm⁻²s⁻¹K⁻⁴)
- CUET (PG) - 2025
- Geophysics
- Thermodynamics
- The ratio of adiabatic to the isobaric coefficient of expansion is
- CUET (PG) - 2025
- Geophysics
- Thermodynamics
- Which of following is correct form of first TdS equation ?
- CUET (PG) - 2025
- Geophysics
- Thermodynamics
- Work done in compressing adiabatically 1g of air, initially at NTP to one fourth of its original volume is: (take density of air = 0.0001465 g/cm³ and \(\gamma\) = 1.5)
- CUET (PG) - 2025
- Geophysics
- Thermodynamics
View More Questions
Questions Asked in JEE Main exam
A square loop of sides \( a = 1 \, {m} \) is held normally in front of a point charge \( q = 1 \, {C} \). The flux of the electric field through the shaded region is \( \frac{5}{p} \times \frac{1}{\varepsilon_0} \, {Nm}^2/{C} \), where the value of \( p \) is:

- JEE Main - 2025
- Gauss's Law
- Let \( \alpha_1 \) and \( \beta_1 \) be the distinct roots of \( 2x^2 + (\cos\theta)x - 1 = 0, \ \theta \in (0, 2\pi) \). If \( m \) and \( M \) are the minimum and the maximum values of \( \alpha_1 + \beta_1 \), then \( 16(M + m) \) equals:
- JEE Main - 2025
- Binomial Expansion
- Let the foci of a hyperbola be \( (1, 14) \) and \( (1, -12) \). If it passes through the point \( (1, 6) \), then the length of its latus-rectum is:
- JEE Main - 2025
- Hyperbola
- Which of the following is the correct IUPAC name of the given organic compound (X)? The structure of compound $ X $ is as follows: $ \text{H}_3\text{C} - \text{CH}_3 - \text{CH} = \text{CH} - \text{H} - \text{Br} $
- JEE Main - 2025
- Nomenclature Of Organic Compounds
- Suppose A and B are the coefficients of the 30th and 12th terms respectively in the binomial expansion of \( (1 + x)^{2n - 1} \). If \( 2A = 5B \), then \( n \) is equal to:
- JEE Main - 2025
- Coordinate Geometry
View More Questions